For a moving body at any instant of time
If the body is not moving, the acceleration is necessarily zero.
If the body is slowing, the retardation is negative
If the body is slowing, the distance is negative.
If displacement, velocity and acceleration at that instant are known, we can find the displacement at any given time in future.
The correct Answer is:D
To solve the question regarding the statements about a moving body at any instant of time, we will analyze each statement step by step.
1. Statement Analysis:
- We have four statements to evaluate regarding a moving body.
2. Statement 1: "If the body is not moving, the acceleration is necessarily zero."
- Evaluation: This statement is false. A body can have zero velocity (not moving) but still have a non-zero acceleration. For example, when a ball is thrown upwards, at its maximum height, its velocity is zero, but it experiences gravitational acceleration downwards.
3. Statement 2: "If the body is slowing, the acceleration is negative."
- Evaluation: This statement is false. If a body is slowing down, it means that the acceleration is in the opposite direction to the velocity. If we consider the motion in the positive direction, the acceleration would be negative. However, it is important to note that the term "slowing" typically refers to a decrease in speed, which indicates that the acceleration is indeed negative.
4. Statement 3: "If the body is slowing, the distance is negative."
- Evaluation: This statement is false. Distance is a scalar quantity and cannot be negative. It only measures how much ground an object has covered regardless of its direction.
5. Statement 4: "If displacement, velocity, and acceleration at that instant are known, we can find the displacement at any given time in the future."
- Evaluation: This statement is true. Using kinematic equations, if we know the initial displacement (x0), initial velocity (u), and acceleration (a) at a given time, we can calculate the displacement (x) at any future time (t) using the equation:
x=x0+ut+12at2
6. Conclusion:
- The only correct statement among the four is the last one. Therefore, the answer to the question is that the fourth statement is true.
Summary of Findings:
- Statement 1: False
- Statement 2: False
- Statement 3: False
- Statement 4: True
- We have four statements to evaluate regarding a moving body.
- Evaluation: This statement is false. A body can have zero velocity (not moving) but still have a non-zero acceleration. For example, when a ball is thrown upwards, at its maximum height, its velocity is zero, but it experiences gravitational acceleration downwards.
- Evaluation: This statement is false. If a body is slowing down, it means that the acceleration is in the opposite direction to the velocity. If we consider the motion in the positive direction, the acceleration would be negative. However, it is important to note that the term "slowing" typically refers to a decrease in speed, which indicates that the acceleration is indeed negative.
- Evaluation: This statement is false. Distance is a scalar quantity and cannot be negative. It only measures how much ground an object has covered regardless of its direction.
- Evaluation: This statement is true. Using kinematic equations, if we know the initial displacement (x0), initial velocity (u), and acceleration (a) at a given time, we can calculate the displacement (x) at any future time (t) using the equation:
x=x0+ut+12at2
- The only correct statement among the four is the last one. Therefore, the answer to the question is that the fourth statement is true.
- Statement 1: False
- Statement 2: False
- Statement 3: False
- Statement 4: True
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - EXERCISE -II
80 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - PRACTICE EXERCISE
58 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-III
49 Videos
Similar Questions
Knowledge Check
A particle is moving on a circular path of 10 m radius. At any instant of time, its speed is 5ms−1 and the speed is increasing at a rate of 2ms−2. At this instant, the magnitude of the net acceleration will be
A5ms−2B2ms−2C3.2ms−2D4.3ms−2
The coordinates of a particle moving in XY-plane at any instant of time t are x=4t2,y=3t2. The speed of the particle at that instant is
View SolutionFrom a metallic charged body a current is drawn. The rate of increase of current at an instant is equal to the charge on the body at that instant. If the initial charge on the body is Q
View SolutionFigure shows the direction of the total acceleration and velocity of a particle moving clockwise in a circle radius m at an instant of time. Tangential acceleration this instant is 5m/s2. Which of the following statements is not correct?

View SolutionAssertion : A body is momentarily at rest at the instant it reverses the direction.
Reason : A body cannot have acceleration if its velocity is zero at a given instant of time.
View Solution(A) : A body can have acceleration even if its velocity is zero at a given instant of time.
(r) : A body is momentarily at rest when it reverses its direction of motion.
View SolutionFigur shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5m at a given instant of time. At this instant, find:
(a) the radius acceleration,
(b) the speed of the acceleration,
(c) its tangential acceleration.
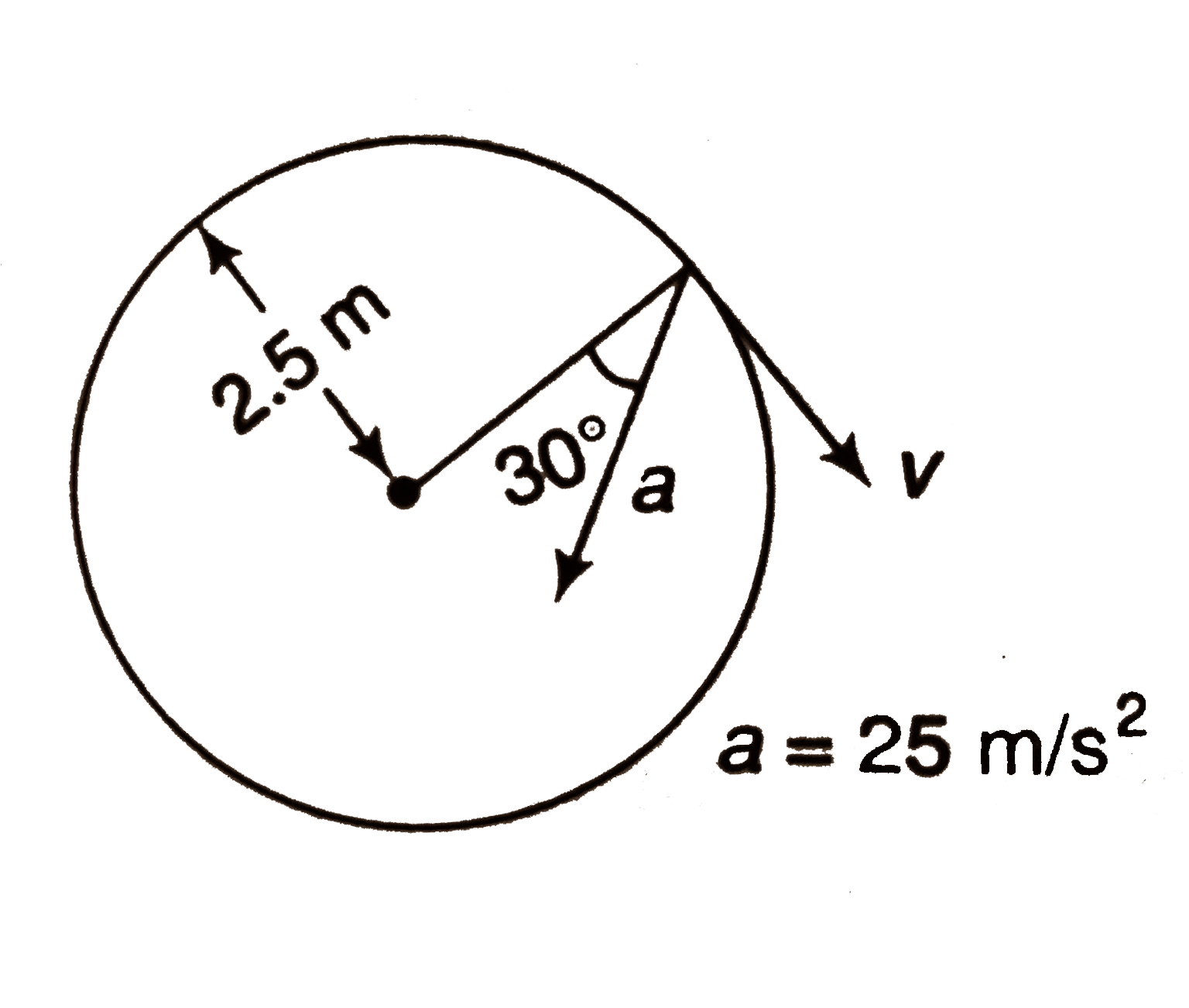
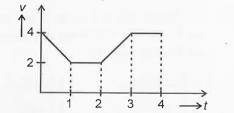
View SolutionThe velocity-time graph for a moving body is shown in figure below. If body moves in straight line along x-axis without changing its direction, then total displacement of body would be
View Solution
