A balloon rases up with uniform velocity 'u'. A body is dropped from ballon. The time of descent for the body is given by is
√2hg
h=ut+12gt2
h=−ut+12gt2
−h=ut+12gt2
The correct Answer is:C
To solve the problem of a body being dropped from a balloon that rises with uniform velocity 'u', we need to analyze the motion of the body after it is released. Here’s a step-by-step solution:
Step 1: Understand the scenario
The balloon is moving upwards with a uniform velocity 'u'. When the body is dropped from the balloon, it initially has the same upward velocity 'u' as the balloon.
Step 2: Define the coordinate system
We will take the upward direction as positive. Therefore, the downward direction will be negative.
Step 3: Identify the variables
- Initial velocity of the body (u) = +u (upward)
- Acceleration (a) = -g (downward)
- Displacement (s) = -h (the body moves downward to reach the ground)
Step 4: Use the kinematic equation
We will use the kinematic equation for uniformly accelerated motion:
s=ut+12at2
Step 5: Substitute the known values
Substituting the values we have:
- s=−h
- u=+u
- a=−g
The equation becomes:
−h=ut+12(−g)t2
Step 6: Simplify the equation
This simplifies to:
−h=ut−12gt2
Rearranging gives:
h=−ut+12gt2
Step 7: Final form of the equation
Thus, we can express the equation as:
h=−ut+12gt2
This indicates that the time of descent for the body can be determined from this equation, confirming that the correct option is:
h=−ut+12gt2
Conclusion
The time of descent for the body is given by the equation:
h=−ut+12gt2
The balloon is moving upwards with a uniform velocity 'u'. When the body is dropped from the balloon, it initially has the same upward velocity 'u' as the balloon.
We will take the upward direction as positive. Therefore, the downward direction will be negative.
- Initial velocity of the body (u) = +u (upward)
- Acceleration (a) = -g (downward)
- Displacement (s) = -h (the body moves downward to reach the ground)
We will use the kinematic equation for uniformly accelerated motion:
s=ut+12at2
Substituting the values we have:
- s=−h
- u=+u
- a=−g
−h=ut+12(−g)t2
This simplifies to:
−h=ut−12gt2
h=−ut+12gt2
Thus, we can express the equation as:
h=−ut+12gt2
h=−ut+12gt2
The time of descent for the body is given by the equation:
h=−ut+12gt2
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - EXERCISE -II
80 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - PRACTICE EXERCISE
58 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-III
49 Videos
Similar Questions
A body moving with uniform velocity
View SolutionA body is dropped from a height H. The time taken to cover second half of the journey is
View SolutionHeight of the body from the ground can be calculated by using the formula h=−ut+(1/2)(gt2) in
(a) A body projected vertically with velocity 'u' from the top of tower, reaches the ground in 't' sec.
( b) A body dropped from a balloon moving up with uniform velocity, reaches the ground in 't' sec
(c) A body dropped from a helicopter moving up with uniform velocity, reaches the ground in 't' sec
(d) A body projected vertically from the ground reaches the ground in 't' sec.
View SolutionA packet is dropped from a balloon rising up with uniform velocity 9.8ms−1. If the balloon is at a height of 39.2 m from the ground at the time of dropping the stone, the stone reaches the ground after
View SolutionThe velocity-time graph of a body is shown in .
The displacement of the body in 8s is.
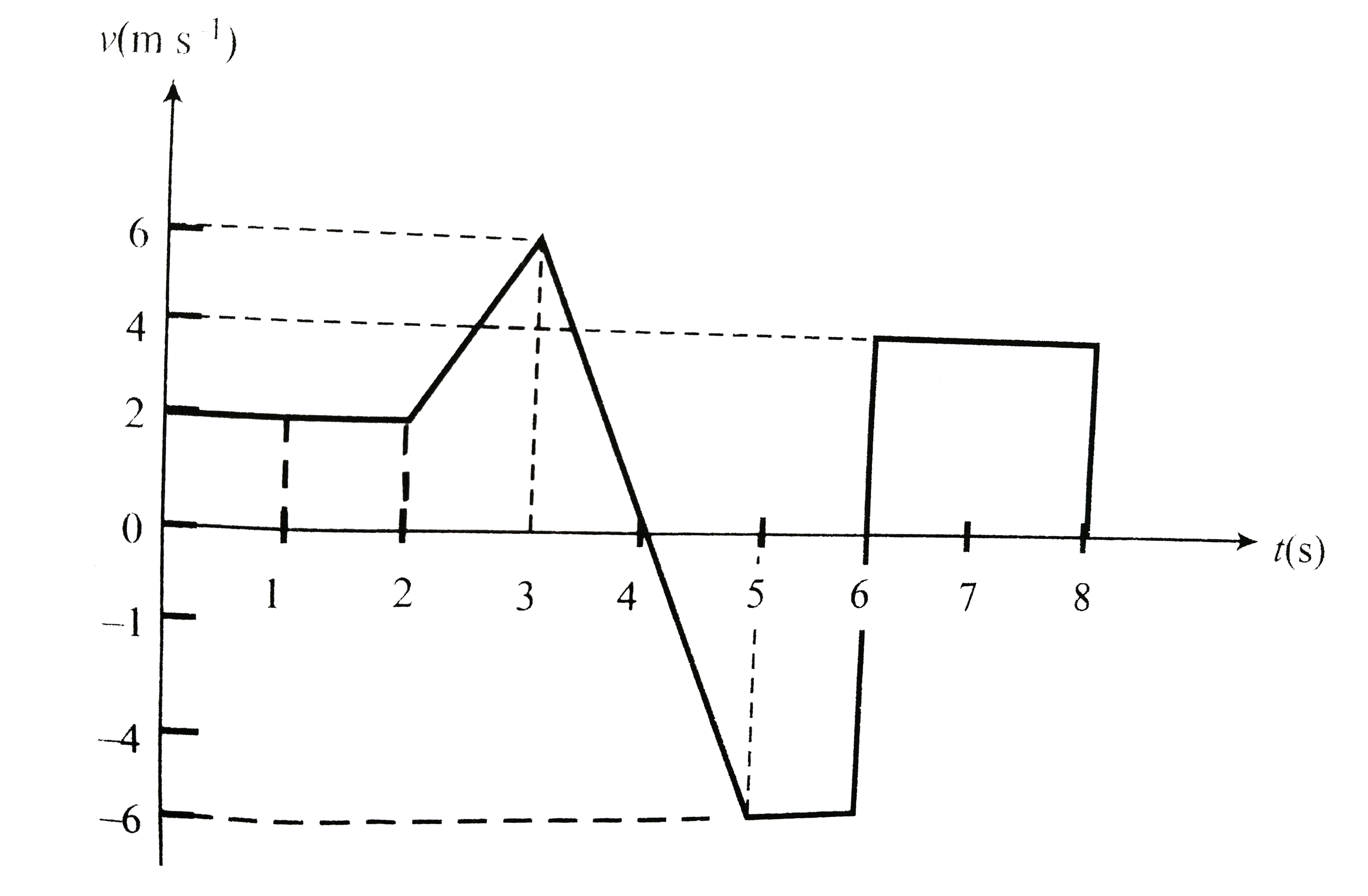 .
.
View SolutionA balloon rises with uniform velocity of 10ms−1. When the balloon is at a height of 75 m if a stone is dropped from it the time taken by it to reach the ground is
View SolutionA body of mass m is moving with a uniform velocity U. A force is applied on the body due to which its velocity increases from u to v: How much work is being done by the force ?
View SolutionA body falls from 80 m. Its time of descent is [g=10ms−2]
View SolutionA stone is dropped from a balloon going up with a uniform velocity of 5.0 m/s. If the balloon was 50 m high when the stone was dropped, find its height when the stone hits the ground. Take g=10ms2.
View SolutionAn aeroplane is moving with a velocity u. It drops a packet from a height h. The time t taken by the packet in reaching the ground will be
View Solution
