Question

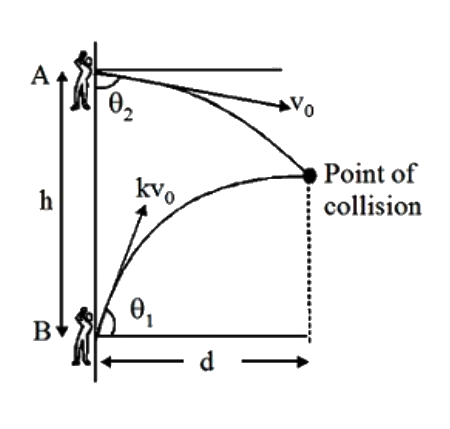
At the same instant two boy throw balls A and B from the window with a speed $v_0$ and $k{v}_0$ respectively , where k is a constant . Show that the balls with collide if $K={\cos \theta }_2/{\cos \theta }_1$.

Explanation

To solve the problem, we need to analyze the motion of the two balls thrown by the boys. Let's denote the following:

- Ball A is thrown with an initial speed $$v_0$$ at an angle $${\theta }_1$$ with the horizontal.
- Ball B is thrown with an initial speed $$k{v}_0$$ at an angle $${\theta }_2$$ with the horizontal.

Step 1: Determine the horizontal displacement of both balls.

The horizontal displacement \( d \) of an object in projectile motion is given by the formula:

\( d = v \cos(\theta) \cdot t \)

For Ball A, the horizontal displacement \( d_A \) is:

\( d_A = v_0 \cos(\theta_1) \cdot t \)

For Ball B, the horizontal displacement \( d_B \) is:

\( d_B = kv_0 \cos(\theta_2) \cdot t \)

Step 2: Set the horizontal displacements equal for collision.

For the two balls to collide, their horizontal displacements must be equal:

\( d_A = d_B \)

Substituting the expressions for \( d_A \) and \( d_B \):

\( v_0 \cos(\theta_1) \cdot t = kv_0 \cos(\theta_2) \cdot t \)

Step 3: Simplify the equation.

Since \( v_0 \) and \( t \) are common terms on both sides, we can cancel them out (assuming \( v_0 \neq 0 \) and \( t \neq 0 \)):

\( \cos(\theta_1) = k \cos(\theta_2) \)

Step 4: Rearrange to find the relationship between \( k \) and the cosines.

Rearranging the equation gives:

\( k = \frac{\cos(\theta_1)}{\cos(\theta_2)} \)

Conclusion

Thus, we have shown that the balls will collide if:

\( k = \frac{\cos(\theta_2)}{\cos(\theta_1)} \)

This completes the proof.

---