SI unit of coefficient of thermal conductivity
The correct Answer is:A
To find the SI unit of the coefficient of thermal conductivity, we can follow these steps:
Step 1: Understand the Definition
The coefficient of thermal conductivity (k) is defined as the amount of heat (Q) that passes through a unit area (A) of a material in a unit time (t) when there is a temperature difference (ΔT) of 1 Kelvin across the material.
Step 2: Write the Formula
The formula for the coefficient of thermal conductivity can be expressed as:
k=QA⋅t⋅ΔT
Where:
- Q = Quantity of heat (in Joules)
- A = Area (in square meters)
- t = Time (in seconds)
- ΔT = Temperature difference (in Kelvin)
Step 3: Identify Units
Now, let's identify the units for each component in the formula:
- The unit of heat (Q) is Joules (J).
- The unit of area (A) is square meters (m²).
- The unit of time (t) is seconds (s).
- The unit of temperature difference (ΔT) is Kelvin (K).
Step 4: Substitute the Units into the Formula
Substituting the units into the formula gives us:
k=Jm2⋅s⋅K
Step 5: Convert Joules to Watts
Since 1 Watt (W) is defined as 1 Joule per second (1 W = 1 J/s), we can rewrite the unit of heat as:
J=W⋅s
Substituting this into our expression for k gives:
k=W⋅sm2⋅s⋅K
Step 6: Simplify the Expression
The seconds (s) in the numerator and denominator cancel out:
k=Wm2⋅K
Conclusion
Thus, the SI unit of the coefficient of thermal conductivity is:
Watt per meter square per Kelvin(W/m2K)
Final Answer
The correct option is Watt per meter square per Kelvin (W/m²K).
---
The coefficient of thermal conductivity (k) is defined as the amount of heat (Q) that passes through a unit area (A) of a material in a unit time (t) when there is a temperature difference (ΔT) of 1 Kelvin across the material.
The formula for the coefficient of thermal conductivity can be expressed as:
k=QA⋅t⋅ΔT
Where:
- Q = Quantity of heat (in Joules)
- A = Area (in square meters)
- t = Time (in seconds)
- ΔT = Temperature difference (in Kelvin)
Now, let's identify the units for each component in the formula:
- The unit of heat (Q) is Joules (J).
- The unit of area (A) is square meters (m²).
- The unit of time (t) is seconds (s).
- The unit of temperature difference (ΔT) is Kelvin (K).
Substituting the units into the formula gives us:
k=Jm2⋅s⋅K
Since 1 Watt (W) is defined as 1 Joule per second (1 W = 1 J/s), we can rewrite the unit of heat as:
J=W⋅s
Substituting this into our expression for k gives:
k=W⋅sm2⋅s⋅K
The seconds (s) in the numerator and denominator cancel out:
k=Wm2⋅K
Thus, the SI unit of the coefficient of thermal conductivity is:
Watt per meter square per Kelvin(W/m2K)
The correct option is Watt per meter square per Kelvin (W/m²K).
Topper's Solved these Questions
- UNITS AND MEASUREMENTBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTEXERCISE - EXERCISE - II
61 Videos
- UNITS AND MEASUREMENTBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTEXERCISE - PRACTICE EXERCISE
45 Videos
- UNITS AND MEASUREMENTBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTEXERCISE - PRACTICE EXERCISE
45 Videos
- SEMICONDUCTOR DEVICESBOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - EXERCISE - III
3 Videos
- UNITS AND MEASUREMENTSBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE -3
66 Videos
Similar Questions
Coefficient of thermal conductivity is the product of heat, distance and reciprocal of (area x difference in temperature x time). The new value of a unit of coefficient of thermal conductivity, if fundamental units are 21.6 kg, 1 decimetre, 4 K and 1 minute will be ______ ×10−6 new units.
View SolutionThe dimensions of coefficient of thermal conductivity is
View SolutionObtain the dimensions of (1) coefficient of thermal conductivity (2) Gas constant (3) Boltzmann constant (4) Planck's constant.
View SolutionDescribe an experiment to determine the coefficient of thermal conductivity of a good conductor by Searle's method.
View SolutionA wall consists of alternating blocks withlength 'd' and coefficient of thermal conductivity k1 and k2. The cross sectional area of the blocks are the same. The equivalent coefficient of thermal conductivity of the wall between left and right is:-

View SolutionA long rod has one end at 0∘C and other end at a high temperature. The coefficient of thermal conductivity varies with distance from the low temperature end as K=K0(1+ax), where K0=102 SI unit and a=1m−1 . At what distance from the first end the temperature will be 100∘C? The area of cross-section is 1cm2 and rate of heat conduction is 1 W.
View SolutionDefine coefficient of thermal conductivity or thermal conductivity of a substance.
View SolutionTwo bar of identical dimensions are kept as shown in figure. If K1 and K2are their coefficients of thermal conductivities, then equivalent K
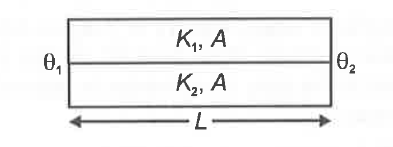
View SolutionTwo rods of length d1 and d2 and coefficients of thermal conductivites K1 and K2 are kept touching each other. Both have the same area of cross-section. The equivalent thermal conductivity.
View SolutionSI unit of work
View Solution
