A man is travelling at 10.8 kmph in a topless car on a rainy day. He holds an umbrella at an angle of 37∘ with the vertical so that he does not wet. If rain drops falls vertically downwards, what is the rain velocity ?
1ms−1
2ms−1
3ms−1
4ms−1
The correct Answer is:D
To solve the problem, we need to determine the velocity of the rain (Vr) given that the man is traveling at a speed (Vm) of 10.8 km/h and holds his umbrella at an angle of 37° with the vertical.
1. Convert the speed of the man from km/h to m/s:
Vm=10.8 km/h=10.8×1000 m3600 s=3 m/s
2. Identify the relationship between the velocities:
The umbrella is held at an angle of 37° with the vertical. The vertical component of the rain's velocity (Vr) and the horizontal component (Vm) can be related using trigonometric functions. Since the rain falls vertically, we can use the tangent of the angle:
tan(37°)=VmVr
3. Substituting the known values:
We know that tan(37°)=34. Therefore, we can write:
34=3 m/sVr
4. Rearranging the equation to find Vr:
Cross-multiplying gives:
3⋅4=3⋅Vr
12=3⋅Vr
Vr=123=4 m/s
5. Conclusion:
The velocity of the rain (Vr) is 4 m/s.
Final Answer:
The rain velocity is 4 m/s.
---
Vm=10.8 km/h=10.8×1000 m3600 s=3 m/s
The umbrella is held at an angle of 37° with the vertical. The vertical component of the rain's velocity (Vr) and the horizontal component (Vm) can be related using trigonometric functions. Since the rain falls vertically, we can use the tangent of the angle:
tan(37°)=VmVr
We know that tan(37°)=34. Therefore, we can write:
34=3 m/sVr
Cross-multiplying gives:
3⋅4=3⋅Vr
12=3⋅Vr
Vr=123=4 m/s
The velocity of the rain (Vr) is 4 m/s.
The rain velocity is 4 m/s.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-2 (A boat in a river)
7 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-2 (Oblique project
30 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-2 (Lami.s theorem :)
8 Videos
- MAGNETISMBOOK - AAKASH SERIESCHAPTER - MAGNETISMEXERCISE - ADDITIONAL EXERCISE
22 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
Similar Questions
A man is travelling at 10.8 kmph in a topless car on a rainy day. He holds an umbrella at an angle of 37∘ with the vertical to protect himself from rain which is falling vertically downwards. What is the velocity of the rain drop ?
View SolutionA glass wind screen whose inclination with the vertical can be changed is mounted on a car. The moves horizontally with a speed of 2m/s. At what angle α with the vertical should the wind screen placed so that the rain drops falling vertically downwards with velcoity 6m/s strike the wind screen perpendicularly?
View SolutionWhen a man moves down the inclined plane with a constant speed 5ms−1 which makes an angle of 37∘ with the horizontal, he finds that the rain is falling vertically downward. When he moves up the same inclined plane with the same speed, he finds that the rain makes an angle θ=tan−1(78) with the horizontal. The speed of the rain is
View SolutionThere are two incline planes AO and OB inclined at 45∘ and 60∘ respectively with the horizontal as shown in the figure. When a man moves on the inclined plane AO he is oberves that the rain drops are falling 45∘ with the vertical. When the man moves on the inclined plane OB, he observes that the raindrops are falling vertically downward with the horizontal. Then actual speed of rain is
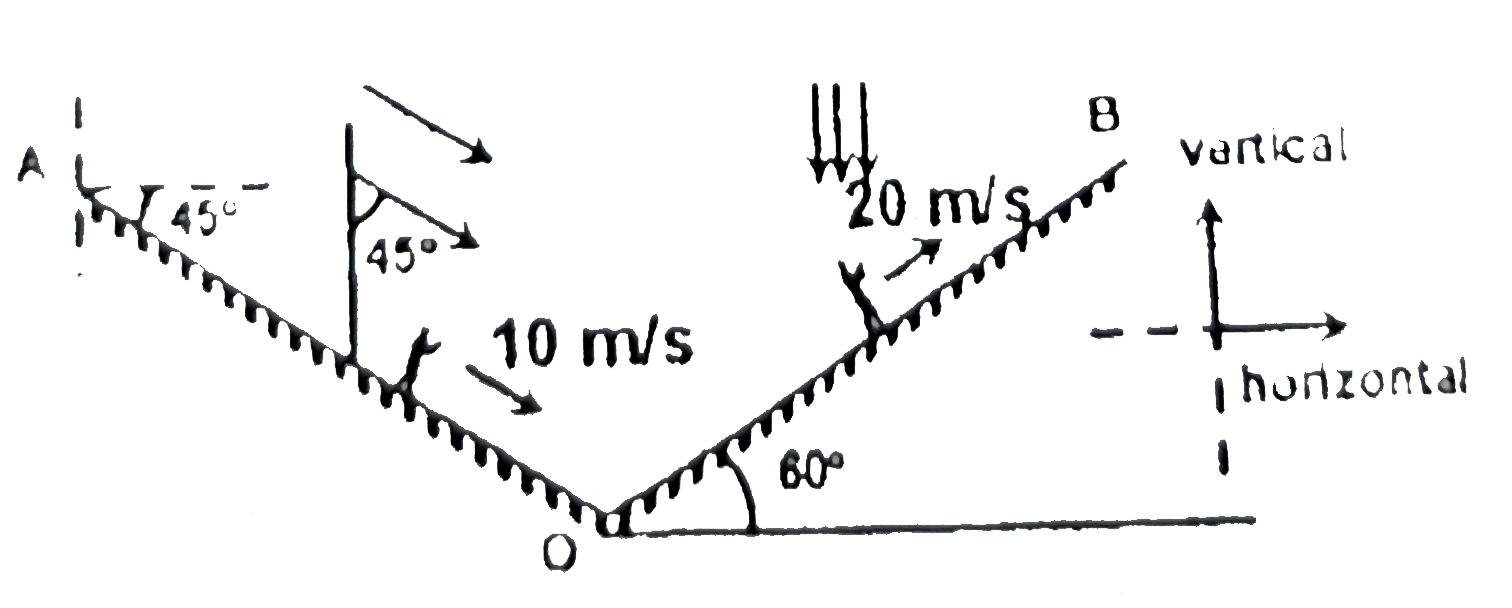
View SolutionA man runs along a horizontal road on a rainy day. His umbrella vertical in order to protect himself from the rain then rain actually
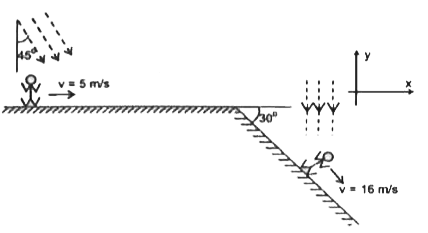
View SolutionA man moving with a velocity of 5m/s on a horizontal road observes that raindrops fall at an angle of 45∘ with the vertical. When he moves with a velocity of 16m/s along an inclined plane, which is inclined at 30∘ with the horizontal , he observes raindrops falling vertically downward as shown in the figure. Find the actual velocity of the raindrops.
View SolutionA person is walking in rain feels the velocity of rain as twice to his velocity. At which angle he should hold the umbrella with vertical if he moves forward, if it is raining vertically downwards
View SolutionA man when standstill observes the rain falling vertically and when he walks at 4km/h he has to hold his umberella at an angle 53∘ from the vertical. Find velocity of the raindrops.
View SolutionA man when standstill observes the rain falling vertically and when he walks at 4km/h he has to hold his umberella at an angle 53∘ from the vertical. Find velocity of the raindrops.
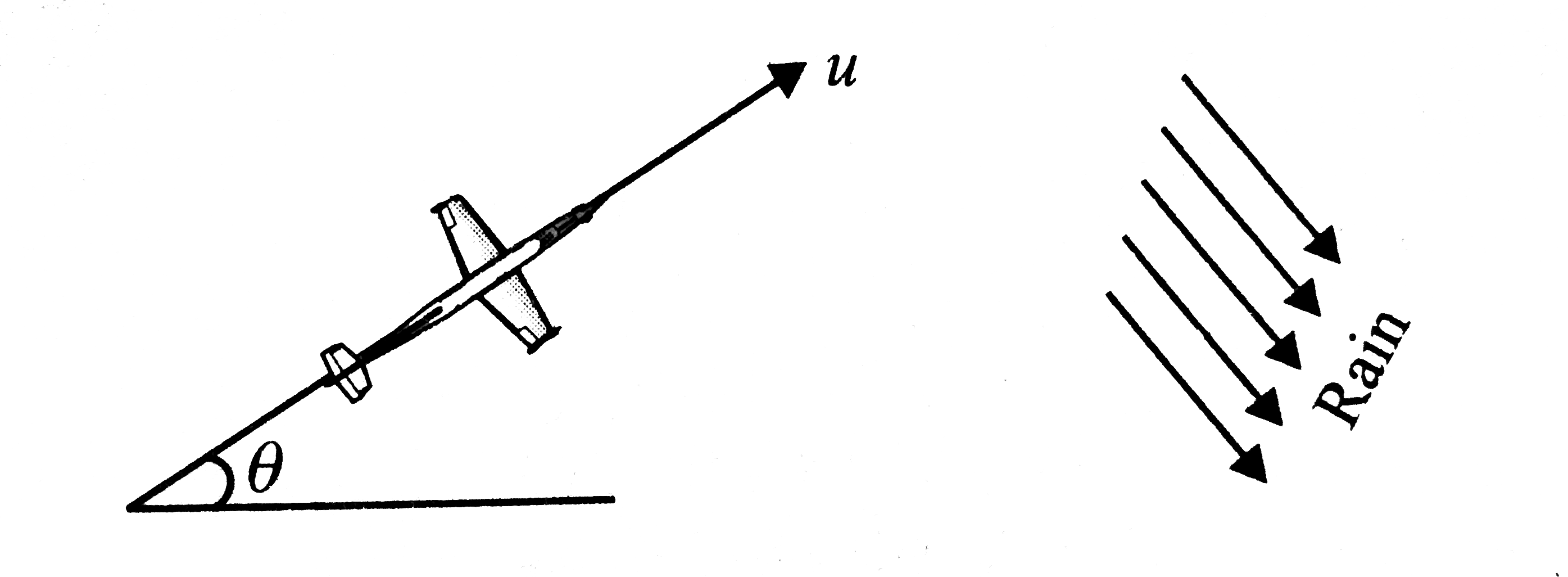
View SolutionRain is falling with a sped of 12√2m/s at an angle 45∘ with the vertical line. A man in a glider going at a speed of u at an angle of 37∘ with respect to the ground. Find the speed of the glider so that rain appears to him falling vertically. Consider the motion of the glider and rain drops in the same vertical plane.
View Solution
