Two trolleys of masses m and 3 m are connected by a spring. The spring is compressed and released the trolleys move off in opposite directions and come to rest after travelling distances s1, and s2, respectively. Assuming coefficient of friction is same for both the ratio of sto s1 to s2 is
1:9
1:3
3:1
9:1
The correct Answer is:C
To solve the problem, we will use the principles of conservation of momentum and the work-energy theorem.
1. Identify the Masses:
- Let the mass of the first trolley be m1=m.
- Let the mass of the second trolley be m2=3m.
2. Apply Conservation of Momentum:
- When the spring is released, the trolleys move in opposite directions. According to the conservation of momentum:
m1v1=m2v2
- This can be rearranged to give:
m1m2=v1v2
- Substituting the values of m1 and m2:
m3m=v1v2⟹13=v1v2
- Thus, we have:
v1=13v2(Equation 1)
3. Apply Work-Energy Principle for Trolley 1:
- The work done by friction on trolley 1 can be expressed as:
12m1v21=μm1gS1
- Rearranging gives:
S1=v212μg
4. Apply Work-Energy Principle for Trolley 2:
- Similarly, for trolley 2:
12m2v22=μm2gS2
- Rearranging gives:
S2=v222μg
5. Find the Ratio S1S2:
- Now, we can find the ratio of the distances:
S1S2=v212μgv222μg=v21v22
- Substituting v1=13v2 into the equation:
S1S2=(v1v2)2=(13)2=19
6. Final Ratio:
- Therefore, the ratio S1:S2 is:
S1:S2=1:9
Conclusion:
The ratio of distances S1 to S2 is 1:9.
- Let the mass of the first trolley be m1=m.
- Let the mass of the second trolley be m2=3m.
- When the spring is released, the trolleys move in opposite directions. According to the conservation of momentum:
m1v1=m2v2
- This can be rearranged to give:
m1m2=v1v2
- Substituting the values of m1 and m2:
m3m=v1v2⟹13=v1v2
- Thus, we have:
v1=13v2(Equation 1)
- The work done by friction on trolley 1 can be expressed as:
12m1v21=μm1gS1
- Rearranging gives:
S1=v212μg
- Similarly, for trolley 2:
12m2v22=μm2gS2
- Rearranging gives:
S2=v222μg
- Now, we can find the ratio of the distances:
S1S2=v212μgv222μg=v21v22
- Substituting v1=13v2 into the equation:
S1S2=(v1v2)2=(13)2=19
- Therefore, the ratio S1:S2 is:
S1:S2=1:9
The ratio of distances S1 to S2 is 1:9.
Similar Questions
Two light trollwys of masses m1 and m2(=3m1) are connected by a spring. The spring is compressed and bound by a cord. The cord is burnt through, the spring expands and the trolleys move off in opposite direction. Assume that the coefficient of friction is the same for both trollwys.
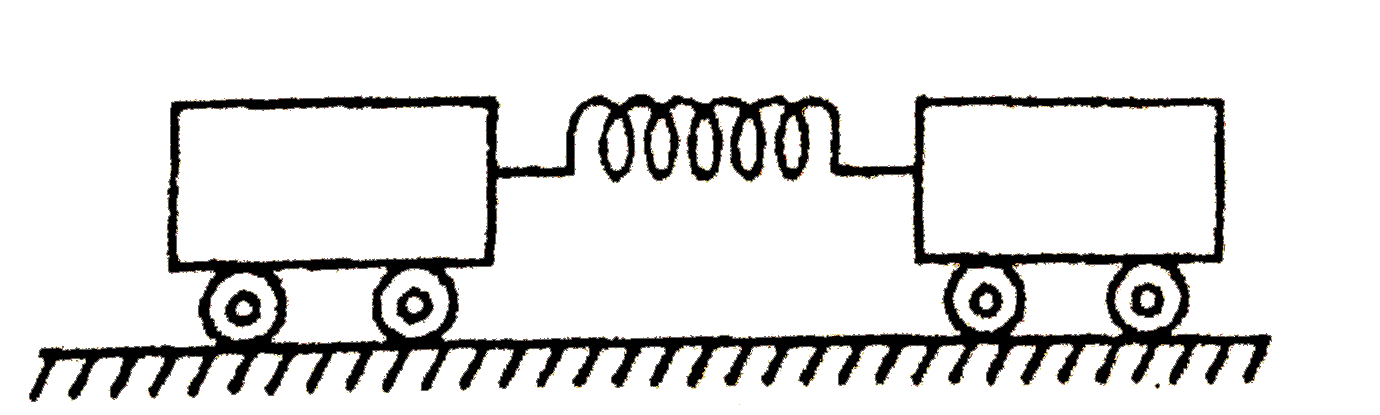
View SolutionA block slides on ice with a velocity of 5m/s comes to rest after moving through a distance of 13.5m . Find the coefficient of friction ?
View SolutionA ball rolled on ice with a velocity of 14 ms−1 comes to rest after travelling 40 m . Find the coefficient of friction . (Given g=9.8m/s2
View SolutionA ball rolled on ice with a velocity of 14ms−1 comes to rest after travelling 20 m. Find the coefficient of friction. (Given g=9.8m/s2)
View SolutionTwo blocks of masses m1 and m2 are connected by a massless spring and placed on smooth surface. The spring initially stretched and released. Then :
View SolutionA body is moving along a rough horizontal surface with an initial velocity 6m/s m If the body comes to rest after travelling 9 m , then the coefficient of sliding friction will be
View SolutionTwo masses m1andm2 re connected by a spring of spring constant k and are placed on as frictionless horizontal surface. Initially the spring is stretched through a distance x0 when the system is released from rest. Find the distance moved by the two masses before they again come to rest.
View SolutionA man standing on a trolley pushes another identical a trolley (both trolleys are at rest on a rough surface), are set in motion and stop alter some time so that they If the ratio of mass of first trolley with man to mass of second trolley is 3, then find the ratio of the stopping distances of the second trolley to that of the first trolley. (Assume coefficient of friction to be the same for both the trolleys)
View SolutionTwo blocks of masses m1 and m2, connected by a weightless spring of stiffness k rest on a smooth horizontal plane as shown in Fig. Block 2 is shifted a small distance x to the left and then released. Find the velocity of centre of mass of the system after block 1 breaks off the wall.

View SolutionTwo particles, of masses M and 2M, moving as shown, with speeds of 10m/s and 5m/s, collide elastically at the origin.After the collision, they move along the indicated directions with speeds v1 and v2 , respectively.The values of v1 and v2 are nearly :

View Solution
