A police car moving at 22ms−1 chases a motor cyclist. The police man sounds horn at 176 Hz. While both of them move towards a stationary siren of frequency 165 Hz. If the number of beats heard by the motor cyclist per second is zero, then the speed of motorcycle is (Speed of sound in air =330ms−1)
33 m/s
22 m/s
0
11m/s
The correct Answer is:B
To solve the problem step by step, we will use the Doppler effect formulas for sound frequency as perceived by moving observers and sources.
Step 1: Understand the Problem
We have a police car moving at a speed of vp=22m/s and sounding a horn at a frequency fp=176Hz. The motorcyclist is moving towards a stationary siren that emits a frequency fs=165Hz. The speed of sound in air is given as v=330m/s. The number of beats heard by the motorcyclist is zero, which means the frequencies heard by the motorcyclist from the police car and the siren are the same.
Step 2: Apply the Doppler Effect for the Police Car
The frequency f′ heard by the motorcyclist from the police car can be calculated using the Doppler effect formula:
f′=fp⋅vv−vp
Substituting the known values:
f′=176⋅330330−22
f′=176⋅330308
Step 3: Apply the Doppler Effect for the Stationary Siren
The frequency f′′ heard by the motorcyclist from the stationary siren is given by:
f′′=fs⋅v+vmv
Where vm is the speed of the motorcyclist. Substituting the known values:
f′′=165⋅330+vm330
Step 4: Set the Frequencies Equal
Since the number of beats heard by the motorcyclist is zero, we set f′=f′′:
176⋅330308=165⋅330+vm330
Step 5: Simplify the Equation
Cross-multiplying gives:
176⋅330=165⋅(330+vm)⋅330308
This simplifies to:
176⋅330⋅308=165⋅330⋅(330+vm)
Step 6: Solve for vm
Expanding and rearranging the equation to isolate vm:
176⋅330⋅308=165⋅3302+165⋅330⋅vm
vm=176⋅330⋅308−165⋅3302165⋅330
Step 7: Calculate vm
Calculating the values:
1. Calculate 176⋅330⋅308.
2. Calculate 165⋅3302.
3. Substitute these values into the equation to find vm.
After performing the calculations, we find:
vm=22m/s
Final Answer
The speed of the motorcycle is 22m/s.
---
We have a police car moving at a speed of vp=22m/s and sounding a horn at a frequency fp=176Hz. The motorcyclist is moving towards a stationary siren that emits a frequency fs=165Hz. The speed of sound in air is given as v=330m/s. The number of beats heard by the motorcyclist is zero, which means the frequencies heard by the motorcyclist from the police car and the siren are the same.
The frequency f′ heard by the motorcyclist from the police car can be calculated using the Doppler effect formula:
f′=fp⋅vv−vp
Substituting the known values:
f′=176⋅330330−22
f′=176⋅330308
The frequency f′′ heard by the motorcyclist from the stationary siren is given by:
f′′=fs⋅v+vmv
Where vm is the speed of the motorcyclist. Substituting the known values:
f′′=165⋅330+vm330
Since the number of beats heard by the motorcyclist is zero, we set f′=f′′:
176⋅330308=165⋅330+vm330
Cross-multiplying gives:
176⋅330=165⋅(330+vm)⋅330308
This simplifies to:
176⋅330⋅308=165⋅330⋅(330+vm)
Expanding and rearranging the equation to isolate vm:
176⋅330⋅308=165⋅3302+165⋅330⋅vm
vm=176⋅330⋅308−165⋅3302165⋅330
Calculating the values:
1. Calculate 176⋅330⋅308.
2. Calculate 165⋅3302.
3. Substitute these values into the equation to find vm.
vm=22m/s
The speed of the motorcycle is 22m/s.
Topper's Solved these Questions
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SET (EXERCISE-VI (LEVEL-II(ADVANCED) STRAIGHT OBJECTIVE TYPE QUESTIONS))
9 Videos
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SET (EXERCISE-VI (LEVEL-II(ADVANCED) MORE THAN ONE CORRECT ANSWERT TYPE QUESTIONS))
4 Videos
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SET (EXERCISE-V (LEVEL-II(ADVANCED) INTEGER TYPE QUESTIONS))
3 Videos
- UNITS AND MEASUREMENTSBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE -3
66 Videos
- WAVE MOTION AND SOUNDBOOK - AAKASH SERIESCHAPTER - WAVE MOTION AND SOUNDEXERCISE - PROBLEMS (LEVEL - II)
97 Videos
Similar Questions
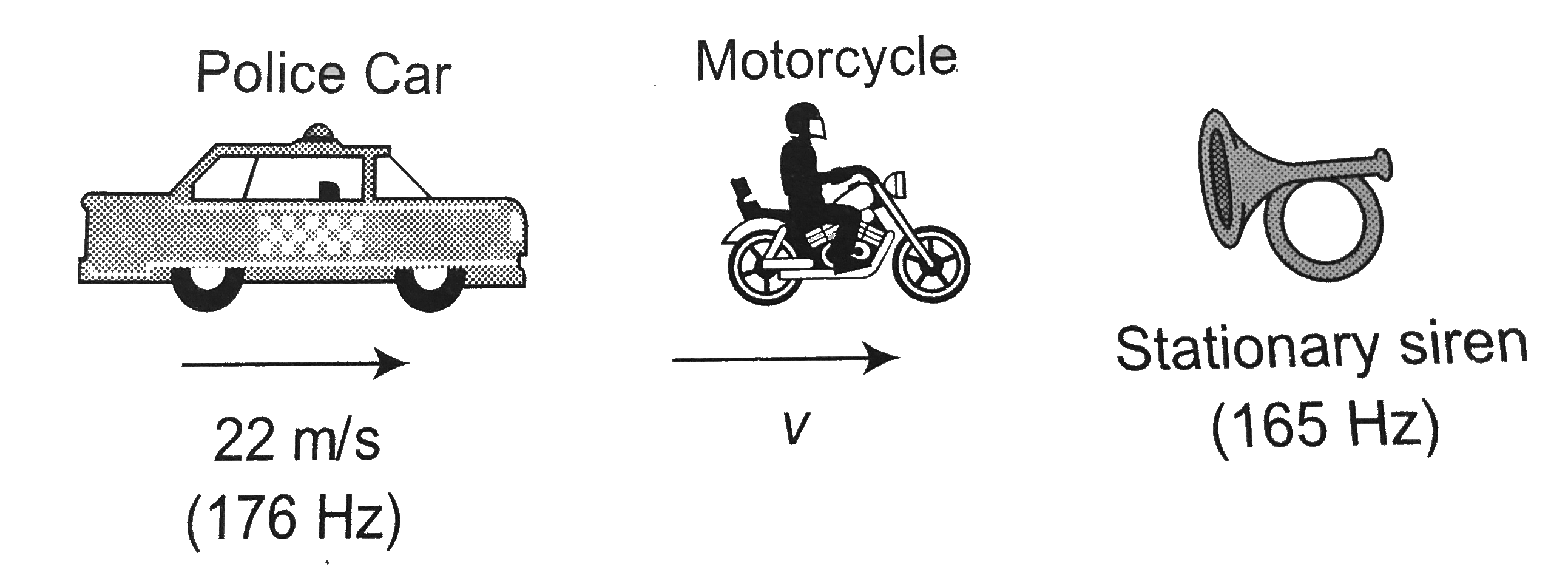
A police car moving at 22m/s, chases a motorcyclist, the police man sounds his horn at 176Hz, while both of them move towards a stationary siren of frequency 165Hz. Calculate the speed of the motorcycle, if it is given that he does not observes any beat
View SolutionA police car moving at 30 m s−1, chases a motorcyclist. The policeman sounds his horn at 180 Hz, while both of them move towards a stationary siren of frequency 160 HZ. He does not observe any beats then, calculate the speed (in m s−1) of the motorcyclist round off two decimal places? [speed of sound =330ms−1]
View SolutionA police car moving at 22m/s, chase a motoclist. The police man has horn at 176HZ, While both of them move towards a stationary siran of frequency 165HZ. Calulate the speed of the motorcyclist, if he does not observer any beats. (velocity of sound in air =330m/s)

View Solutiona bus is moving towards and stationary observer with speed 20 m/s blows a horn of frequency 200 Hz. the frequency of sound heard by the observer is approximately ( take speed of sound 320 m/s)
View SolutionA person riding a car moving at 72kmh−1 sounds a whistle emitting a wave of frequency 1250 Hz. What frequency will be heard by another person standing on the road (a) in front of the car (b) behind the car ? Speed of sound in air =340ms−1 .
View SolutionA car travelling with speed V50 towards a hill, (where v is speed of sound in air), sounds a horn of frequency 196 Hz. The number of beats heard per second by the driver when reflected sound reaches the driver is
View SolutionA bus is moving towards a huge wall with a velocity of 5m/s−1. The driver sounds a horn of frequency 200Hz. The frequency of the beats heard by a passenger of the bus will be ………… Hz (Speed of sound in air =342m/s−1)
View SolutionBus moving with speed v forwards a stationary wall. it produces sound of frequency f=420Hz.Theheardequencyofref≤ctedsoundomwallbydriveris490 Hz.Calca––tethespeedvofbus.Thevelocityofsound∈airis330 m/s.
View SolutionA train is moving with speed 72 km/h towards a hill blows a whistle of frequency 1240Hz. the frequency of echo heard by driver is(speed of sound=330m/s)
View SolutionA person is riding a motorcycle at a speed v towards a stationary car operating its siren at 165Hz. A police car is chasing the person at a speed 22 m/s with its siren operating at 176 Hz. If the motorcyclist does not heard beats, the speed v (m/s) is (speed of sound in air = 330 m/s)
View Solution