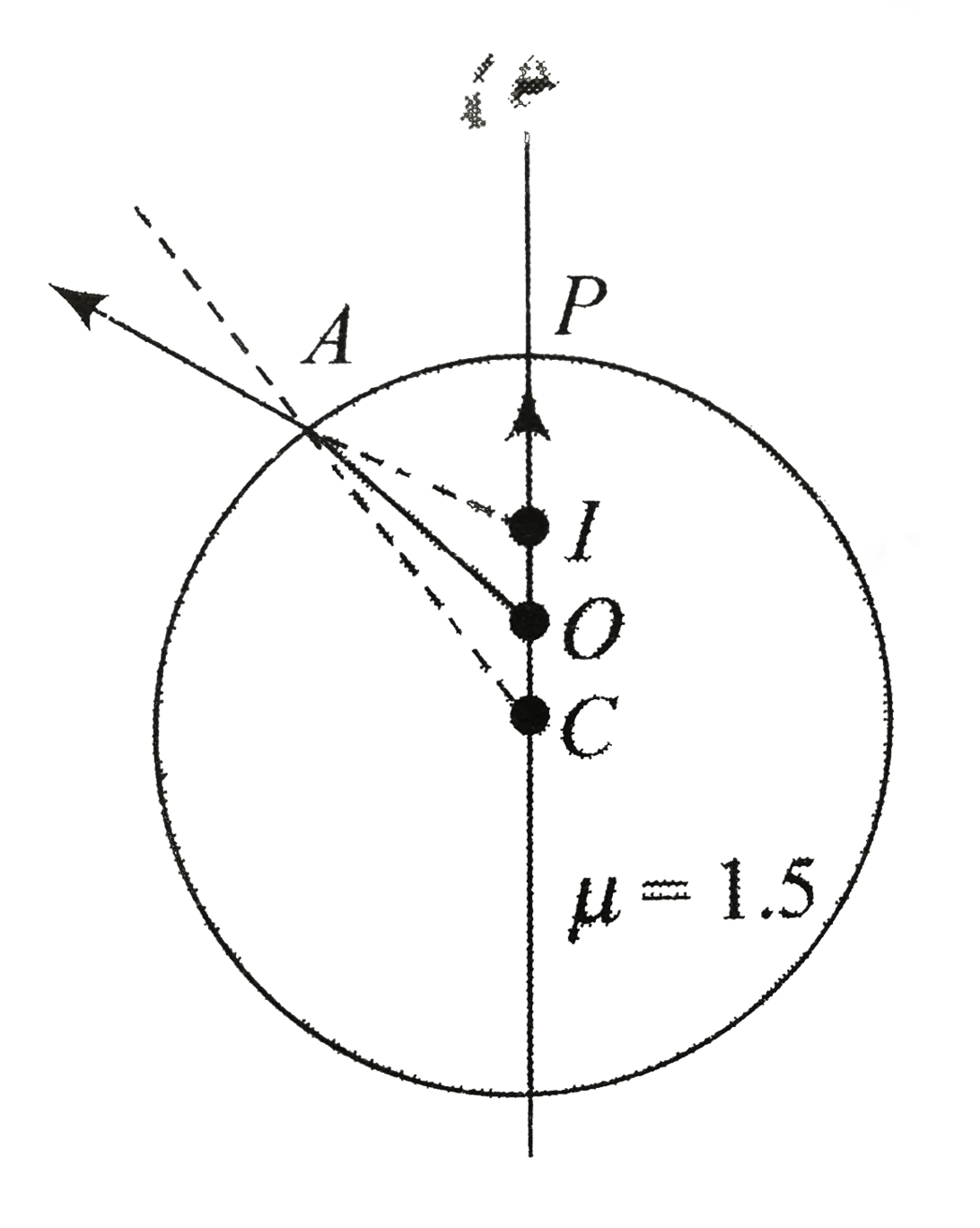
A glass sphere (μ=1.5) of radius 20 cm has a small air bubble 4 cm below its centre. The sphere is viewed from outside and along a vertical line through the bubble. The apparent depth of the bubble below the surface of sphere is (in cm)
13.33
26.67
15
30
The correct Answer is:B
To find the apparent depth of the air bubble located 4 cm below the center of a glass sphere with a refractive index of μ=1.5 and a radius of 20 cm, we can use the formula for apparent depth in a spherical medium.
1. Identify the Given Values:
- Refractive index of air, μ1=1
- Refractive index of glass, μ2=1.5
- Radius of the glass sphere, R=20 cm
- Depth of the bubble below the center of the sphere, d=4 cm
2. Calculate the Actual Depth of the Bubble from the Surface of the Sphere:
- The distance from the center of the sphere to the surface is equal to the radius of the sphere. Therefore, the actual depth of the bubble from the surface of the sphere is:
Actual Depth=R−d=20 cm−4 cm=16 cm
3. Use the Formula for Apparent Depth:
- The formula for apparent depth when viewing through a spherical medium is given by:
Apparent Depth=μ1μ2×Actual Depth
- Substituting the values:
Apparent Depth=11.5×16 cm=161.5 cm=323 cm≈10.67 cm
4. Adjust for the Apparent Depth Below the Surface:
- Since the bubble is viewed from outside the sphere, the apparent depth below the surface of the sphere is:
Apparent Depth Below Surface=R−Apparent Depth=20 cm−10.67 cm≈9.33 cm
5. Final Calculation:
- The apparent depth of the bubble below the surface of the sphere is approximately 9.33 cm. However, since we need to find the depth of the bubble as viewed from outside, we have to consider the total depth from the surface to the bubble, which is:
Total Apparent Depth=20 cm−9.33 cm=10.67 cm
Conclusion:
The apparent depth of the bubble below the surface of the sphere is approximately 10.67 cm.
- Refractive index of air, μ1=1
- Refractive index of glass, μ2=1.5
- Radius of the glass sphere, R=20 cm
- Depth of the bubble below the center of the sphere, d=4 cm
- The distance from the center of the sphere to the surface is equal to the radius of the sphere. Therefore, the actual depth of the bubble from the surface of the sphere is:
Actual Depth=R−d=20 cm−4 cm=16 cm
- The formula for apparent depth when viewing through a spherical medium is given by:
Apparent Depth=μ1μ2×Actual Depth
- Substituting the values:
Apparent Depth=11.5×16 cm=161.5 cm=323 cm≈10.67 cm
- Since the bubble is viewed from outside the sphere, the apparent depth below the surface of the sphere is:
Apparent Depth Below Surface=R−Apparent Depth=20 cm−10.67 cm≈9.33 cm
- The apparent depth of the bubble below the surface of the sphere is approximately 9.33 cm. However, since we need to find the depth of the bubble as viewed from outside, we have to consider the total depth from the surface to the bubble, which is:
Total Apparent Depth=20 cm−9.33 cm=10.67 cm
The apparent depth of the bubble below the surface of the sphere is approximately 10.67 cm.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - LECTURE SHEET (EXERCISE III LEVEL -II (ADVAMCED) MORE THAN ONE CORRECT TYPE QUESTIONS)
1 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - LECTURE SHEET (EXERCISE III LEVEL -II (ADVAMCED) LINKED COMPREHENSION TYPE QUESTIONS)
3 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - LECTURE SHEET (EXERCISE III LEVEL -I (MAIN) STRAIGHT OBJECTIVE TYPE QUESTIONS)
7 Videos
- ELEMENTS OF VECTORSBOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
10 Videos
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE
106 Videos
Similar Questions
A glass sphere of (μ=1.5) with a radius of 15.0cm has a tiny air bubble 5cm above its centre. The sphere is viewed looking down along the extended radius containing the bubble. What is the apparent depth of the bubble below the surface of the sphere?
View SolutionThere is a small air bubble inside a glass sphere (μ=1.5) of radius 10 cm. The bubble is 4.0cm below the surface and is viewd normally from the outside. Find the apparent dipth of the bubble.
View SolutionA glass sphere of radius 5 cm has a small bubble at a distance 2 cm from its centre.The bubble is viewed along a Diameter of the sphere from the side on which it lies.How far from the surface will it appear.Refractive index of glass is 1.5
2.5 cm behind the surface
View SolutionThere is small air bubble inside a glass sphere (μ=1.5) of radius 5cm. The bubble is at 'O' at 7.5cm below the surface of the glass. The sphere is placed inside water (μ=43) such that the top surface of glass is 10cm below the surface of water. The bubble is viewed normally from air. Find the apperent depth of the bubble.

View SolutionA small object is embedded in a glass sphere (mu =1.5) of radius 5.0 cm at a distance 1.5 cm left to the centre. Locate the image of the object as seen by an observer standing (a) to the left of the sphere and (b) to the right of the sphere.
View SolutionA solid transparent sphere made of glass (R.I.=μ>1) has a small, opaque dot at its centre. When observed from outside, apparent position of dot will be
View SolutionA sphere (μ=43) of radius 1m has a small cavity of diameter 1cm at its centre. An observer who is looking at it from tight, sees the magnification of diameter of the cavity as
View SolutionA glass sphere of diameter 50 cm and μ=1.5 has a small air bubble. Looking from outside the diameter, the bubble appears to be at a distance 10 cm the surface. Find the apparent position of the bubble when it is viewed from the diametrically opposite position.
View SolutionA sphere made of transparent material of refractive index (μ=32 )and of radius 50 cm has a small air bubble 10 cm below the surface. The apparent depth of the bubble if viewed from outside normally is
View SolutionA small air bubble is trapped inside a transparent cube of size 12cm. When viewed from one of the vertical faces, the bubble apears to be at 5cm. From it. When viewed from opposite face, it appears at 3cm from it.
View Solution
