How is average velocity different from instantaneous velocity ?
Average velocity:
i)It is defined as ratio Δx displacement to the time interval Δt. ¯vx=ΔxΔt−x2−x1t2t1.
(ii)Average velocity is independent of the path followed by the particle between initial and final positions.
Instantaneous velocity:
i)The velocity of a particle at a particular instant of time is known as instaneous velocity .
i.e., vxLmΔ→0ΔxΔt=dxdt
ii)Instantaneous velocity is the rate of change of position with time.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - Short answer type question16videos
MOTION IN A STRAIGHT LINE
- BOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER7videos
MOTION IN A PLANE
- BOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-III49videos
MOVING CHARGES AND MAGNETISM
Similar Questions
When a particle moves with constant velocity, its average velocity, its instantaneous velocity and its speed are all equal. Comment on this statement.
View SolutionWhen a particle moves with constant velocity its average velocity, its instantaneous velocity and its speed all are equal. Is this statement true or false ?
View SolutionAssertion: When a particle moves with constant velocity its average velocity, its insttantaneous velocity and its speed are all equal in magnitude.
Reason: If the average velocity of a particle moving on a straight line is zero in a time interval, then it is possible that the instantaneous velocity is never zero in the internal.View SolutionFigure shows the graph of the x-coordinaste of a particle going along the X-axis as a function of time.Find a. te average velocity during 0 to 10s, b. instantaneous velocity at 2,5,8 and 12s.
View SolutionA particle moves in x−y plane, starting from A along straight line path AB and BC as shown in the graph. When it is at point P angle between diections of its average velocity and instantaneous velocity is [tan37∘=3/4]
View SolutionA particle is moving with a constant speed in a circular path. Find the ratio of average velocity to its instantaneous velocity when the particle rotates an angle θ=(π2) .
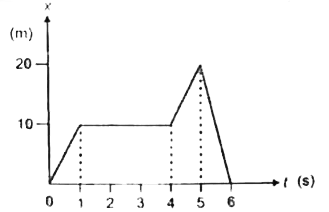
View SolutionFig. shows the graph of the x-coordinate of a particle going along the x-axis as a function of time. Find (a) the average velocity during 0 to 10s, (b) instantaneous velocity at 2, 5, 8 and 12s.
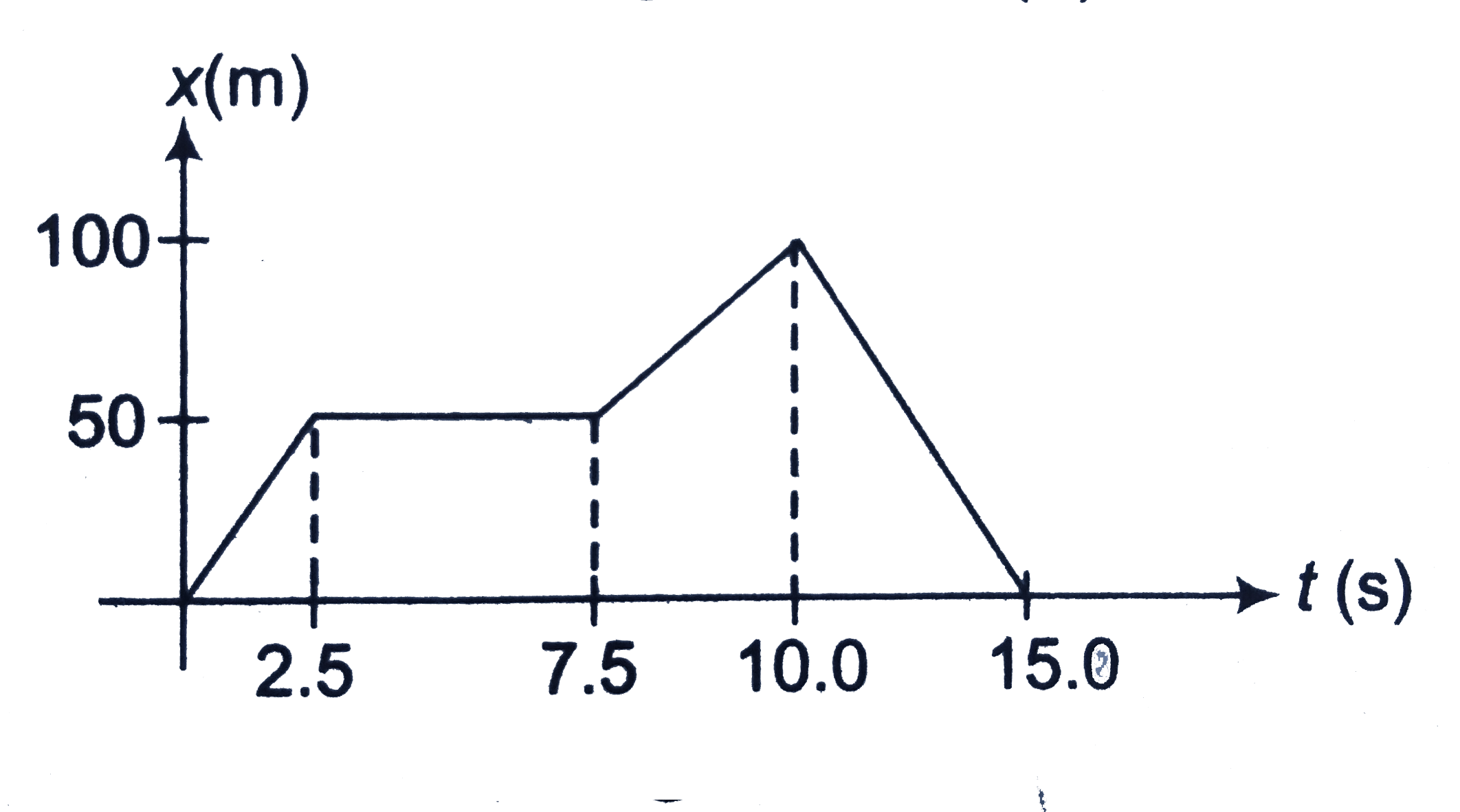
View SolutionFigure shows the graph of x-coordinate of a particle moving along x-axis as a function of time. Average velocity during t = 0 to 4 s and instantaneous velocity at t = 4.113 s respectively will be
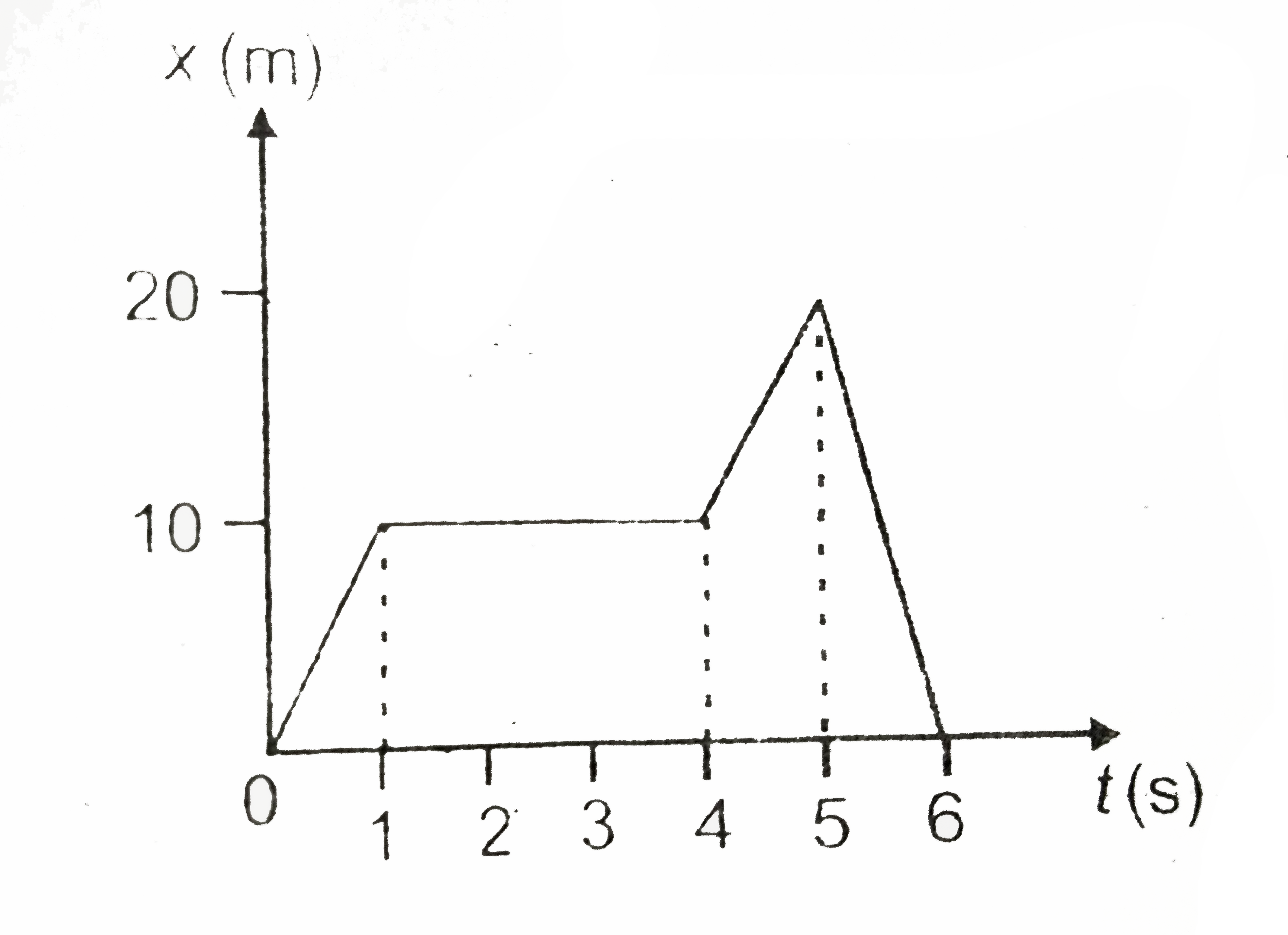
View SolutionFigure shows the graph of x - coordinate of a particle moving along x - axis as a function of time. Average velocity during t = 0 to 6 s and instantaneous velocity at t = 3 s respectively, will be
View SolutionWhat is the difference between average velocity and instantaneous velocity?
View Solution