A force of constant magnitude starts acting on a moving particle when it is at some point 'P'. Depending on the orientation of the force, the particle may
a) pass through point P at some time later
b) not return to point P
c) describe a circular path
d) describe a parabolic path
a is correct
a, b, c, d are correct
c only correct
d only correct
The correct Answer is:B
Step by step video & image solution for A force of constant magnitude starts acting on a moving particle when it is at some point 'P'. Depending on the orientation of the force, the particle may a) pass through point P at some time later b) not return to point P c) describe a circular path d) describe a parabolic path by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - II
136 Videos
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE
106 Videos
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - Application
6 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
10 Videos
- LOGIC GATESBOOK - AAKASH SERIESCHAPTER - LOGIC GATESEXERCISE - Exercise (Very Short Answer)
10 Videos
Similar Questions
Knowledge Check
A particle is moving on a circular path of radius R with constant speed v. During motion of the particle form point A to point B
 AAverage speed is v/2BThe magnitude of average velocity is v/πCThe magnitude of average acceleration is 2v2πRDAverage velocity is zero.
AAverage speed is v/2BThe magnitude of average velocity is v/πCThe magnitude of average acceleration is 2v2πRDAverage velocity is zero.
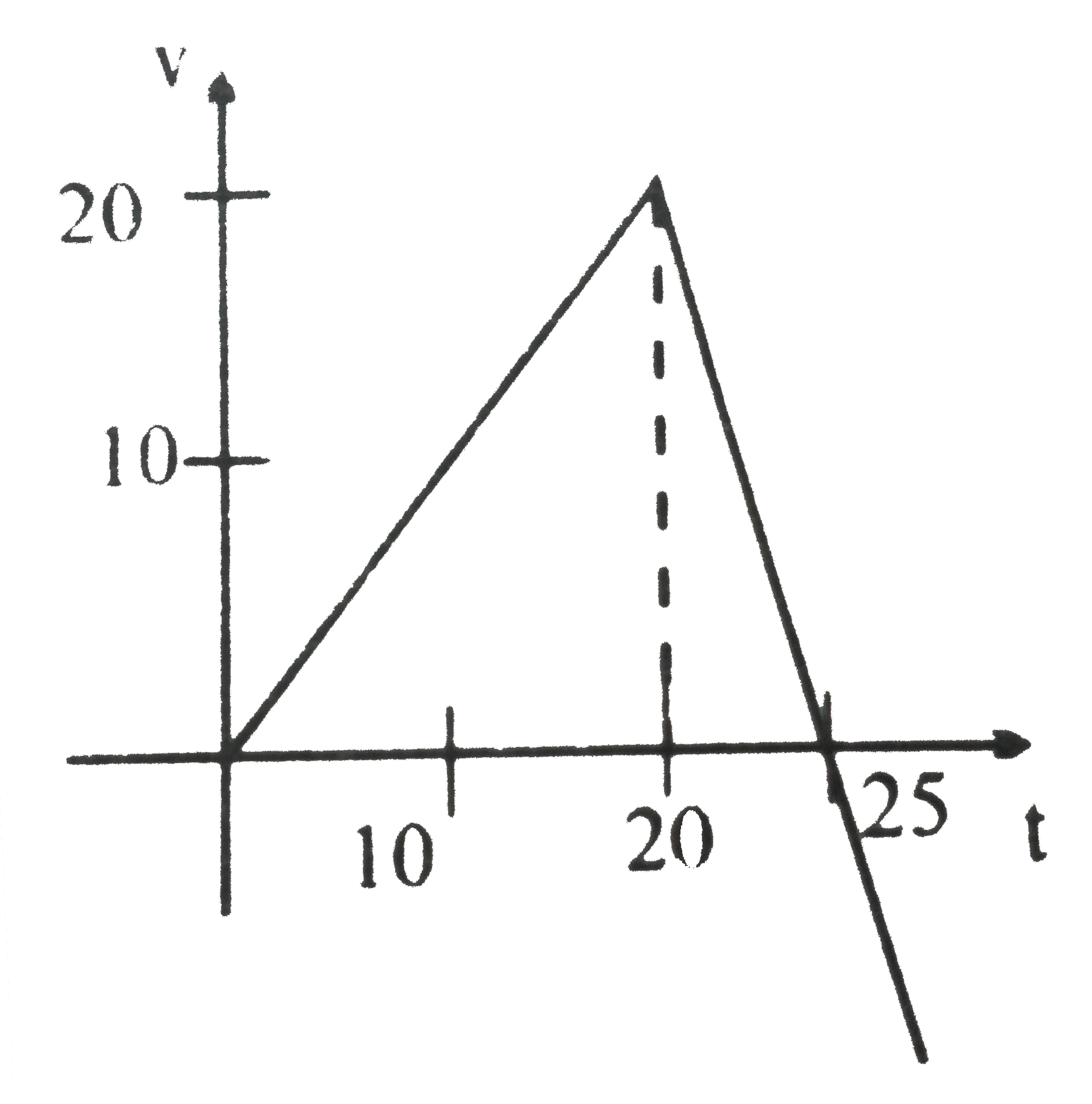
The fig. shows the v−t graph of a particle moving in straight line. Find the time when particle returns to the starting point.
View SolutionA particle tied to a string moves in a circular path in vertical plane. Its velocity at lowerst point is such that it just completes circle. Find the ratio of resultant force on particle at the lowerst point to that at highest point.
View SolutionThe period of a particle executing SHM is T. There is a point P at a distance 'x' from the mean position 'O'. When the particle passes P towards OP, it has speed v. Find the time in which it returns to P again.
View SolutionThe linear momentum P of a particle varies with time as follows P=a+bt2
Where a and b are constants. The net force acting on the particle is
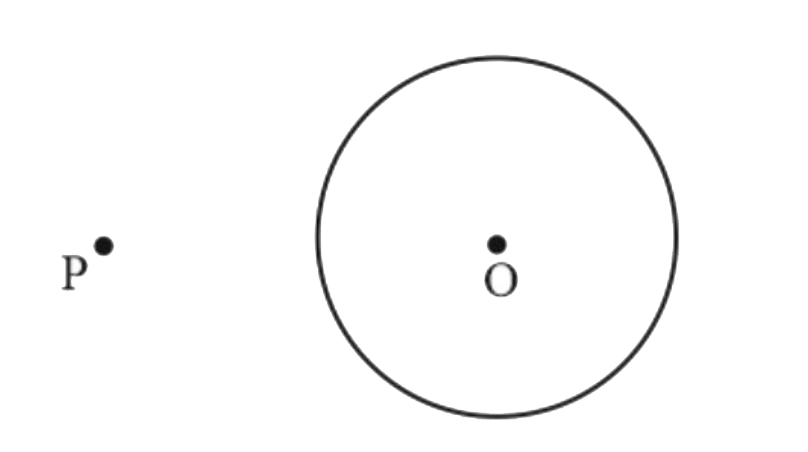
View SolutionConsider a particle , moving in a circle with constant speed . P is a point outside the circle , in the same plane . Then , the angular momentum of the particle about the point P is
View SolutionA point P moves such that the chord of contact of P with respect to the circle x2+y2=4 passes through the point (1, 1). The coordinates of P when it is nearest to the origin are
View SolutionA force →F=(3ˆi+4ˆj) N is acting on a particle. Work done by the force is zero, when particle is moving on the line 2y+Px=2, the value of P is
View Solution
