The electric flux through a Gaussian surface that encloses three charges given by q1=−14nC,q2=78.85nC,q3=−56nC
103Nm2C−1
103CN−1m−2
6.32×103Nm2C−1
6.32×103CN−1m−2
The correct Answer is:A
To find the electric flux through a Gaussian surface that encloses three charges, we can use Gauss's law, which states that the electric flux (Φ) through a closed surface is equal to the total charge (Q_enc) enclosed by that surface divided by the permittivity of free space (ε₀).
1. Identify the Charges:
- We have three charges:
- q1=−14nC=−14×10−9C
- q2=78.85nC=78.85×10−9C
- q3=−56nC=−56×10−9C
2. Calculate the Total Charge Enclosed (Q_enc):
- The total charge enclosed by the Gaussian surface is the sum of the individual charges:
Qenc=q1+q2+q3
Qenc=(−14×10−9)+(78.85×10−9)+(−56×10−9)
Qenc=(−14+78.85−56)×10−9
Qenc=(8.85)×10−9C
3. Use Gauss's Law to Find Electric Flux (Φ):
- According to Gauss's law, the electric flux is given by:
Φ=Qencϵ0
- Where ϵ0 (the permittivity of free space) is approximately 8.85×10−12C2/N m2.
4. Substituting Values:
Φ=8.85×10−98.85×10−12
5. Simplifying the Expression:
- The 8.85 in the numerator and denominator cancels out:
Φ=1×10−91×10−12=103N m2/C
6. Final Result:
- Thus, the electric flux through the Gaussian surface is:
Φ=1000N m2/Cor103N m2/C
- We have three charges:
- q1=−14nC=−14×10−9C
- q2=78.85nC=78.85×10−9C
- q3=−56nC=−56×10−9C
- The total charge enclosed by the Gaussian surface is the sum of the individual charges:
Qenc=q1+q2+q3
Qenc=(−14×10−9)+(78.85×10−9)+(−56×10−9)
Qenc=(−14+78.85−56)×10−9
Qenc=(8.85)×10−9C
- According to Gauss's law, the electric flux is given by:
Φ=Qencϵ0
- Where ϵ0 (the permittivity of free space) is approximately 8.85×10−12C2/N m2.
Φ=8.85×10−98.85×10−12
- The 8.85 in the numerator and denominator cancels out:
Φ=1×10−91×10−12=103N m2/C
- Thus, the electric flux through the Gaussian surface is:
Φ=1000N m2/Cor103N m2/C
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Exercise
57 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise-I
80 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
42 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)
26 Videos
Similar Questions
In the given figure, there are four point charges placed at the vertices of a square of side, a=1.4m. If q1=+18nC,q2=−24nC,q3+35nCandq4=+16nCm then find the electric at the centre P of the square Assume the potential to be zero at infinity.

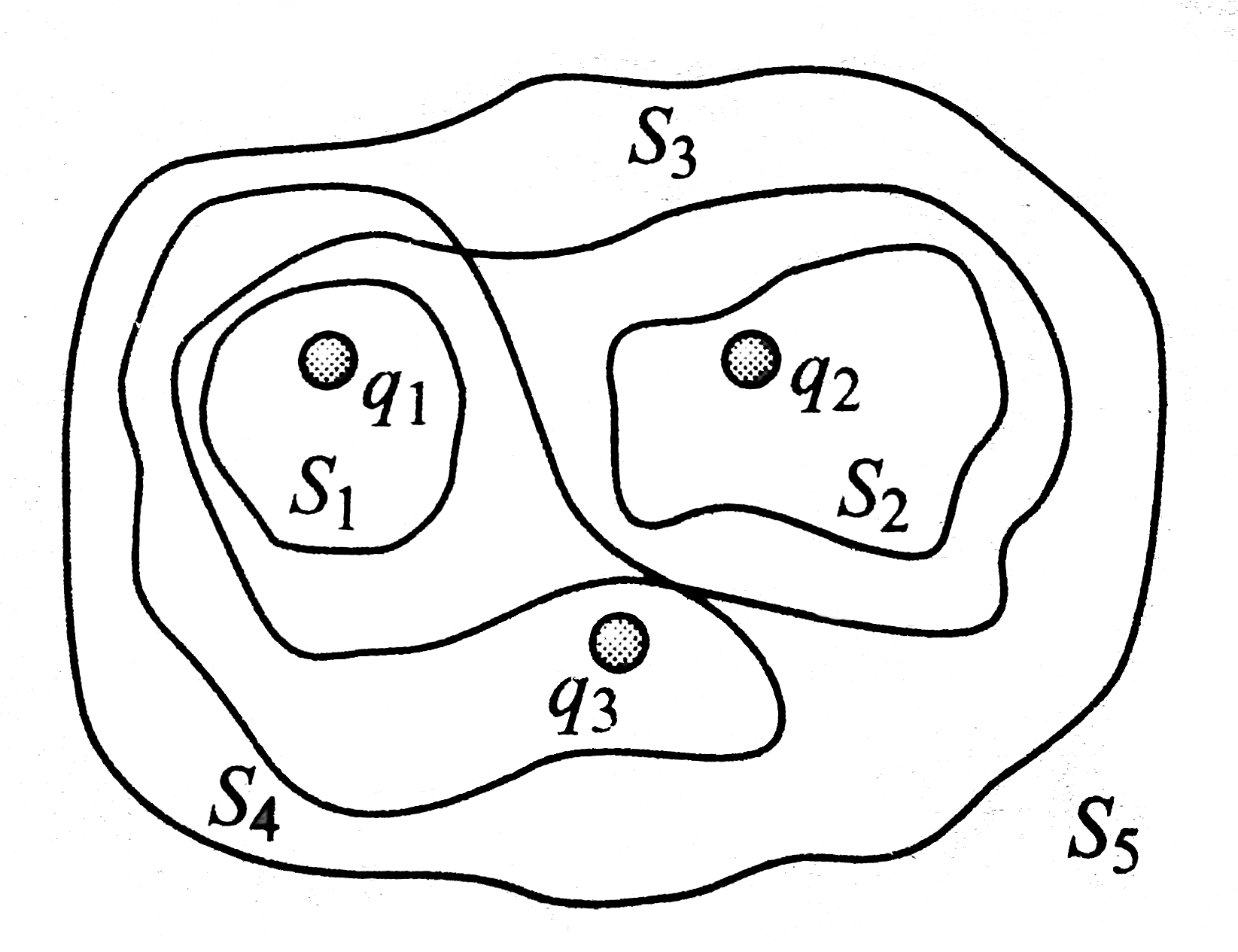
View SolutionThe three small spheres as shown in figure carry charges q1=4nC,q2=−7.8nCandq3=2.4nC. Find the net electic flux through each of the following closed surfaces shown in cross section in the figure.
a. S1 b.S2
c. S3 d. S4
e. S5.
Do your answers to parts from (a) to (e) depend on how the charge is distributed over each small sphere? Why or why not?
 .
.
View SolutionConsider a Gaussian spherical surface covering a dipole of charge q and -q, then

View SolutionThree concentric metallic spherical shells of radii R, 2R, 3R are given charges Q1Q2Q3, respectively. It is found that the surface charge densities on the outer surface of the shells are equal. Then, the ratio of the charges given to the shells Q1:Q2:Q3 is
View SolutionGauss's law and Coulomb's law , although expressed in different forms , are equivalent ways of describing the relation between charge and electric field in static conditions . Gauss's law is ε0ϕ=qencl,when q(encl) is the net charge inside an imaginary closed surface called Gaussian surface. The two equations hold only when the net charge is in vaccum or air .
If the charge q3 and q4 are displaced (always remaining outside the Gaussian surface), then consider the following two statements :
A: Electric field at each point on the Gaussian surface will remain same .
B: The value of ∮→E.d→A for the Gaussian surface will remain same.
View SolutionIf a current of 0.5 apere flows through a metallic wire for 2 hour then how many electrons flow through the wire ?
View SolutionThe electric force on a charge q1 due to q2 is (4ˆi−3ˆj)N. What is the force on q2 due to q1
View SolutionA Gaussian surface S encloses two charges q1=qandq2=−qthe field at p is
where →E1,→E2and→E3 are the field contributed by q1,q2andq3at P respectively.

View SolutionThe electric field at (30, 30) cm due to a charge of −8nC at the origin in NC−1 is
View SolutionThree charges are placed at the vertices of an equilateral triangle of side 10 cm. Assume q1=1μC,q2=−2μCandq3=4μC. Work done in separating the charges to infinity is
View Solution
