Two identical capacitors are connected in series. Charge on each capacitor is q0. A dielectric slab is now introduced between the plates of one of the capacitors so as to fill the gap, the battery remaining connected. The charge in each capacitor will now be
2q01+1K
q01+1K
2q01+K
q01+K
The correct Answer is:A
To solve the problem, we need to analyze the situation step by step.
Step 1: Understand the Initial Setup
We have two identical capacitors, C1 and C2, connected in series. The charge on each capacitor is given as q0. The voltage across the series combination of capacitors can be expressed as:
V=V1+V2
where V1 and V2 are the voltages across capacitors C1 and C2, respectively.
Step 2: Relate Charge and Voltage
For capacitors in series, the charge on each capacitor is the same, so:
V1=q0CandV2=q0C
Thus, the total voltage across the series combination is:
V=q0C+q0C=2q0C
Step 3: Introduce the Dielectric
Now, a dielectric slab with dielectric constant K is introduced between the plates of one of the capacitors (let's say C2). The capacitance of C2 will now change to:
C′2=K⋅C
Step 4: Analyze the New Configuration
Now, the new charge on C1 remains q′ (which we will find), and the charge on C2 (with the dielectric) will be q′ as well. The voltage across C2 with the dielectric becomes:
V′2=q′K⋅C
Thus, the total voltage across the series combination is now:
V=q′C+q′K⋅C
Step 5: Set Up the Equation
We can factor out q′:
V=q′(1C+1K⋅C)=q′⎛⎝1+1KC⎞⎠
This simplifies to:
V=q′C(1+1K)
Step 6: Relate New Charge to Old Charge
From the initial setup, we know:
V=2q0C
Equating the two expressions for V:
2q0C=q′C(1+1K)
Cancelling C from both sides gives:
2q0=q′(1+1K)
Solving for q′:
q′=2q01+1K=2q0KK+1
Conclusion
The new charge on each capacitor after the introduction of the dielectric slab is:
q′=2q0KK+1
We have two identical capacitors, C1 and C2, connected in series. The charge on each capacitor is given as q0. The voltage across the series combination of capacitors can be expressed as:
V=V1+V2
where V1 and V2 are the voltages across capacitors C1 and C2, respectively.
For capacitors in series, the charge on each capacitor is the same, so:
V1=q0CandV2=q0C
Thus, the total voltage across the series combination is:
V=q0C+q0C=2q0C
Now, a dielectric slab with dielectric constant K is introduced between the plates of one of the capacitors (let's say C2). The capacitance of C2 will now change to:
C′2=K⋅C
Now, the new charge on C1 remains q′ (which we will find), and the charge on C2 (with the dielectric) will be q′ as well. The voltage across C2 with the dielectric becomes:
V′2=q′K⋅C
Thus, the total voltage across the series combination is now:
V=q′C+q′K⋅C
We can factor out q′:
V=q′(1C+1K⋅C)=q′⎛⎝1+1KC⎞⎠
This simplifies to:
V=q′C(1+1K)
From the initial setup, we know:
V=2q0C
Equating the two expressions for V:
2q0C=q′C(1+1K)
Cancelling C from both sides gives:
2q0=q′(1+1K)
Solving for q′:
q′=2q01+1K=2q0KK+1
The new charge on each capacitor after the introduction of the dielectric slab is:
q′=2q0KK+1
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (CUTTENT ELECTRICITY )108videos
APPENDICES ( REVISION EXERCISE )
- BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (MOVING CHANGES & MEGNETISM)95videos
APPENDICES ( REVISION EXERCISE )
- BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (MAGNETISM AND MATTER )52videos
APPENDICES ( REVISION EXERCISE )
- BOOK - AAKASH SERIESCHAPTER - ALTERNATING CURRENTEXERCISE - EXERCISE - III24videos
ALTERNATING CURRENT
- BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - LAW OF MOTION128videos
APPENDICES (REVISION EXERCISE)
Similar Questions
A capacitor and a bulb are connected in series with a source of alternating emf. If a dielectric slab is inserted between the plates of the capacitor, then
View SolutionA dielectric slab is inserted between the plates of an isolatted charged capacitor. Which of the following quantities will remain the same?
View SolutionTwo identical parallel plate capacitors are connected in series to a battery of 100N . A dielectric slab of dielectric constant 4.0 is inserted between the plates of second capacitor. The potential difference across the capaitors will now be respectively.
View SolutionTwo identical capacitors A and B shown in the given circuit are joined in series with a battery. If a dielectric slab of dielectric constant K is slipped between the plates of capacitor B and battery remains connected, then the energy of capacitor A will-
 View Solution
View SolutionTwo identical parallel plate capacitors are connected in series and then joined in series with a battery of 100V. A slab of dielectric constant K=3 is inserted between the plates of the first capacitor. Then, the potential difference across the capacitor will be, respectively.
View SolutionA dielectric slab is inserted between the plates of a capacitor. The charge on the capacitor is Q and the magnitude of the induced charge on each surface of the dielectric is Q'.
View SolutionTwo identical capacitor C1 and C2 are connected in series with a battery. They are fully charged. Now a dielectric slab is inserted between the plates of C2. The potential across C1 will :
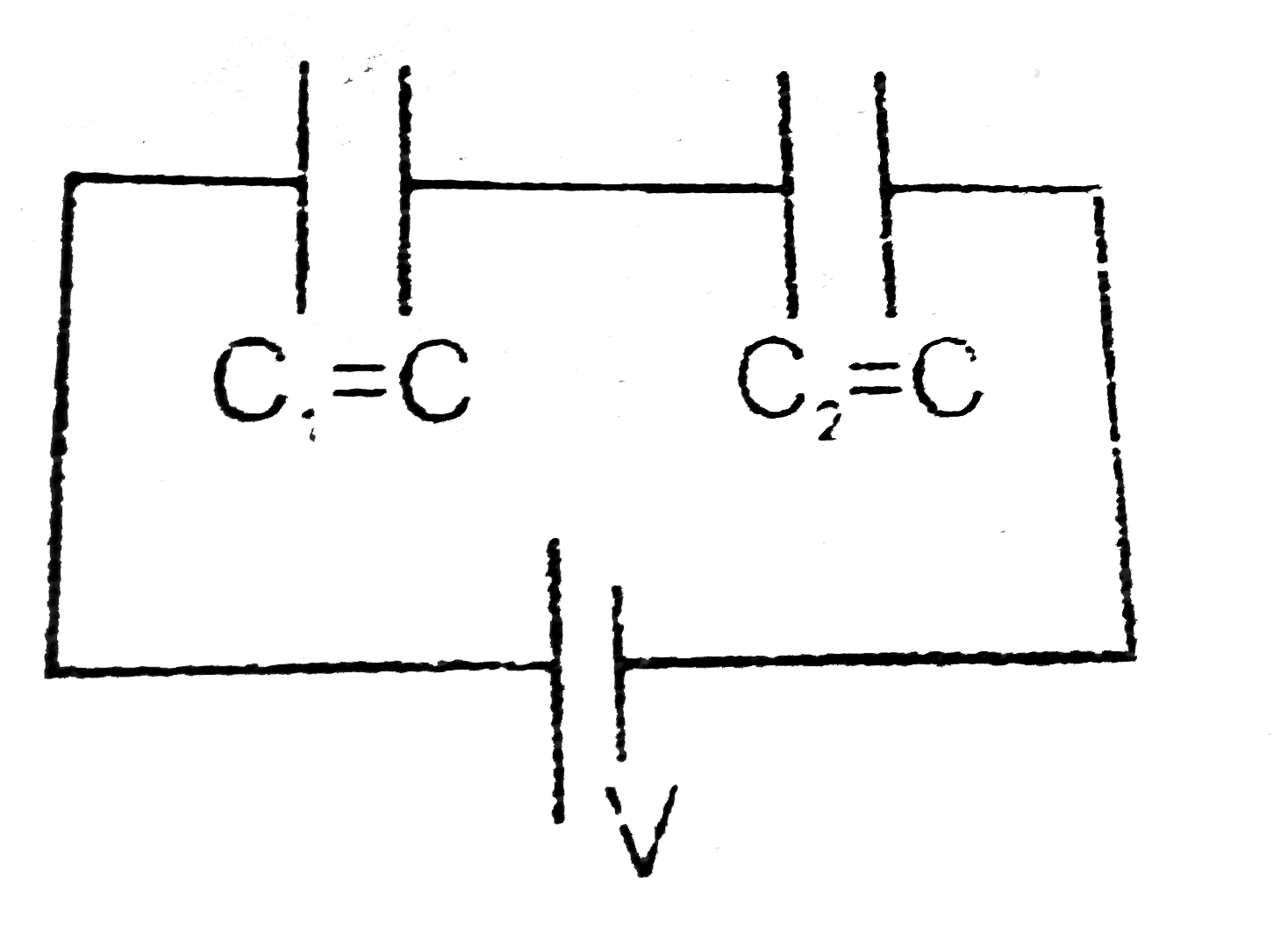 View Solution
View SolutionTwo identical parallel plate capacitors are connected in series to a battery of 100V. A dielectric slab of dielectric constant 4.0 is inserted between the plates of second capacitor. The potential difference across the capacitors will now be respectively
View SolutionAll the capacitors were uncharged before they are connected in circuit. Now the charge on 4μF capacitor is
 View Solution
View SolutionA parallel plate capacitor is connected across a battery. Now, keeping the battery connected, a dielectric slab is inserted between the plates. In the process,
View Solution
