A student finds the balancing length to be 'l' with a cell of constant emf in the secondary circuit. Another student connects the same cell in the secondary circuit of potentiometer of double the length but with a cell of half the emf in the primary circuit. The balancing length will be [cell in primary is ideal and no series resistance is present in primary circuit.]
4l
l/4
2l
l/2
The correct Answer is:A
To solve the problem, we need to analyze the situation step by step.
Step 1: Understanding the First Case
In the first case, a student finds the balancing length to be L with a cell of constant EMF E in the secondary circuit. The potential difference across the potentiometer wire is given by:
V=I×L
where I is the current flowing through the potentiometer.
Step 2: Setting Up the Second Case
In the second case, the same cell is connected in the secondary circuit of a potentiometer that has double the length (i.e., 2L). However, the EMF in the primary circuit is now half the original value, E2.
Step 3: Analyzing the Potential Difference in the Second Case
For the second case, the potential difference across the new potentiometer wire of length 2L is:
V′=I′×(2L)
where I′ is the new current flowing through the circuit.
Step 4: Relating the Currents and EMFs
Since the EMF in the secondary circuit remains the same as the first case (which is E), we can set up the following relationship:
I×L=E(from the first case)
I′×(2L)=E(from the second case)
Step 5: Finding the Current in the Second Case
From the first case, we can express the current I as:
I=EL
For the second case, substituting I′ gives:
I′=E2L
Step 6: Setting Up the Balancing Length Equation
Now, we can relate the two cases using the balancing condition:
I×L=I′×L′
Substituting the expressions for I and I′:
EL×L=E2L×L′
This simplifies to:
E=E2L×L′
Step 7: Solving for the Balancing Length L′
Cancelling E from both sides (since E≠0):
1=L′2L
Thus, we find:
L′=2L
Step 8: Final Calculation for Balancing Length
Now, since the EMF in the primary circuit is half, we need to adjust our previous equation:
I′=E/22L=E4L
Substituting this back gives us:
L′=4L
Conclusion
The balancing length in the second case will be 4L.
Final Answer
The balancing length will be 4L.
---
In the first case, a student finds the balancing length to be L with a cell of constant EMF E in the secondary circuit. The potential difference across the potentiometer wire is given by:
V=I×L
where I is the current flowing through the potentiometer.
In the second case, the same cell is connected in the secondary circuit of a potentiometer that has double the length (i.e., 2L). However, the EMF in the primary circuit is now half the original value, E2.
For the second case, the potential difference across the new potentiometer wire of length 2L is:
V′=I′×(2L)
where I′ is the new current flowing through the circuit.
Since the EMF in the secondary circuit remains the same as the first case (which is E), we can set up the following relationship:
I×L=E(from the first case)
I′×(2L)=E(from the second case)
From the first case, we can express the current I as:
I=EL
For the second case, substituting I′ gives:
I′=E2L
Now, we can relate the two cases using the balancing condition:
I×L=I′×L′
Substituting the expressions for I and I′:
EL×L=E2L×L′
This simplifies to:
E=E2L×L′
Cancelling E from both sides (since E≠0):
1=L′2L
Thus, we find:
L′=2L
Now, since the EMF in the primary circuit is half, we need to adjust our previous equation:
I′=E/22L=E4L
Substituting this back gives us:
L′=4L
The balancing length in the second case will be 4L.
The balancing length will be 4L.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (MOVING CHANGES & MEGNETISM)95videos
APPENDICES ( REVISION EXERCISE )
- BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (MAGNETISM AND MATTER )52videos
APPENDICES ( REVISION EXERCISE )
- BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (ELECTRIC POTENTIAL & CAPACITANCE )143videos
APPENDICES ( REVISION EXERCISE )
- BOOK - AAKASH SERIESCHAPTER - ALTERNATING CURRENTEXERCISE - EXERCISE - III24videos
ALTERNATING CURRENT
- BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - LAW OF MOTION128videos
APPENDICES (REVISION EXERCISE)
Similar Questions
When a Daniel cell is connected in the secondary circuit of a potentiometer, the balancing length is found to be 540 cm. If the balancing length becomes 500 cm. When the cell is short-circuited with 1Ω, the internal resistance of the cell is
View SolutionThe length of potentiometer wire is 200 cm and the emf of standard cell is primary circuit is E volts. It is employed to a battery of emf 0.4 v the balance point is obtained at l = 40 cm from positive end, the E of the battery is (cell in primary is ideal and series resistance is zone
View Solution
Knowledge Check
- Question 1 - Select One
In a potentiometer the balancing with a cell is at length of 220cm. On shunting the cell with a resistance of 3Ω balance length becomes 130cm. What is the internal resistance of this cell.
A4.5ΩB7.8ΩC6.3ΩD2.08Ω
In a potentiometer experiment the balancing with a cell is at length 240 cm. On shunting the cell with a resistance of 2Ω, the balancing length becomes 120 cm.The internal resistance of the cell is
View SolutionWhen 6 identical cells of no internal resistance are connected in series in the secondarycircuit of a potentio meter, the balancing lengths is .l., balancing length becomes 1/3 when some cells are connected wrongly, the number of cells connected wrongly are
View SolutionA cell in the secondary circuit gives null deflection for 2.5 m length of a potentiometer having 10m length of wire. If the length of the potentiometer wire is increased by 1m without changing the cell in the primary, the position of the null point wil be
View SolutionFind the reading of the ideal ammeter connected in the given circuit. Assume that the cells have negligible internal resistance.
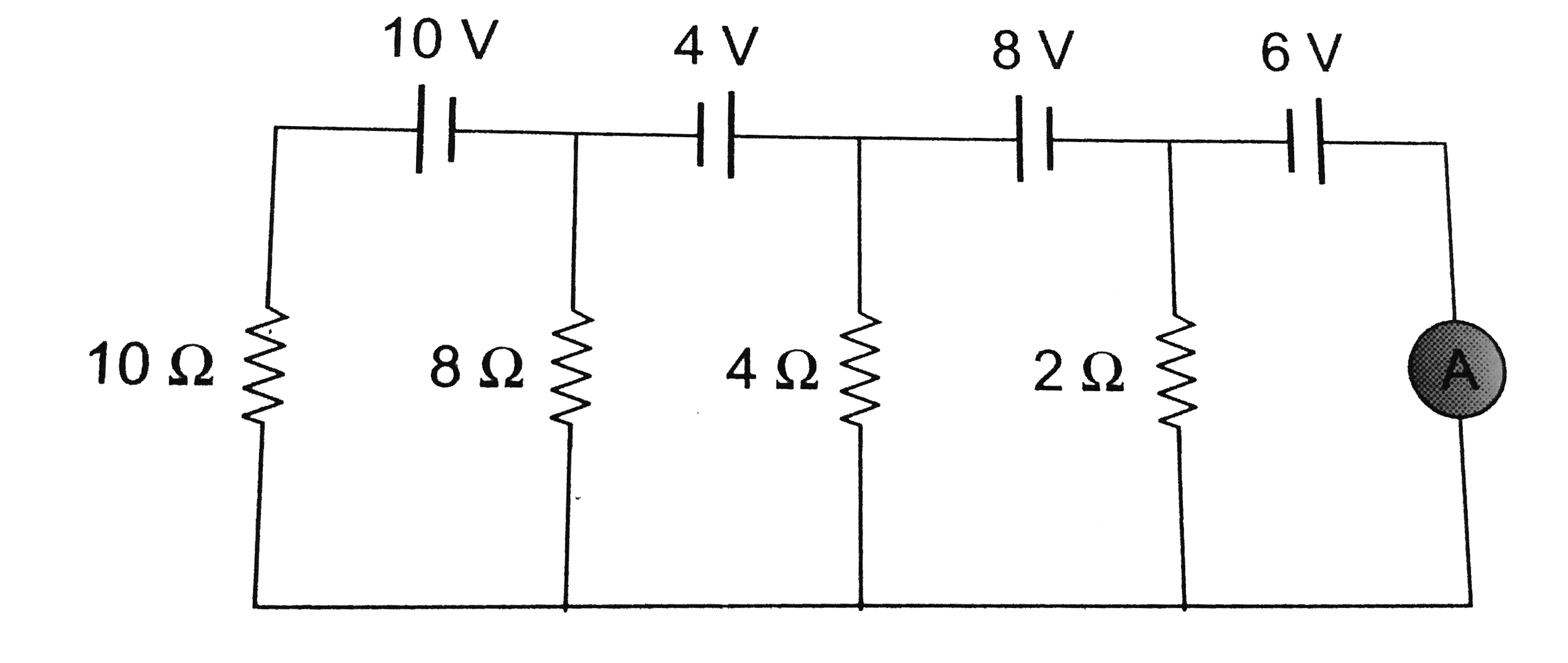 View Solution
View SolutionThe wire of potentiometer has resistance 4Ω and length 1m. It is connected to a cell of emf 2 volt and internal resistance 1Ω. If a cell of emf 1.2 volt is balanced by it, the balancing length will be
View SolutionThe mutual inductance between a primary and secondary circuit is 0.5H. The resistance of the primary and the secondary circuits are 20ohms and 5ohms respectvely. To genrate a current of 0.4A in the secondary,current in the primary must be changed at the rate of
View SolutionThe emf of a cell is 2V and its internal resistance is 2 ohm. A resistance of 8 ohm is joined to battery in parallel. This is contacted in secondary circuit of potentio meter. If IV standard cell balances for 100cm of potentiometer wire, the balance point of above cell is
View Solution
