A full-wave p-n diode rectifier uses a load resistor of 1500Ω. No filter is used. The forward bias resistance of the diode is 10Ω. The efficiency of the rectifier is
81.2%
40.6%
80.4%
40.2%
The correct Answer is:C
To solve the problem of finding the efficiency of a full-wave p-n diode rectifier, we will follow these steps:
Step 1: Understand the formula for efficiency
The efficiency (η) of a full-wave rectifier can be calculated using the formula:
η=0.812×RLRL+RF×100
where:
- RL is the load resistance,
- RF is the forward bias resistance of the diode.
Step 2: Identify the given values
From the problem, we have:
- Load resistance, RL=1500Ω
- Forward bias resistance, RF=10Ω
Step 3: Substitute the values into the formula
Now we substitute the values into the efficiency formula:
η=0.812×15001500+10×100
Step 4: Calculate the denominator
Calculate RL+RF:
RL+RF=1500+10=1510Ω
Step 5: Substitute and calculate the efficiency
Now we can substitute this back into the formula:
η=0.812×15001510×100
Step 6: Calculate the numerator
Calculate 0.812×1500:
0.812×1500=1218
Step 7: Divide by the denominator
Now divide by 1510:
12181510≈0.80795
Step 8: Multiply by 100 to get percentage
Now multiply by 100 to convert to percentage:
η≈0.80795×100≈80.8%
Step 9: Round to the nearest option
Rounding this value gives us approximately 80.4%.
Final Answer
Thus, the efficiency of the rectifier is approximately 80.4%, which corresponds to option C.
---
The efficiency (η) of a full-wave rectifier can be calculated using the formula:
η=0.812×RLRL+RF×100
where:
- RL is the load resistance,
- RF is the forward bias resistance of the diode.
From the problem, we have:
- Load resistance, RL=1500Ω
- Forward bias resistance, RF=10Ω
Now we substitute the values into the efficiency formula:
η=0.812×15001500+10×100
Calculate RL+RF:
RL+RF=1500+10=1510Ω
Now we can substitute this back into the formula:
η=0.812×15001510×100
Calculate 0.812×1500:
0.812×1500=1218
Now divide by 1510:
12181510≈0.80795
Now multiply by 100 to convert to percentage:
η≈0.80795×100≈80.8%
Rounding this value gives us approximately 80.4%.
Thus, the efficiency of the rectifier is approximately 80.4%, which corresponds to option C.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - EXERCISE -II (TRANSISTORS )9videos
SEMICONDUCTOR DEVICES
- BOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - EXERCISE -II (LOGIC GATES)12videos
SEMICONDUCTOR DEVICES
- BOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - EXERCISE -II (ENERGY BANDS AND CLASSIFICATION OF SOLIDS )3videos
SEMICONDUCTOR DEVICES
- BOOK - AAKASH SERIESCHAPTER - RAY OPTICSEXERCISE - PROBLEMS ( LEVEL-II)60videos
RAY OPTICS
- BOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTEXERCISE - PRACTICE EXERCISE45videos
UNITS AND MEASUREMENT
Similar Questions
A fullwave P.N diode rectifier used load resistor of 1500Ω. No filter is used. Assume each diode to have idealized characteristic with Rf=10Ω and Rr=ac. Since wave voltage applied to each diode has amplitude of 30 volts and frequency 50 Hz. Calculate.
(i) Peak, d.c rms load current
(ii) d.c power output
(iii) A.C power input
(iv) Rectifier efficiency.View SolutionA sinusoidal voltage of amplitude 25 volts and frequency 50 Hz is applied to a half wave rectifier using PN diode. No filter is used and the load resistor is 1000Ω. The forward resistance Rf ideal diode is 10Ω. Calculate.
(i) Peak, average and rms values of load current
(ii) d.c power output
(ii) a.c power input
(iv) % Rectifier efficiency
(v) Ripple factor.View Solution
Knowledge Check
- Question 1 - Select One
The circuit shown in the figure contains two diodes each with a forward resistance of 30 Ω and with infinite backward resistance. If the battery is 3 V, the current through the 50 Ω resistance (in ampere) is
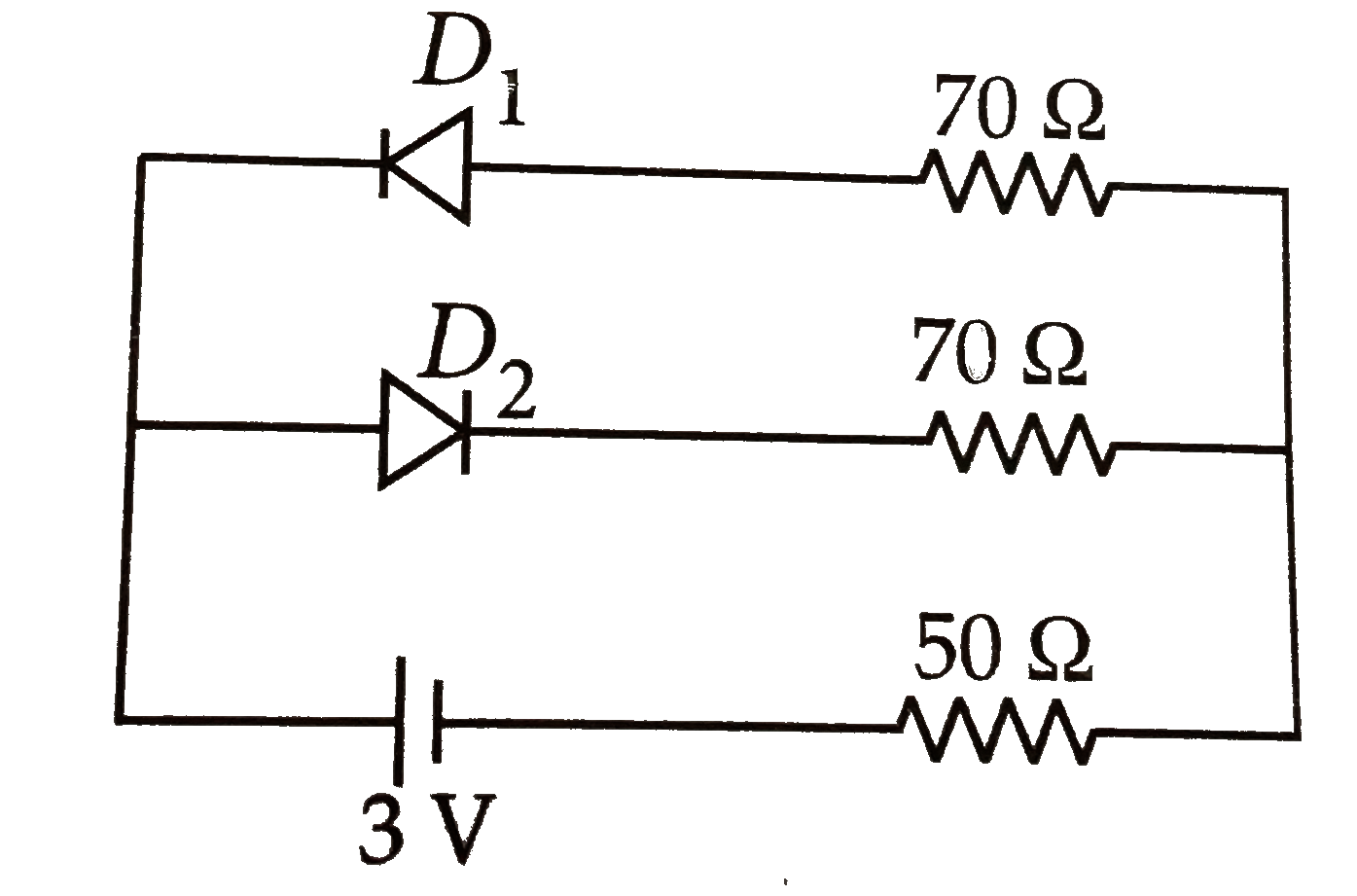 AzeroB0.01C0.02D0.03
AzeroB0.01C0.02D0.03
A p-n junction in series with a resistance of 5kΩ is connected across a 50 V DC source . If the forward bias resistance of the junction is 50Ω , the forward bias current is
View SolutionTwo batteries with emf 12 V and 13V are connected in parallel across a load resistor of 10Ω . The internal resistances of the two batteries are 1Ωand2Ω respectively . The voltage across the load lies between
View SolutionWhat is the ratio of forward and reverse resistance of p-n junction diode?
View Solutionp−n junction diode can be used as
View SolutionIn input in a full - wave rectifier is e=50sin(314t) volt, diode resistance is 100Ω and load resistance is 1kΩ then, pulse frequency of output voltage is
View SolutionForward bias characteristics of a p -n junction diode is used in which of the following devices ?
View SolutionA full wave rectifier circuit along with the input and output are shown in the figure. The contribution from the diode D2 is (are)
View Solution

