Two speakers C and E are placed 5 m apart and are driven by the same source. Let a man stand at A which is 10 m away from the mid point O of C and E. The man walks towards the point O which is at 1 m (parallel to OC) as shown in the figure. He receives the first minimum in sound intensity at B. Then calculate the frequency of the source. (Assume speed of sound = 343 m s−1)
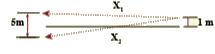
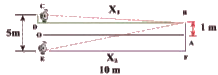
The first minimum occurs when the two waves reaching the point B are 180∘ (out of phase). The path difference Δx=λ2.
In order to calculate the path difference, we have to find the path lengths x1 and x2.
In a right triangle BDC,
DB = 10m and OC=12(5)=2.5m
CD=OC−1=(2.5m)−1m=1.5m
x1=√(10)2+(1.5)2=√100+2.25
=√102.25=10.1m
In a right triangle EFB,
DB = 10m and OE=12(5)=2.5m=FA
FB = FA + AB = (2.5 m) + 1 m = 3.5 m
x2=√(10)2+(3.5)2=√100+12.25=√112.25 = 10.6m
The path difference Δx=x2−x1 = 10.6 m - 10.1m = 0.5m. Required that this path difference
Δx=λ2=0.5⇒λ=1.0m
To obtain the frequency of source, we use
v=λf⇒f=vλ=3431=343Hz
= 0.3 kHz
Topper's Solved these Questions
- WAVESBOOK - NCERT TAMILCHAPTER - WAVESEXERCISE - EVALUATION(I. MULTIPLE CHOICE QUESTIONS)
15 Videos
- WAVESBOOK - NCERT TAMILCHAPTER - WAVESEXERCISE - EVALUATION(IV. NUMERICAL PROBLEMS)
7 Videos
- WAVESBOOK - NCERT TAMILCHAPTER - WAVESEXERCISE - EVALUATION(IV. NUMERICAL PROBLEMS)
7 Videos
- UNITS AND MEASUREMENTBOOK - NCERT TAMILCHAPTER - UNITS AND MEASUREMENTEXERCISE - EXERCISE (ADDITIONAL EXERCISE)
9 Videos
- WORK, ENERGY AND POWERBOOK - NCERT TAMILCHAPTER - WORK, ENERGY AND POWEREXERCISE - EXERCISE - IV. NUMERICAL PROBLEMS
5 Videos
Similar Questions
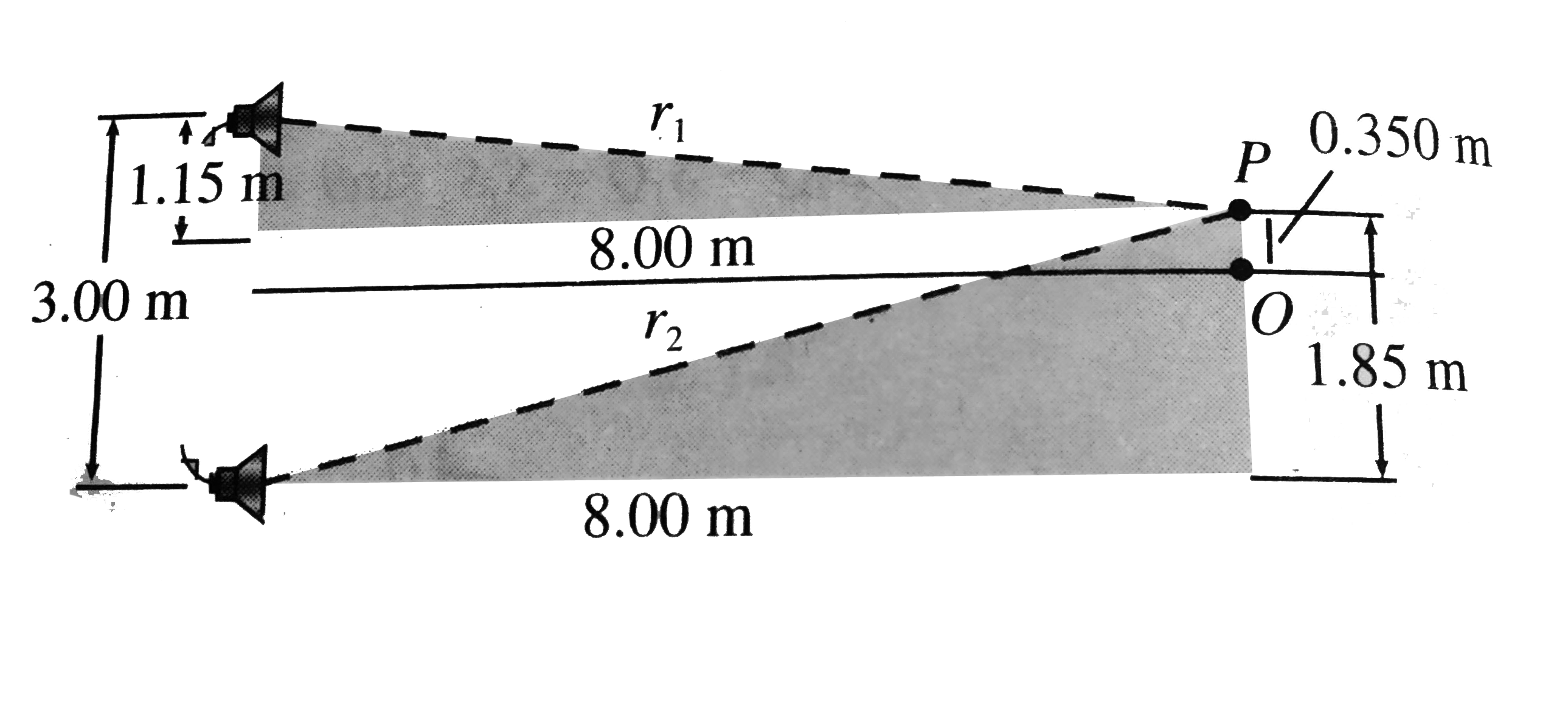
Two identical loudspeakers placed 3.00m apart are driven by the same oscillator as shown in Fig. 7.12. A listener is originally at point O , located 8.00m from the centre of the line connecting the two speakers. The listener then moves to point P, which is a perpendicular distance 0.350m from O , and she experiences the first minimum sound intensity . What is the frequency of the oscillator ?
View SolutionTwo speakers are driven by the same oscillator with frequency of 200 Hz. They are located 4 m part on a vertical pole. A man walks straight towards the lower speaker in a direction perpendicular to the pole, as shown in figure. (i) How many times will he hear a minimum in sound intensity, and (ii) how far is he from the pole and at these moments ? Take the speed of sound to be 330 m/s, and ignore any sound reflections coming off the ground.
_E01_139_Q01.png) .
.
View SolutionTwo speakers are driven by the same oscillator with frequency of 200 Hz. They are located 4 m part on a vertical pole. A man walks straight towards the lower speaker in a direction perpendicular to the pole, as shown in figure. (i) How many times will he hear a minimum in sound intensity, and (ii) how far is he from the pole and at these moments ? Take the speed of sound to be 330 m/s, and ignore any sound reflections coming off the ground.
_E01_139_Q01.png) .
.
View SolutionThe distance between the two identical speakers shown in Fig. 14.3.3 are 4.00m. They are driven by the same osicllator. Initially a listner stays at L at a distance 8.0 m from the centre of the line connecting the two speakers. The listner then walks to the point A where AL=0.4m. The first minium is sound intensity in heard just the listener reaches the point A. Find the frequency of the oscillator. Speed of sound in air is 340 m/s.
View SolutionA man standing 25 m away from a wall produces a sound and receives the reflected sound.
Calculate the time after which he receives the reflected sound if the speed of sound in air is 350 m s−1.
View SolutionA man standing 25 m away from a wall produces a sound and receives the reflected sound.
Calculate the time after which he receives the reflected sound if the speed of sound in air is 350ms−1.
View SolutionThe sound level at a point 5-0 m away from a point source is 40 dB. What will be the level at a point 50 m away from the source ?
View SolutionThe sound level at a point 5.0 m away from a point source is 40 dB. What will be the level at a point 50 m away from the source ?
View SolutionTwo speakers S1 and S2 are a driven by same source. You walk along a line l that is perpendicular bisector of the line joining the two speakers and record the intensity at different points. Then you walk along a line m that is parallel to the line joining the speakers and record the intensity of sound at various points. On which path you observe the loudness to alternate between faint and loud? Explain.

View SolutionA person standing at a distance of 6 m from a source of sound receives sound wave in two ways, one directly from the source and other after reflection from a rigid boundary as shown in the figure. The maximum wavelength for which, the person will receive maximum sound intensity, is
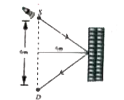
View Solution
