Check the dimensional of the wave y = sin(x-vt). If it is dimensionally wrong, write the above equation in the correct form.
Text Solution
Dimensionally it is not correct. we know that y = sin(x-vt) must be a dimensionless quantity but x-vt has dimension. The correct equation is y= sin (k x-ωt), where k and ω have the dimensions of inverse of length and inverse of time respectively. The sine functions and cosine functions are periodic functions with period 2π. Therefore, the correct expression is y=sin(2πλx−2πTt) where λ and T are wavelength and time period, respectively. In general, y(x,t)=Asin(kx−ωt)
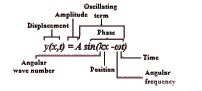 .
.
|
Topper's Solved these Questions
- WAVESBOOK - NCERT TAMILCHAPTER - WAVESEXERCISE - EVALUATION(I. MULTIPLE CHOICE QUESTIONS)
15 Videos
- WAVESBOOK - NCERT TAMILCHAPTER - WAVESEXERCISE - EVALUATION(IV. NUMERICAL PROBLEMS)
7 Videos
- WAVESBOOK - NCERT TAMILCHAPTER - WAVESEXERCISE - EVALUATION(IV. NUMERICAL PROBLEMS)
7 Videos
- UNITS AND MEASUREMENTBOOK - NCERT TAMILCHAPTER - UNITS AND MEASUREMENTEXERCISE - EXERCISE (ADDITIONAL EXERCISE)
9 Videos
- WORK, ENERGY AND POWERBOOK - NCERT TAMILCHAPTER - WORK, ENERGY AND POWEREXERCISE - EXERCISE - IV. NUMERICAL PROBLEMS
5 Videos
Similar Questions
Knowledge Check
From the dimensional consideration, which of the following equations is correct?
AT=2π√R3GMBT=2π√GMR3CT=2π√GMR2DT=2π√R2GMFrom the dimensional consideration which of the following equation is correct ?
AT=2π√R3GMBT=2π√GMR3CT=2π√GMR2DT=2π√R2GMWhich of the following equations is dimensionally correct?
Apressure = Engery per units volumeBpressure = Energy per units areaCpressure = Momentum × Volume × timeDpressure = Force × area
A : All physically correct equations are dimensionally correct.
R : All dimensionally correct equations are physically correct.
View SolutionThe dimensional formula for ω in the relation y=ASinωt is
View SolutionA : All physically correct equations are dimensionally correct.
R : All dimensionally correct equations are physically correct.
View SolutionAssertion: A dimensionally wrong or inconsistaent equation must be wrong.
Reason: A dimensionally consistent equation is a exact or a correct equation.
View SolutionAssertion: A dimensionally wrong or inconsistaent equation must be wrong.
Reason: A dimensionally consistent equation is a exact or a correct equation.
View Solution
