Locate the center of mass of a uniform rod of mass M and length A.
Consider a uniform rod of mass M and length A whose one end coincides with the origin as shown in Figure. The rod is kept along the x axis. To find the center of mass
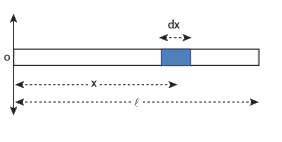
of this rod, we choose an infinitesimally small mass dm of elemental length dx at a distance x from the origin.
λ is the linear mass density (i.e. mass per unit length) of the rod. λ=Ml
The mass of small element (dm) is, dm =Mldx
Now, we can write the center of mass equation for this mass distribution as,
xCM=∫xdm∫dm
xCM=l∫ox(Mldx)M=1ll∫oxdx
=1l[x22]10=1l(l22)
xCM=l2
As the position l2 is the geometric center of the rod, it is concluded that the center of mass of the uniform rod is located at its geometric center itself.
Topper's Solved these Questions
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES BOOK - NCERT TAMILCHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES EXERCISE - EXERCISE (I. MULTI CHOICE QUESTION)
15 Videos
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES BOOK - NCERT TAMILCHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES EXERCISE - EXERCISE (V. NUMERICAL PROBLEMS)
6 Videos
- MOTION IN A STRAIGHT LINEBOOK - NCERT TAMILCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ADDITIONAL EXERCISES
5 Videos
- NATURE OF PHYSICAL WORLD AND MEASUREMENTBOOK - NCERT TAMILCHAPTER - NATURE OF PHYSICAL WORLD AND MEASUREMENTEXERCISE - EXERCISE (V. Conceptual Questions)
5 Videos
Similar Questions
Show that the centre of mass of uniform rod of mass M and length L lies at the middle point of the rod.
View SolutionCalculate the moment of inertia of a uniform rod of mass M and length l about an axis passing through an end and perpendicular to the rod. The rod can be divided into a number of mass elements along the length of the rod.

View Solution
Knowledge Check
Find the M.I. of thin uniform rod of mass M and length L about the axis as shown.
 A
A B
B C
C D
D
Choose the most appropriate option.
The potential energy of gravitational interaction of a point mass m and a thin uniform rod of mass M and length L, if they are located along a straight line at a distance a from each other, isAU=GMmaln(a+Lα)BU=GMm(1a−1a+L)CU=−GMmaDU=−GMmLln(a+La)
Calculate the moment of inertia of a uniform rod of mass M and length l about an axis passing through an end and perpendicular to the rod. The rod can be divided into a number of mass elements along the length of the rod.

View SolutionCentre Of Mass Of Uniform Rod
View SolutionFind the potential energy of the gravitational interation of a point mass of mass m and a thin uniform rod of mass M and length l, if they are located along a straight line such that the point mass is at a distance 'a' from one end. Also find the force of their interaction.
View SolutionFind the potential energy of the gravitational interation of a point mass of mass m and a thin uniform rod of mass M and length l, if they are located along a straight line such that the point mass is at a distance 'a' from one end. Also find the force of their interaction.
View SolutionTwo identical uniform rods AB and CD, each of length L are jointed to form a T-shaped frame as shown in figure. Locate the centre of mass of the frame. The centre of mass of a uniform rod is at the middle point of the rod.
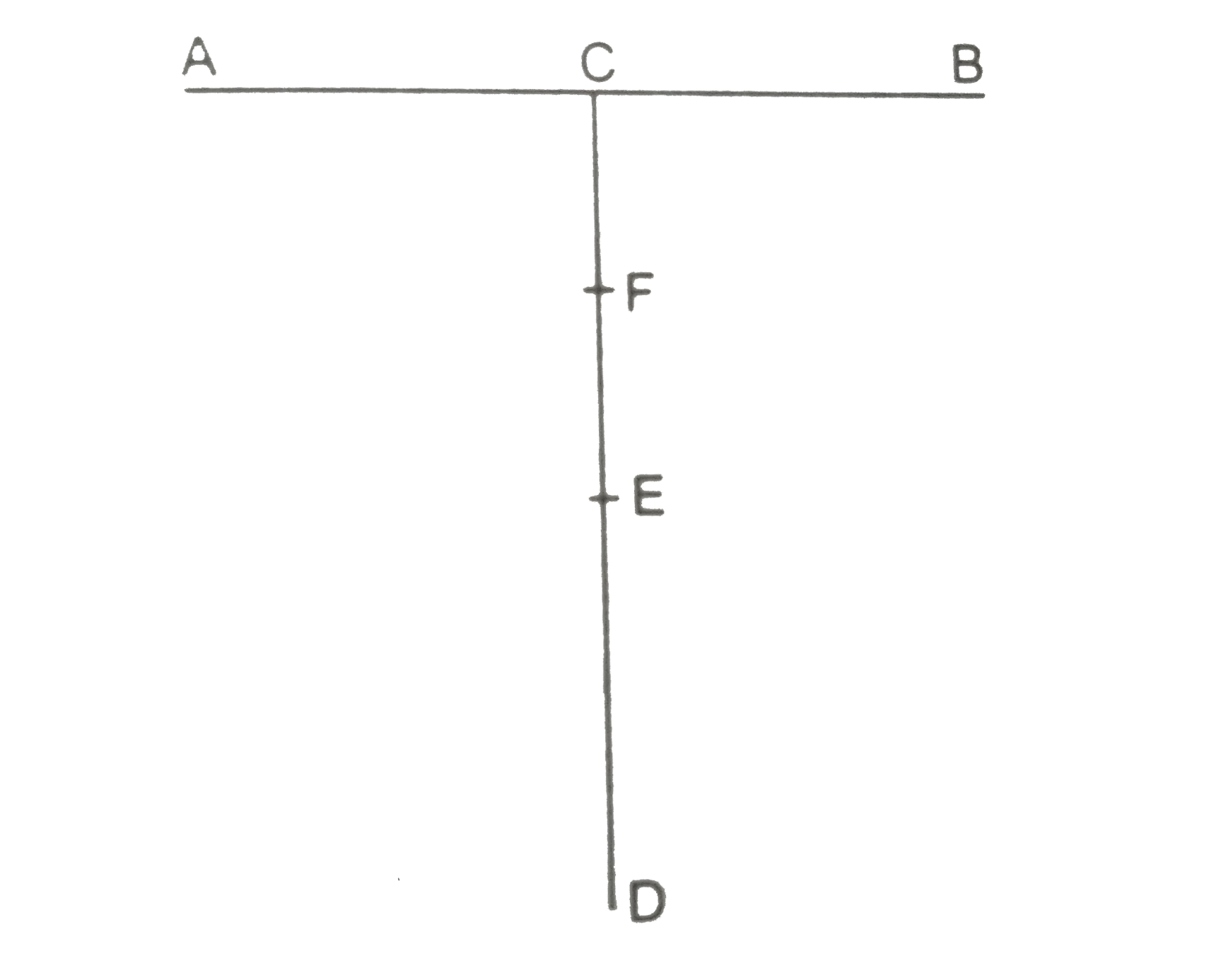
View SolutionTwo identical uniform rods AB and CD, each of length L are jointed to form a T-shaped frame as sown in figure. Locate the centre of mass of the frame. The centre of mass of a uniform rod is at the middle point of the rod.

View Solution
