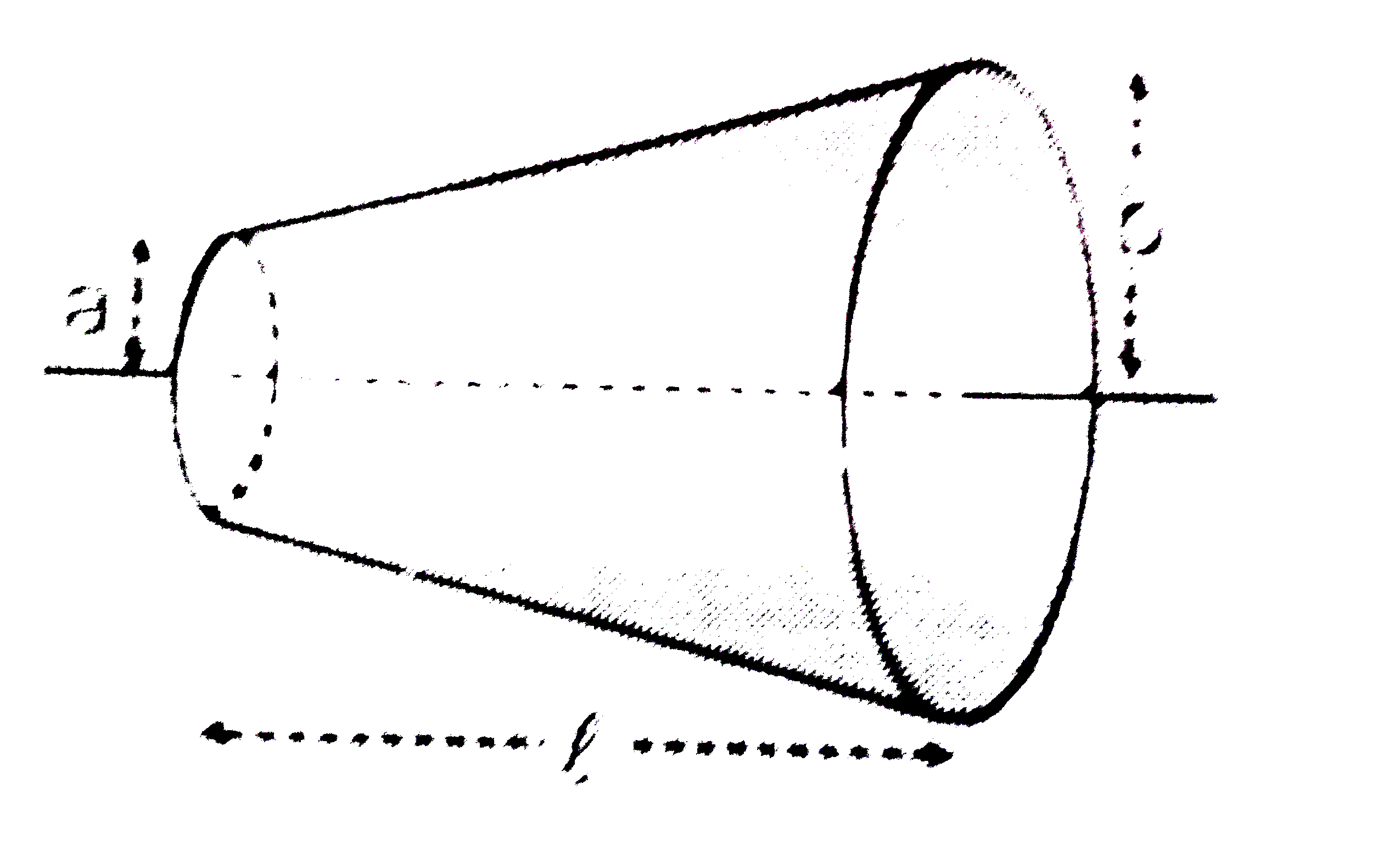
Figure shows a conductor of length l carrying current i and having a circular cross-section. The radius of cross section varies linearly from a to b. assuming that $(b - a) < < l$ calculate current density at distance x from left end.
Solution in Gujrati

Video Solution

Text Solution

Verified by Experts

$d R d u e \to t h e s m a l l s t r i p d x a t a d i s \tan c e < b r > y,$ R = (rhodx/ y_2) $\dots \dots . (i) < b r >$ tan theta = ((y-a)/x) = ((b-a)/L) $< b r >$ rArr ((y-a)/x) = ((b-a)/L) $< b r >$ rArr L xx ( y-a) = x(b-a) $< b r >$ rArr Ly - La = xb - xa $< b r >$ rArr L dy/dx -0 = b-a (diff. w.r. t. x) $< b r >$ dx = Ldy/ (b-a) $< b r > P u \in g t h e v a l u e o f d x \in e q n . (i)$
$d R = (ρ L \frac{d y}{π} (y^{2}) (b - a))$
$d R = (ρ \frac{L}{π} (b - a)) . \frac{d y}{y^{2}}$
$\Rightarrow (\int_{0}^{R}) d R = (ρ \frac{L}{π} (b - a)) (\int_{a}^{b}) \frac{d y}{y^{2}}$
$R = (ρ \frac{L}{π} (b - a)) ({[- \frac{1}{y}]}_{a}^{b})$
$= (ρ \frac{L}{π} (b - a)) (- \frac{1}{b}) (- \frac{1}{a})$
$(ρ \frac{L}{π} a b)$ .

|

Updated on:21/07/2023

Class 12 PHYSICS CURRENT ELECTRICITY

Topper's Solved these Questions

Similar Questions

HC VERMA-ELECTRIC CURRENT IN CONDUCTORS-Exercises

Home
Ask Doubt
Profile