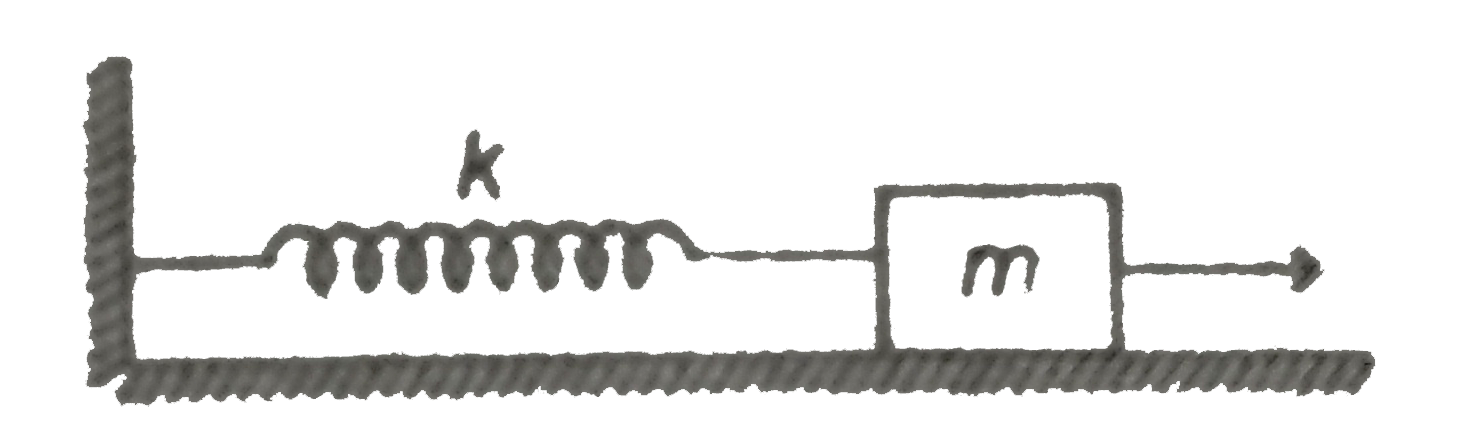
a force F acts on a block conected to sprig a force constant K as shown .The extension obtained in equillibrium x1 .If the spring constant is quadrapled ,then extension in equillibrium with same force F is X2 .Then x1:x2 is
Step by step video & image solution for a force F acts on a block conected to sprig a force constant K as shown .The extension obtained in equillibrium x1 .If the spring constant is quadrapled ,then extension in equillibrium with same force F is X2 .Then x1:x2 is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
A constant force produces maximum velocity V on the block connected to the spring of force constant K as shown in the figure. When the force constant of spring becomes 4K, the maximum velocity of the block is
 View Solution
View SolutionA block of mass m is suspended by different springs of force constant shown in figure.
 View Solution
View SolutionA spring of force constant k extends by a length X on loading . If T is the tension in the spring then the energy stored in the spring is
View SolutionTwo springs are in a series combination and are attached to a block of mass 'm' which is in equilibrium. The spring constants and extension in the springs are as shown in the figure. Then the force exerted by the spring on the block is :
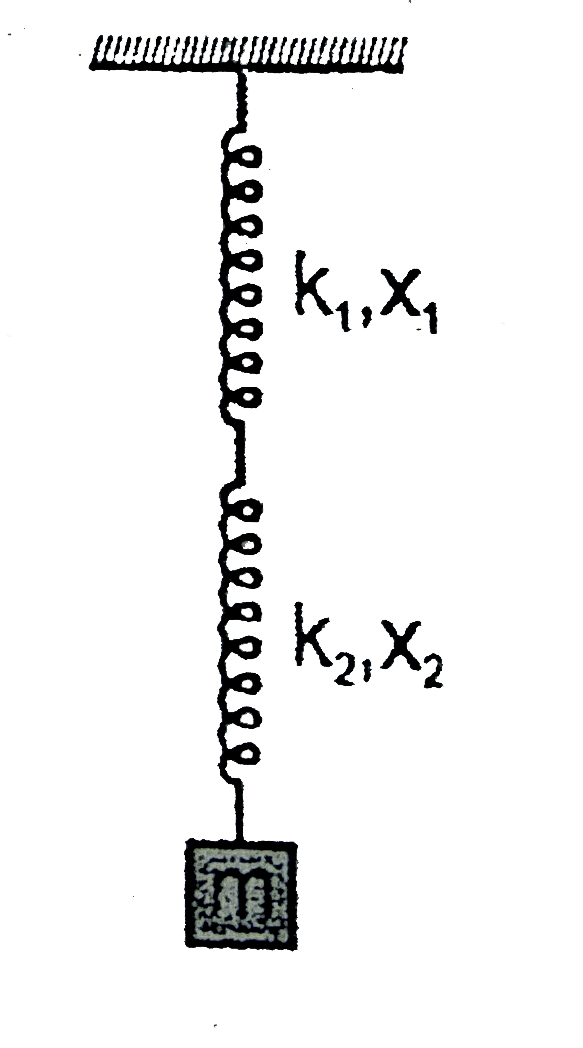 View Solution
View SolutionA spring of force constant k. is cut into lengths of ratio 1:2:3. They are connected in series and the new force constant is k'. Then they are connected in parallel and force constant is k''. Then K':K'' is
View SolutionA spring of force constant k is cut into lengths of ratio 1:2:3. They are connected in series and the new force constant is k'. Then they are connected in parallel and force constant is k'. Then k' : k" is :
View SolutionA block of mass m rests on a rough horizontal plane having coefficient of kinetic friction μk and coefficient of static friction μs. The spring is in its natural, when a constant force of magnitude P=5μkmg4 starts acting on the block. The spring force F is a function of extension x as F=kx2.( Where k is spring constant )
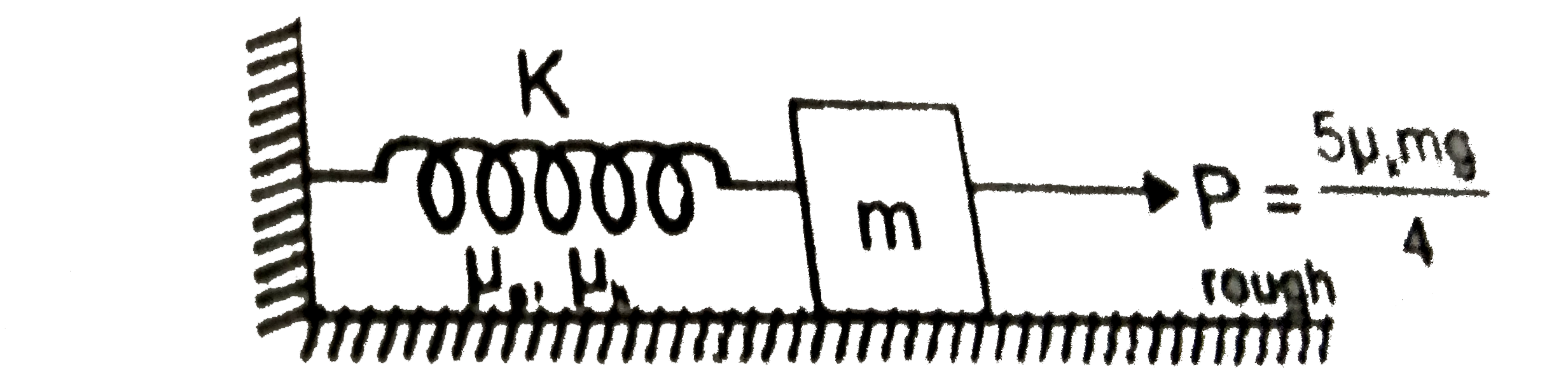
(a) Comment on the relation between μs and μk for the motion to start.
(b) Find the maximum extension in the spring ( Assume the force P is sufficient make the block move ).View SolutionA string of length L and force constant k is stretched to obtain extension l. It is further stretched to obtain extension l1. The work done in second stretching is
View SolutionInfinite springs with force constantk , 2k, 4k and 8 k .... respectively are connected in series. The effective force constant of the spring will b
View SolutionA force F=−kx3 is acting on a block moving along x-axis. Here, k is a positive constant. Work done by this force is
View Solution
