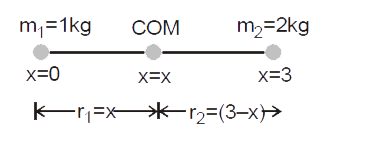Two fixed point masses of 10 kg and 20 kg are located at (0 m, 0 m) and (5 m, 0 m). Where should a third point mass of 30 kg be kept so that the net force of attraction on 30 kg mass due to point masses 10 kg and 20 kg is zero?
At 2.05 m from 10 kg mass
At 2.05 m from 20 kg mass
At 2.95 m from 10 kg mass
At 3 m from 20 kg mass
The correct Answer is:A
To solve the problem, we need to find the position of a third mass (30 kg) such that the gravitational forces exerted on it by the two fixed masses (10 kg and 20 kg) balance each other out.
1. Identify the Positions of the Masses:
- The 10 kg mass (let's call it Mass A) is located at point A (0 m, 0 m).
- The 20 kg mass (let's call it Mass B) is located at point B (5 m, 0 m).
- We need to place the 30 kg mass (let's call it Mass C) at a point C along the x-axis.
2. Define the Distance from Mass A:
- Let the distance from Mass A to Mass C be x meters. Therefore, the distance from Mass B to Mass C will be (5−x) meters.
3. Write the Gravitational Force Equations:
- The gravitational force F1 exerted by Mass A on Mass C is given by:
F1=G⋅mA⋅mCx2=G⋅10⋅30x2
- The gravitational force F2 exerted by Mass B on Mass C is given by:
F2=G⋅mB⋅mC(5−x)2=G⋅20⋅30(5−x)2
4. Set the Forces Equal for Equilibrium:
- For the net force on Mass C to be zero, we set F1=F2:
10⋅30x2=20⋅30(5−x)2
- We can cancel G and 30 from both sides:
10x2=20(5−x)2
5. Cross Multiply to Solve for x:
- Cross multiplying gives:
10(5−x)2=20x2
- Expanding both sides:
10(25−10x+x2)=20x2
250−100x+10x2=20x2
- Rearranging the equation:
0=10x2+100x−250
0=10x2−100x+250
6. Simplify the Quadratic Equation:
- Dividing the entire equation by 10:
0=x2−10x+25
7. Factor the Quadratic:
- This factors to:
(x−5)2=0
- Thus, x=5.
8. Determine the Position of Mass C:
- Since x=5 is the distance from Mass A, we can conclude that Mass C should be placed at 5 meters from Mass A, which is at the position of Mass B.
9. Check for Other Possible Positions:
- Since the forces are equal at this point, we also need to check if there are any other positions. The only other possible position would be to the left of Mass A, but that would not balance the forces as Mass A would always exert a greater force due to being closer.
Final Answer:
The third mass of 30 kg should be kept at a distance of 2.05 meters from the 10 kg mass (to the left of the 10 kg mass).
- The 10 kg mass (let's call it Mass A) is located at point A (0 m, 0 m).
- The 20 kg mass (let's call it Mass B) is located at point B (5 m, 0 m).
- We need to place the 30 kg mass (let's call it Mass C) at a point C along the x-axis.
- Let the distance from Mass A to Mass C be x meters. Therefore, the distance from Mass B to Mass C will be (5−x) meters.
- The gravitational force F1 exerted by Mass A on Mass C is given by:
F1=G⋅mA⋅mCx2=G⋅10⋅30x2
- The gravitational force F2 exerted by Mass B on Mass C is given by:
F2=G⋅mB⋅mC(5−x)2=G⋅20⋅30(5−x)2
- For the net force on Mass C to be zero, we set F1=F2:
10⋅30x2=20⋅30(5−x)2
- We can cancel G and 30 from both sides:
10x2=20(5−x)2
- Cross multiplying gives:
10(5−x)2=20x2
- Expanding both sides:
10(25−10x+x2)=20x2
250−100x+10x2=20x2
- Rearranging the equation:
0=10x2+100x−250
0=10x2−100x+250
- Dividing the entire equation by 10:
0=x2−10x+25
- This factors to:
(x−5)2=0
- Thus, x=5.
- Since x=5 is the distance from Mass A, we can conclude that Mass C should be placed at 5 meters from Mass A, which is at the position of Mass B.
- Since the forces are equal at this point, we also need to check if there are any other positions. The only other possible position would be to the left of Mass A, but that would not balance the forces as Mass A would always exert a greater force due to being closer.
The third mass of 30 kg should be kept at a distance of 2.05 meters from the 10 kg mass (to the left of the 10 kg mass).
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Centre of mass of 3 particles 10 kg, 20 kg and 30 kg is at (0, 0, 0). Where should a particle of mass 40 kg be placed so that the combination centre of mass will be at (3,3,3)
A(0,0,0)B(7.5,7.5,7.5)C(1,2,3)D(4,4,4) - Question 2 - Select One
Two particles of masses 1kg and 2kg are located at x=0 and x=3m. Find the position of their centre of mass.
A2m from 1 kgB2m from 2 kgC1m from 1 kgDnone of these - Question 3 - Select One
Centre of mass of 3 particles 10 kg, 20 kg and 30 kg is at (0, 0, 0). Where should a particle of mass 40 kg be placed so that the combined centre of mass will be at (3, 3, 3)?
A(0,0,0)B(7.5 , 7.5 , 7.5)C(1 , 2 , 3)D(4 , 4 , 4)
Two bodies A and B having masses 2 kg and 4 kg respectively are separated by 2 m. Where should a body of mass 1 kg be placed so that the gravitational force on this body due to bodies A and B is zero?
View SolutionTwo bodies A and B having masses 20kg and 40kg are separated by 10 m. at what distance from body a should another body C of mass 15 kg be placed so that net gravitational force on C is zero?
View SolutionCentre of mass of 3 particles 10 kg, 20 kg and 30 kg is at (0, 0, 0). Where should a particle of mass 40 kg be placed so that the combination centre of mass will be at (3,3,3)
View SolutionTwo bodies of masses 5 kg and 20 kg are separated by a distance of 300 m. A body of mass 1 kg is placed between the two masses in a straight line. If the forces of attraction due to the two masses on the body of 1 kg mass are the same, the distance between the 1 kg body and the 5 kg body is ____________
View SolutionTwo bodies of masses 5 kg and 20 kg are separated by a distance of 300 m. A body of mass 1 kg is placed between the two masses in a straight line. If the forces of attraction due to the two masses on the body of 1 kg mass are the same, the distance between the 1 kg body and the 5 kg body is ____________
View Solution