In the adjoining figure, sides QP and RQ of ΔPQR are produced to points S and T respectively. If ∠SPR=135∘ and ∠PQT=110∘, find ∠PRQ.
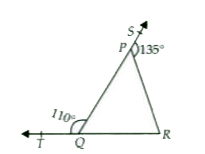
The correct Answer is:65∘
Topper's Solved these Questions
- LINES AND ANGLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - LINES AND ANGLESEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL -1)
34 Videos
- LINES AND ANGLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - LINES AND ANGLESEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL -2)
15 Videos
- LINES AND ANGLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - LINES AND ANGLESEXERCISE - NCERT SECTION( EXERCISE 6.2)
6 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - OLYMPIAD/HOTS CORNER
5 Videos
- NUMBER SYSTEMSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - NUMBER SYSTEMSEXERCISE - Olympiad/HOTS Corner
20 Videos
Similar Questions
In figure, sides QP and RQ of PQR are produced to point S and T respectively. If ∠SPR=135∘ and ∠PQT=110∘, find ∠PRQ. Figure
View SolutionIn Figure, side QP and RQ of PQR are produced to point S and T respectively. If ∠SPR=1350and ∠PQT=1100, f∈d ∠PRQ
View SolutionIn Fig. 6.39, sides QP and RQ of ΔPQR are produced to point S and T respectively. If ∠SPR=135∘ and ∠PQT=110∘, find ∠PRQ.
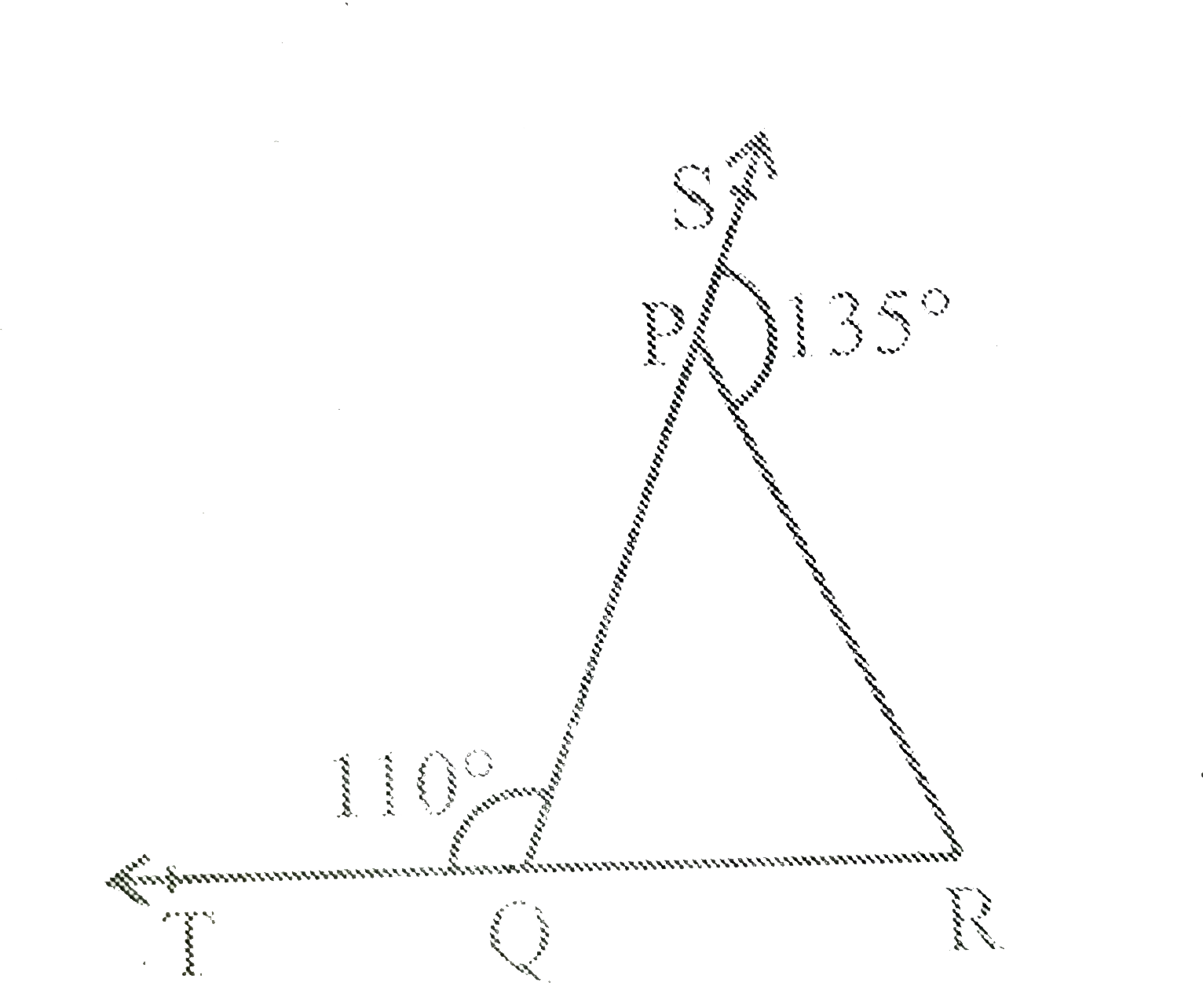
View SolutionIn Fig. 6.39, sides QP and RQ of ΔPQR are produced to point S and T respectively. If ∠SPR=135∘ and ∠PQT=110∘, find ∠PRQ.

View Solutionsides QP and RQ of PQR are produced to point S and T respectively. If ∠SPR=1350 and ∠PQT=1100, find ∠PRQ.
View SolutionIn figure, sides QP and RQ of PQR are produced to point S and T respectively. If ∠SPR=1350 and ∠PQT=1100, find ∠PRQ. Figure
View SolutionIn figure, sides QP and RQ of PQR are produced to point S and T respectively. If ∠SPR=1350 and ∠PQT=1100, find ∠PRQ. Figure
View SolutionIn Figure, side QP and RQ of PQR are produced to point S and T respectively. If ∠SPR=1350and ∠PQT=110∘, find ∠PRQ
View SolutionIn triangle PQR sides QR and RQ respectively produced to points S and T. If ∠SPR=135∘ and ∠PQT=110∘ find ∠PRQ
View SolutionIn the adjoining figure D, E and F are the mid - points of the sides BC, AC and AB respectively to triangle. Show that ∠EDF=∠A, ∠DEF=∠B and ∠DFE=∠C:

View Solution
