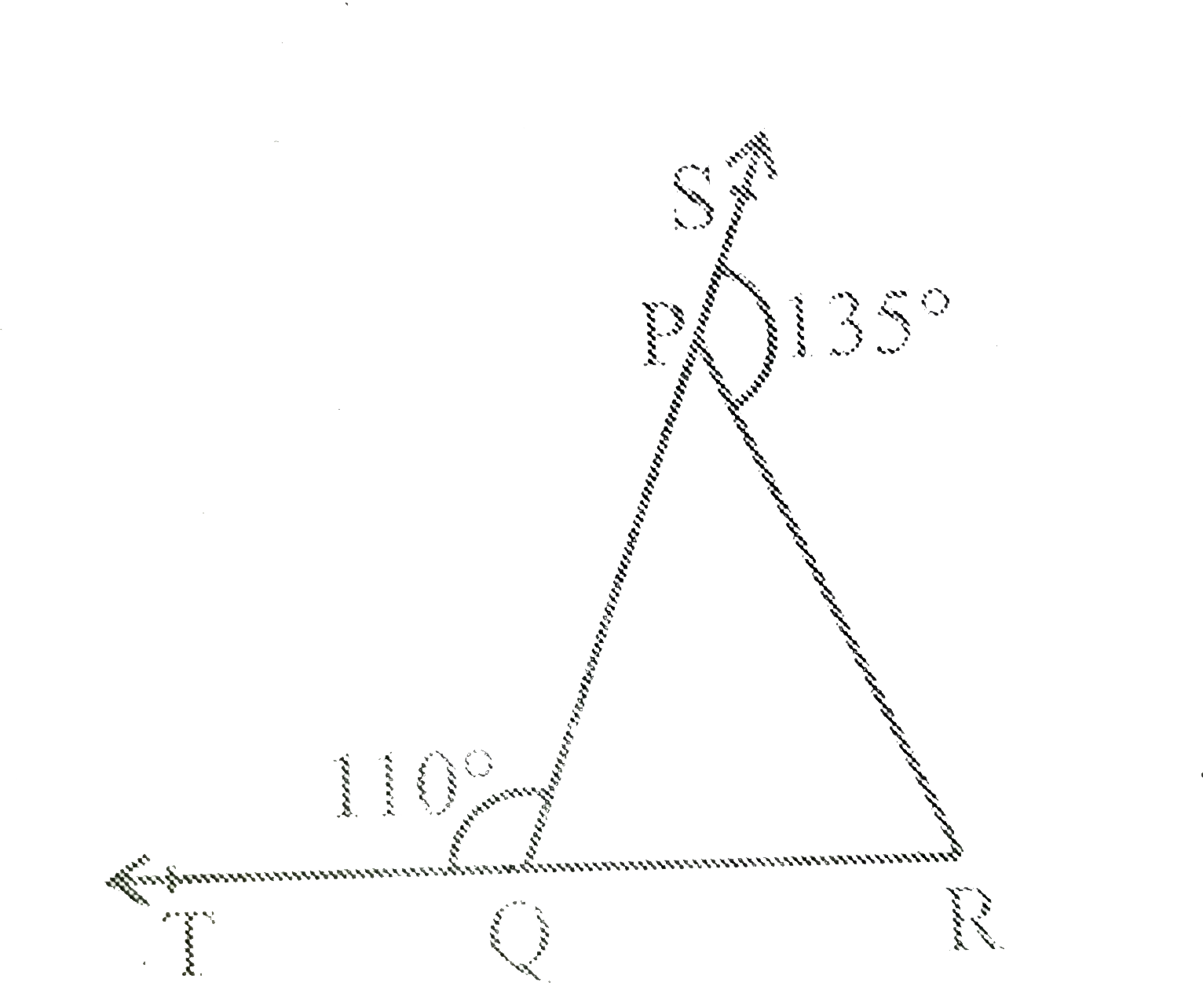
In figure, sides QP and RQ of PQR are produced to point S and T respectively. If ∠SPR=1350 and ∠PQT=1100, find ∠PRQ. Figure
To solve the problem step by step, we will follow the reasoning provided in the video transcript and use the properties of triangles and angles.
Step 1: Draw the Diagram
We start by drawing triangle PQR. Extend the sides QP to point S and RQ to point T. Label the angles as follows:
- ∠SPR=135∘
- ∠PQT=110∘
Step 2: Identify Angles in Triangle
In triangle PQR:
- Let ∠PRQ=A
- Let ∠PQR=B
- Let ∠QRP=C
According to the properties of triangles:
A+B+C=180∘(Equation 1)
Step 3: Use Exterior Angle Theorem
The exterior angle theorem states that an exterior angle of a triangle is equal to the sum of the two opposite interior angles. Thus, we can write:
1. For ∠SPR:
A+C=135∘(Equation 2)
2. For ∠PQT:
B+C=110∘(Equation 3)
Step 4: Add Equations 2 and 3
Now, we add Equation 2 and Equation 3:
(A+C)+(B+C)=135∘+110∘
This simplifies to:
A+B+2C=245∘(Equation 4)
Step 5: Subtract Equation 1 from Equation 4
Now, we subtract Equation 1 from Equation 4:
(A+B+2C)−(A+B)=245∘−180∘
This simplifies to:
2C=65∘
Dividing both sides by 2 gives:
C=32.5∘
Step 6: Find Angle C
We can now substitute C back into either Equation 2 or Equation 3 to find A or B. Let's use Equation 2:
A+32.5∘=135∘
Thus,
A=135∘−32.5∘=102.5∘
Step 7: Find Angle B
Using Equation 3:
B+32.5∘=110∘
Thus,
B=110∘−32.5∘=77.5∘
Final Answer
Thus, the angles in triangle PQR are:
- A=102.5∘
- B=77.5∘
- C=32.5∘
The angle ∠PRQ is 32.5∘.
We start by drawing triangle PQR. Extend the sides QP to point S and RQ to point T. Label the angles as follows:
- ∠SPR=135∘
- ∠PQT=110∘
In triangle PQR:
- Let ∠PRQ=A
- Let ∠PQR=B
- Let ∠QRP=C
A+B+C=180∘(Equation 1)
The exterior angle theorem states that an exterior angle of a triangle is equal to the sum of the two opposite interior angles. Thus, we can write:
1. For ∠SPR:
A+C=135∘(Equation 2)
2. For ∠PQT:
B+C=110∘(Equation 3)
Now, we add Equation 2 and Equation 3:
(A+C)+(B+C)=135∘+110∘
This simplifies to:
A+B+2C=245∘(Equation 4)
Now, we subtract Equation 1 from Equation 4:
(A+B+2C)−(A+B)=245∘−180∘
This simplifies to:
2C=65∘
Dividing both sides by 2 gives:
C=32.5∘
We can now substitute C back into either Equation 2 or Equation 3 to find A or B. Let's use Equation 2:
A+32.5∘=135∘
Thus,
A=135∘−32.5∘=102.5∘
Using Equation 3:
B+32.5∘=110∘
Thus,
B=110∘−32.5∘=77.5∘
Thus, the angles in triangle PQR are:
- A=102.5∘
- B=77.5∘
- C=32.5∘
Topper's Solved these Questions
Similar Questions
In Figure, side QP and RQ of PQR are produced to point S and T respectively. If ∠SPR=1350and ∠PQT=110∘, find ∠PRQ
View SolutionIn Fig. 6.39, sides QP and RQ of ΔPQR are produced to point S and T respectively. If ∠SPR=135∘ and ∠PQT=110∘, find ∠PRQ.
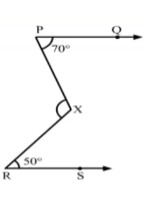
View SolutionIn Figure, TQ and TR are the bisectors of ∠Q and ∠R respectively. If ∠QPR=800and ∠PRT=300, determine ∠TQR and ∠QTR
View SolutionIn figure, PQ=PR . Show that PS>PQ.
View SolutionIn Figure , find out ∠PXR, if PQ ∣∣ RS.
View SolutionIn Figure, PQ>PR.QS and RS are the bisectors of ∠Q and ∠R respectively. Prove that SQ>SR.
View SolutionIf Figure, if QT⊥PR,∠TQR=400 AND ∠SPR=300, find x and y. Figure
View SolutionIn figure, if lines PQ and RS intersect at a point T such that PRT=400,∠RPT=950 and ∠TSQ=750, find ∠SQT. Figure
View SolutionIn Fig. , the side QR of △ PQR is produced to a point S. If the bisectors of ∠PQR and ∠PRS meet at point T, then prove that ∠QTR=12∠QPR.

View SolutionIn Fig. 6.31, if PQ∣∣ST,∠PQR=110∘ and∠RST=130∘, find ∠QRS.
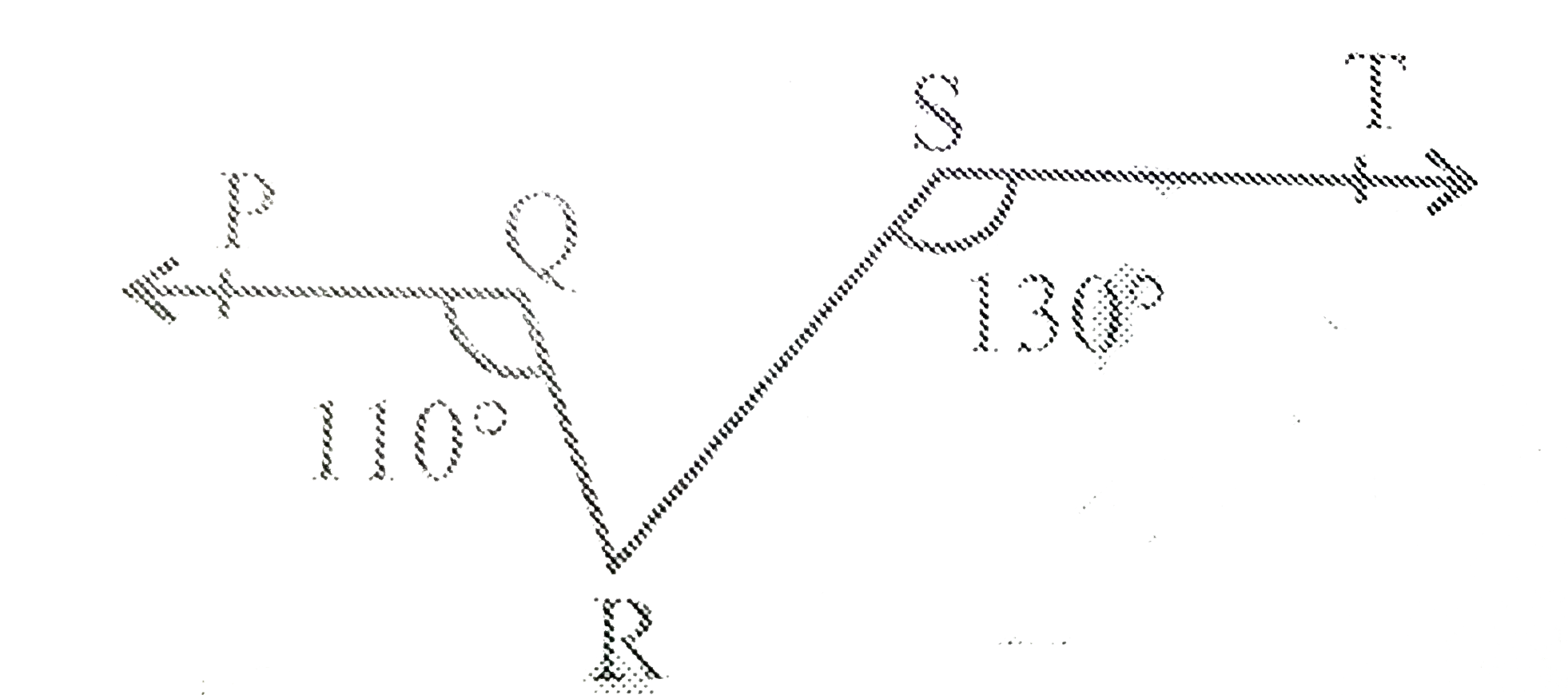
View Solution