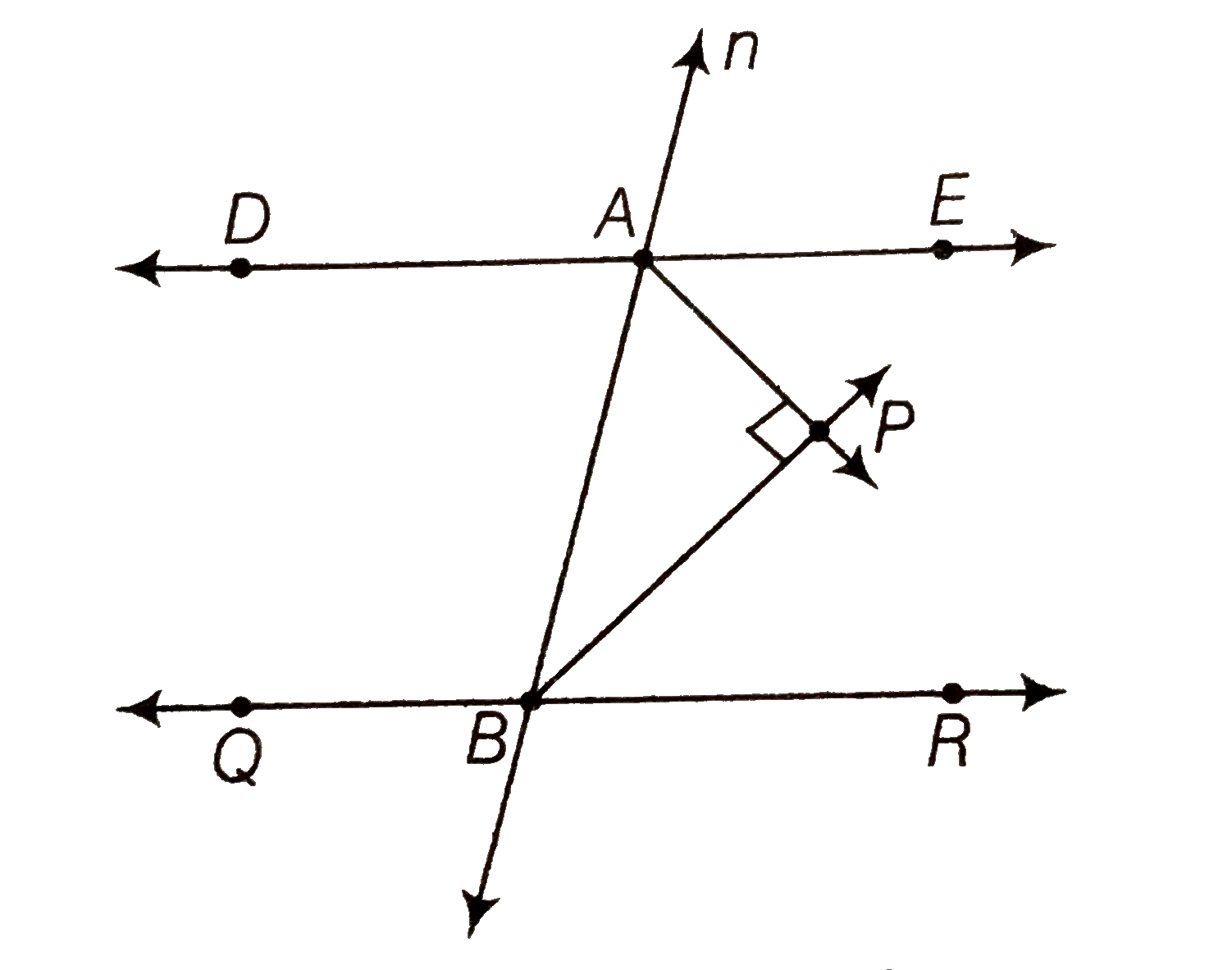
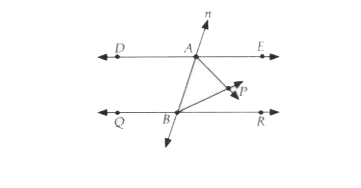
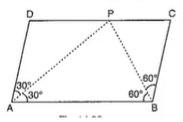
In figure DE||QR and AP and BP are bisectors of ∠EAB and ∠RBA respectively. Find ∠APB.
A
90∘
B
45∘
C
100∘
D
80∘
Text Solution
The correct Answer is:A
|
Topper's Solved these Questions
- LINES AND ANGLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - LINES AND ANGLESEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL -2 COMPREHENSION TYPE)
9 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - OLYMPIAD/HOTS CORNER
5 Videos
- NUMBER SYSTEMSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - NUMBER SYSTEMSEXERCISE - Olympiad/HOTS Corner
20 Videos
Similar Questions
Knowledge Check
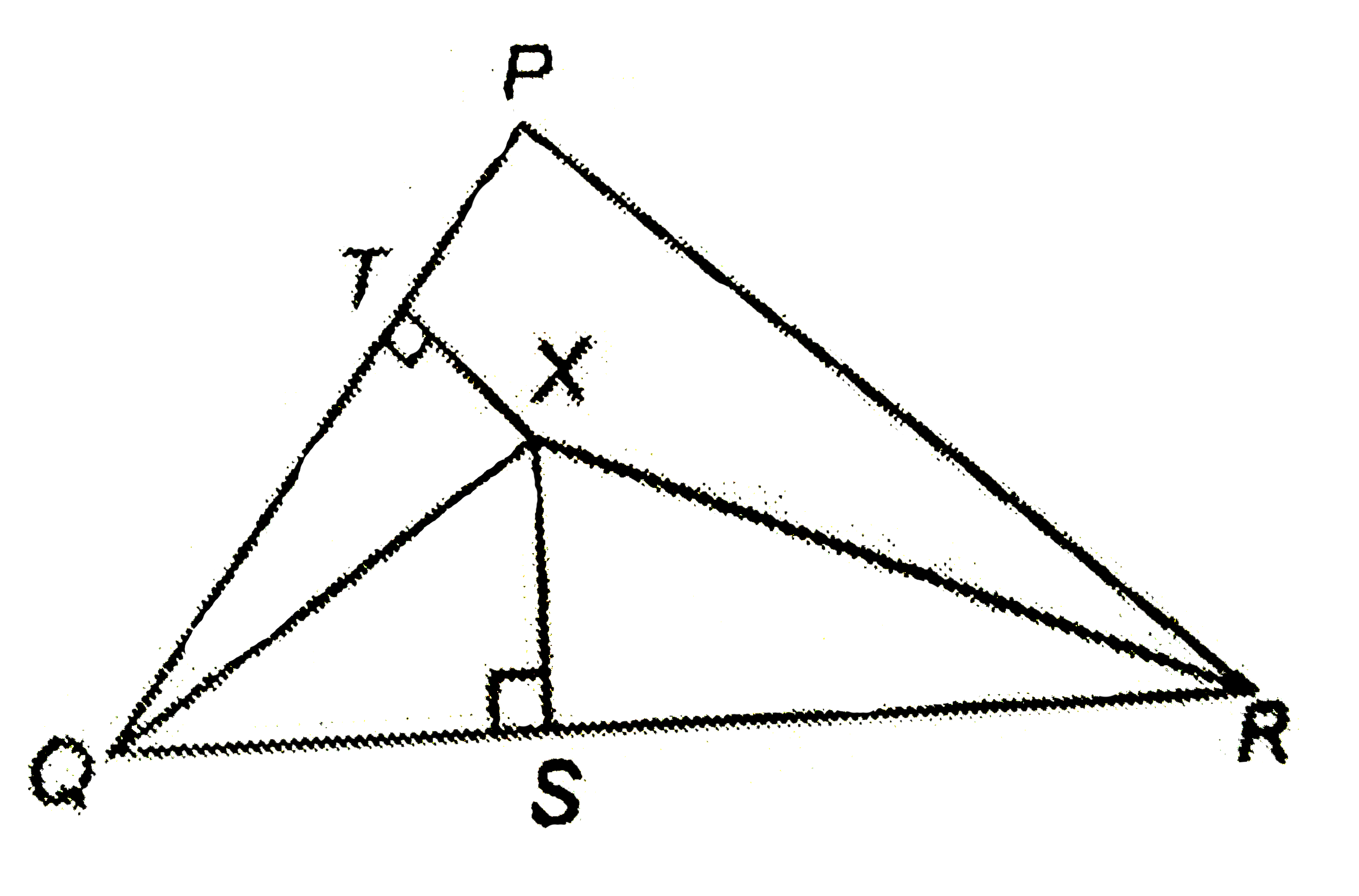
In the adjoining figure, QX and RX are the bisectors of the angles Q and R respectively of the angles Q and R respectively of the triangle PQR. If XS⊥PQ. Prove that :
(i) ΔXTQ≅ΔXSQ
(ii) PX bisects angle P.
 ASASBRHSCAASDASA
ASASBRHSCAASDASAIn figure , if AP = 10 cm, then BP
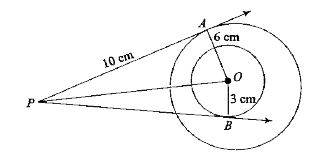 A√91 cmB√127cmC√119 cmD√109 cm
A√91 cmB√127cmC√119 cmD√109 cm
In the given figure, DE || QR and AP and BP are bisectors of ∠EABand∠RBA, respectively. The value of ∠APB=
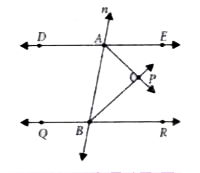
View SolutionIn the adjoining figure, OX and RX are the bisectors of the angle Q and R respectively of the triangle PQR. If XS⊥QR and XT⊥PQ.
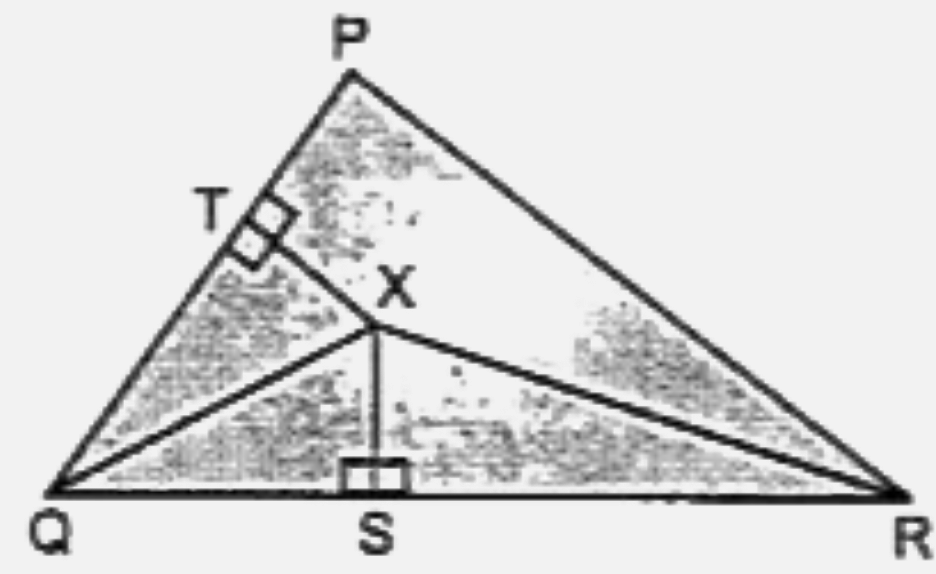
Prove that :
ΔXTQ≅ΔXSQ
View SolutionIn the adjoining figure, OX and RX are the bisectors of the angle Q and R respectively of the triangle PQR. If XS⊥QR and XT⊥PQ.
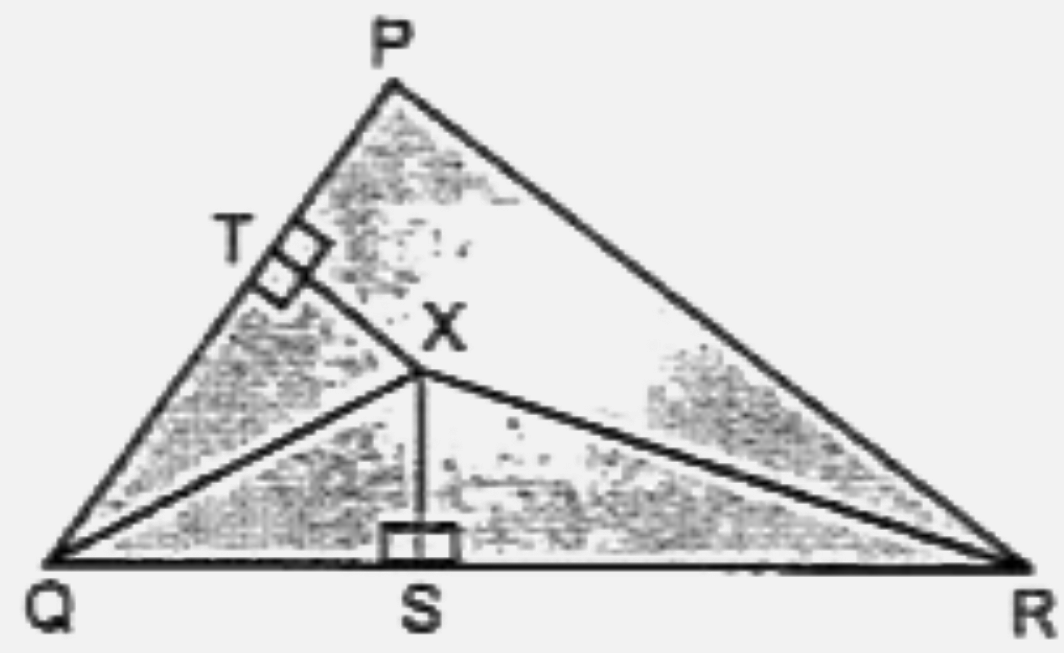
Prove that :
PX bisects angle P.
View SolutionIn the following figure, find AP and BP.
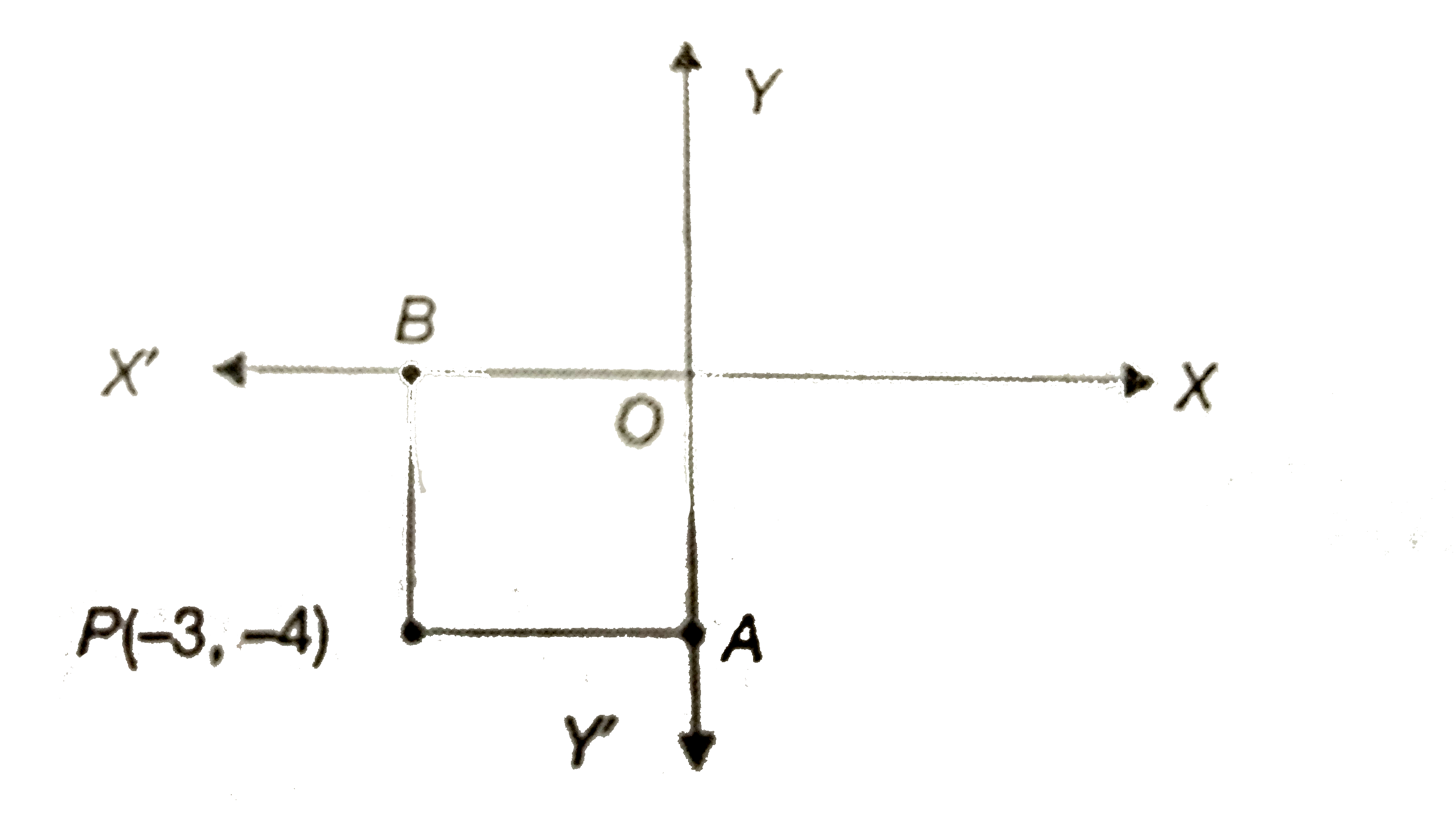
View SolutionIn Figure, ABCD is a parallelogram and ∠DAB=600. If the bisectors AP and BP of angles A and B respectively, meet at P on CD, prove that P is the mid-point of CD.
View SolutionIn figure DE || OQ and DF || OR. Show that EF||QR.
View Solution