If the charge on a capacitor is increased by 2 C, the energy stored in it increases by 44%. The original charge on the capacitor is (in C)
10
29
30
40
To solve the problem, we need to find the original charge Q on a capacitor given that increasing the charge by 2 C results in a 44% increase in the energy stored in the capacitor.
1. Understanding the Energy Stored in a Capacitor:
The energy U stored in a capacitor is given by the formula:
U=Q22C
where Q is the charge on the capacitor and C is the capacitance.
2. Initial Energy:
Let the initial charge on the capacitor be Q. The initial energy stored in the capacitor is:
U=Q22C
3. Charge After Increase:
When the charge is increased by 2 C, the new charge becomes:
Q′=Q+2
4. New Energy After Charge Increase:
The new energy U′ stored in the capacitor after the charge increase is:
U′=(Q+2)22C
5. Energy Increase Relation:
According to the problem, the energy increases by 44%. Therefore, we can express this as:
U′=U+0.44U=1.44U
6. Setting Up the Equation:
We can now set up the equation using the expressions for U and U′:
(Q+2)22C=1.44(Q22C)
7. Canceling Common Terms:
Since 2C appears in both sides of the equation, we can cancel it:
(Q+2)2=1.44Q2
8. Expanding the Left Side:
Expanding the left side gives:
Q2+4Q+4=1.44Q2
9. Rearranging the Equation:
Rearranging the equation leads to:
0=1.44Q2−Q2−4Q−4
Simplifying this gives:
0=0.44Q2−4Q−4
10. Multiplying Through by 100:
To eliminate the decimal, we can multiply the entire equation by 100:
0=44Q2−400Q−400
11. Using the Quadratic Formula:
We can apply the quadratic formula Q=−b±√b2−4ac2a where a=44,b=−400,c=−400:
Q=400±√(−400)2−4⋅44⋅(−400)2⋅44
Q=400±√160000+7040088
Q=400±√23040088
Q=400±48088
12. Calculating the Values:
This gives us two potential solutions:
Q1=88088=10andQ2=−8088 (not physically meaningful)
13. Final Answer:
Thus, the original charge on the capacitor is:
Q=10 C
The energy U stored in a capacitor is given by the formula:
U=Q22C
where Q is the charge on the capacitor and C is the capacitance.
Let the initial charge on the capacitor be Q. The initial energy stored in the capacitor is:
U=Q22C
When the charge is increased by 2 C, the new charge becomes:
Q′=Q+2
The new energy U′ stored in the capacitor after the charge increase is:
U′=(Q+2)22C
According to the problem, the energy increases by 44%. Therefore, we can express this as:
U′=U+0.44U=1.44U
We can now set up the equation using the expressions for U and U′:
(Q+2)22C=1.44(Q22C)
Since 2C appears in both sides of the equation, we can cancel it:
(Q+2)2=1.44Q2
Expanding the left side gives:
Q2+4Q+4=1.44Q2
Rearranging the equation leads to:
0=1.44Q2−Q2−4Q−4
Simplifying this gives:
0=0.44Q2−4Q−4
To eliminate the decimal, we can multiply the entire equation by 100:
0=44Q2−400Q−400
We can apply the quadratic formula Q=−b±√b2−4ac2a where a=44,b=−400,c=−400:
Q=400±√(−400)2−4⋅44⋅(−400)2⋅44
Q=400±√160000+7040088
Q=400±√23040088
Q=400±48088
This gives us two potential solutions:
Q1=88088=10andQ2=−8088 (not physically meaningful)
Thus, the original charge on the capacitor is:
Q=10 C
Topper's Solved these Questions
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2022EXERCISE - PHYSICS (SECTION -A)20videos
JEE MAINS 2022
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2022EXERCISE - PHYSICS (SECTION -B)10videos
JEE MAINS 2022
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2022EXERCISE - PHYSICS60videos
JEE MAINS 2022
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics (Section B )10videos
JEE MAINS 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2023 JAN ACTUAL PAPEREXERCISE - Question360videos
JEE MAINS 2023 JAN ACTUAL PAPER
Similar Questions
Knowledge Check
- Question 1 - Select One
If the charge on a capacitor is increased by 2C, then the energy stored in it increases by 21 %. The original charge on the capacitor is
A10 CB20 CC30 CD40 C - Question 2 - Select One
The charge on a 48 μF capacitor is increased from 0.1 C to 0.5 C. the enerrgy stored in the capacitor increases by
A250 JB2500 JC2.5×106JD2.42×10−6J - Question 3 - Select One
If a 4μF capacitor is charged to 1 kV, then energy stored in the capacitor is
A1 JB4 JC6 JD2 J
Find the charge stored in the capacitor.
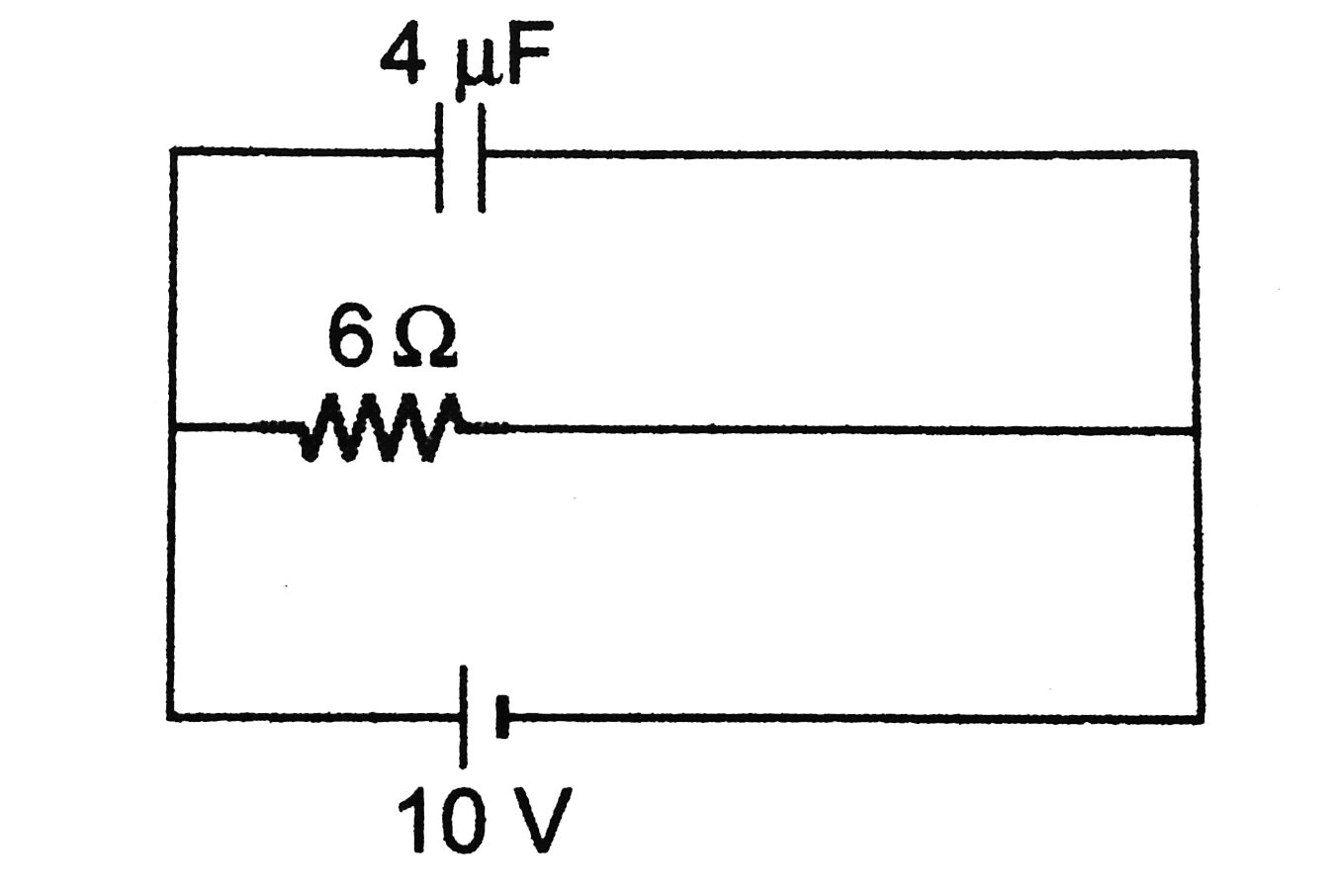 View Solution
View SolutionFind the charge stored in the capacitor.
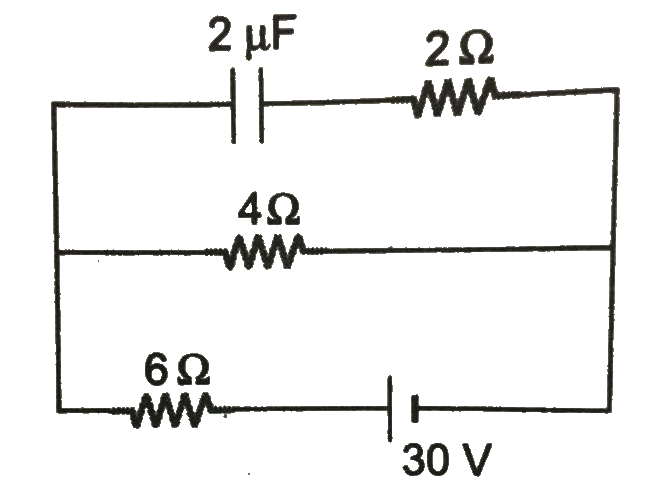 View Solution
View SolutionThe capacitance of a charged capacitor is C and the energy stored in it is U. What is the value of charge on the capacitor?
View SolutionIf a 4μF capacitor is charged to 1KV, then energy stored is conductor is
View SolutionIn a charged capacitor, the energy is stored in
View Solution