A stream of a positively charged particles and velocity is deflected by an electric field .Theelectric field exists in a region of 10 cm along x direction. Due to the electric field, the deflection of the charge particles in the y direction is _____mm.
The correct Answer is:B
To solve the problem, we need to find the deflection of positively charged particles in the y-direction due to an electric field. Let's break down the solution step by step.
Step 1: Identify the Given Values
- Charge-to-mass ratio: qm=2×1011C/kg
- Velocity of particles: →v=3×107^im/s
- Electric field: →E=1.8^jkV/m=1.8×103^jV/m
- Length of the region with electric field: x=10cm=0.1m
Step 2: Calculate the Time of Flight
The time t taken to travel through the electric field can be calculated using the formula:
t=xvx
Substituting the values:
t=0.1m3×107m/s=13×108s
Step 3: Calculate the Acceleration in the y-direction
The acceleration ay experienced by the charged particles due to the electric field can be calculated using:
ay=qEm
Using the charge-to-mass ratio:
ay=qmE=(2×1011C/kg)(1.8×103V/m)
Calculating this gives:
ay=3.6×1014m/s2
Step 4: Calculate the Deflection in the y-direction
Using the kinematic equation for displacement in the y-direction:
y=uit+12ayt2
Since the initial velocity in the y-direction ui=0:
y=12ayt2
Substituting the values of ay and t:
y=12(3.6×1014m/s2)(13×108s)2
Calculating t2:
t2=(13×108)2=19×1016s2
Now substituting this back into the equation for y:
y=12(3.6×1014)(19×1016)
y=3.6×101418×1016=2×10−2m=0.02m=20mm
Final Answer
The deflection of the charged particles in the y-direction is 20 mm.
- Charge-to-mass ratio: qm=2×1011C/kg
- Velocity of particles: →v=3×107^im/s
- Electric field: →E=1.8^jkV/m=1.8×103^jV/m
- Length of the region with electric field: x=10cm=0.1m
The time t taken to travel through the electric field can be calculated using the formula:
t=xvx
Substituting the values:
t=0.1m3×107m/s=13×108s
The acceleration ay experienced by the charged particles due to the electric field can be calculated using:
ay=qEm
Using the charge-to-mass ratio:
ay=qmE=(2×1011C/kg)(1.8×103V/m)
Calculating this gives:
ay=3.6×1014m/s2
Using the kinematic equation for displacement in the y-direction:
y=uit+12ayt2
Since the initial velocity in the y-direction ui=0:
y=12ayt2
Substituting the values of ay and t:
y=12(3.6×1014m/s2)(13×108s)2
Calculating t2:
t2=(13×108)2=19×1016s2
Now substituting this back into the equation for y:
y=12(3.6×1014)(19×1016)
y=3.6×101418×1016=2×10−2m=0.02m=20mm
The deflection of the charged particles in the y-direction is 20 mm.
Topper's Solved these Questions
Similar Questions
A mass of 10kg is suspended vertically by a rope of length 5 m from the roof. A force of 30 N is applied at the middle point of rope in horizontal direction .The angle made by upper half of the rope with vertical is θ=tan−1(x×10−1). The value of x is _______
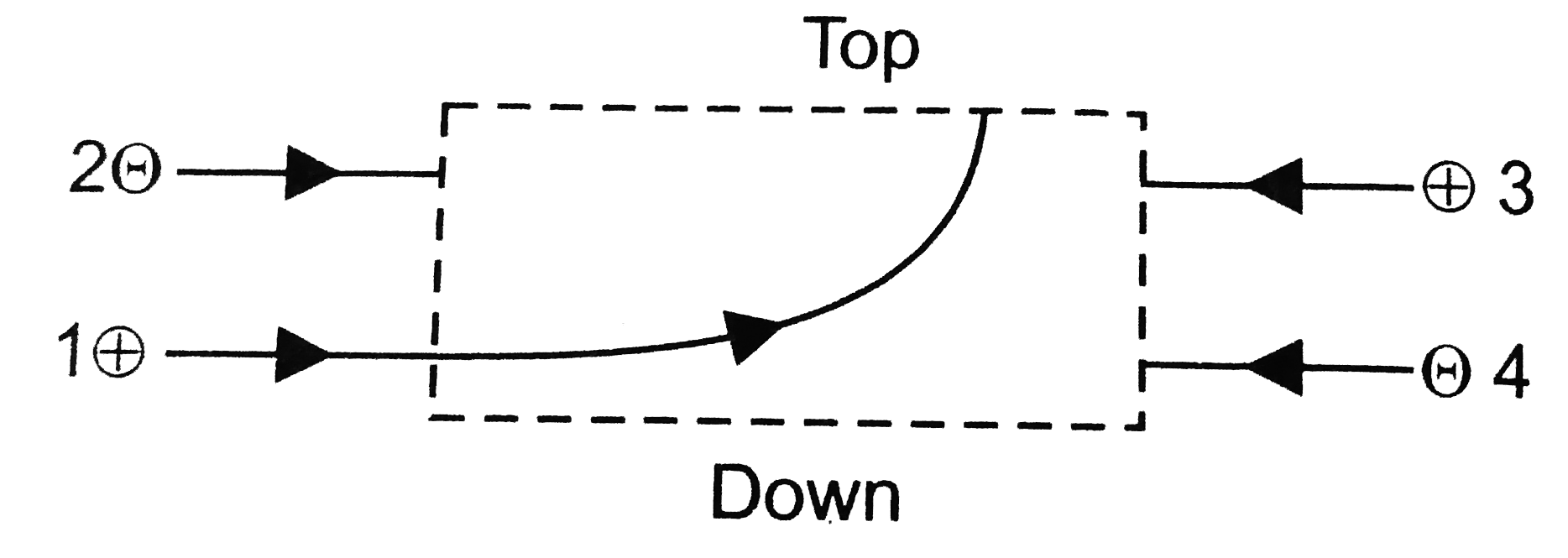
(Given , g =10m/s2)02:13View SolutionThe figure shows the path of a positively charged particle 1 through a rectangualr region of unifrom electric field as shown in the figure. What is the direction of electric field and the direction of deflection of particles 2,3 and 4 ?
 06:06View Solution
06:06View Solutionएक इलेक्ट्रान धारामें इलेक्ट्रान को वेना 2.0×107 मी/से, 1.6×103 वोल्ट/मी के वैद्युत क्षेत्र के लंबवत दिशा में 10 सेमी चलने में 3.4 मिमी विक्षेपित को जाता है । इलेक्ट्रान का विशिष्ट आवेश (e /m ) ज्ञात कीजिए ।
03:40View SolutionAn electron having mass 9.1×10−11 kg, charge 1.6×10−19 C and moving with the velocity of 106 m/s enters a region where magnetic field exists. If it describes a circle of radius 0.2 m then the intensity of magnetic field must be _________ ×10−5T
03:49View SolutionA charged particle of mass 3 xx 10^(-6) kg is held stationary in spacing in an electric field of strength 10^(6) NC^(-1) directed vertically downwards. The charge on the particle is ( g = 10 ms^(-2))
07:21View SolutionA proton is moving with a velocity of 5 xx 10^(5) m//s along the Y-direction. It is acted upon by an electric field of intensity 10^(5) V/m along the X-direction and a magnetic field of 1 Wb//m^(2) along the Z-direction. Then the Lorentz force on the particle is :
01:47View SolutionThe electric field due to a proton of charge 1.6×10−19C at a distance 1 A (10−10m) from it is
02:53View SolutionA charged particle with a velocity 2 xx 10^(3) m s^(-1) passes undeflected through electric field and magnetic fields in the mutually perpendicular directions. The magnetic field is 1.5 T. The magnitude of electric field will be
03:41View SolutionIf particle of charge 10^(-12) coulomb moving along the hatx - direction with a velocity 10^(5)m//s experiences a force of 10^(-10) newton in haty - direction due to magnetic field, then the minimum magnetic field is
04:03View SolutionTwo charged particles are attached to an x axis: Particle 1 of charge -4.00 xx 10^(-7) C is at position x = -5.00 cm and particle 2 of charge +4.00 xx 10^(-7)C is at position x = 10.cm. Midway between the particles, what is their net electric field in unit-vector notation ?
02:28View Solutionविद्युत क्षेत्र 3×10−10Vm−1 में अनुगमन वेग 7.5×10−4ms−1 वाले आवेशित कण की चलायमानता (Mobility) है
02:16View Solution32×10−19 कूलॉम आवेश वाला एक α- कण 500 न्यूटन/एम्पियर-मी तीव्रता वाले चुाजकीय क्षेत्र में 100 मी/से के वेग से क्षेत्र की दिशा में प्रवेश करता है। कण पर लगने वाला बल है
02:33View SolutionThe figure shows the path of a positively charged particle 1 through a rectangualr region of unifrom electric field as shown in the figure. What is the direction of electric field and the direction of deflection of particles 2,3 and 4 ?
 03:23View Solution
03:23View SolutionA particle of mass 2 xx 10^(-3) kg, charge 4 xx 10^(-3)C enters in an electric field of 5 V//m , then its kinetic energy after 10 s is
04:12View SolutionIn a region, an electric field →E=5×103ˆjNC−1 and a magnetic field of →B=0.1ˆKT are applied. A beam of charged particles are projected along X-direction. Find the velocity of charged particles which move an deflected in this crossed fields.
02:04View SolutionA charged particle with a velocity 2×103ms−1 passes undeflected through electric field and magnetic fields in mutually perpendicular directions. The magnetic field is 1.5 T. The magnitude of electric field will be
02:19View Solution

