As per the given figure,a small ball P slides down the quadrant of a circle and hits the other ball Q of equal mass which is initially at rest.Neglecting the effect of friction and assume the collision to be elastic, the velocity of ball Q after collision will be (g=10ms2)
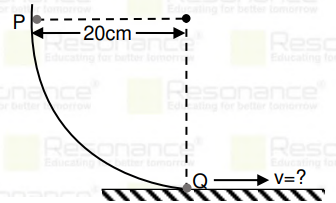
4ms
0
0.25ms
2ms
The correct Answer is:D
Step by step video, text & image solution for As per the given figure,a small ball P slides down the quadrant of a circle and hits the other ball Q of equal mass which is initially at rest.Neglecting the effect of friction and assume the collision to be elastic, the velocity of ball Q after collision will be (g=10m/s^(2)) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
A ball P is moving with a certain velocity , collides head-on with another ball Q of same mass is rest. The coefficient of restitution is 1/4, then ratio of velocity of P and Q just after the collision is
View SolutionA glass ball collides with an identical ball at rest with v0=2 m/sec. If the coefficient of restitution of collision is e = 0.5, find the velocities of the glass balls just after the collision.
View Solution
Knowledge Check
- Question 1 - Select One
A ball of mass 10 kg is moving with a velocity of 10 m / s . It strikes another ball of mass 5 kg which is moving in the same direction with a velocity of 4 m / s . If the collision is elastic, their velocities after the collision will be, respectively
A6m/s, 12m/sB12m/s, 6m/sC12 m/s, 10 m/sD12m/s, 25m/s - Question 2 - Select One
A ball of mass m moving with velocity v collides head-on which the second ball of mass m at rest. I the coefficient of restitution is e and velocity of first ball after collision is v1 and velocity of second ball after collision is v2 then
Av1=(1−e)u2,v2=(1+e)u2Bv1=(1+e)u2,v2=(1−e)u2Cv1=u2,v2=−u2Dv1=(1+e)u,v2=(1−e)u - Question 3 - Select One
The first ball of mass m moving with the velocity υ collides head on with the second ball of mass m at rest. If the coefficient of restitution is e, then the ratio of the velocities of the first and the second ball after the collision is
A1−e1+eB1+e1−eC1+e2D1−e2
A ball of mass m makes an elastic collision with another identical ball at rest. Show that if the collision is oblique, the bodies go at right angles to each other after collision.
View SolutionA ball of 0.1 kg makes an elastic head on collision with a ball of unknown mass that is initially at rest. If the 0.1kg ball rebounds at one third of its original speed, what is the mass of the other ball?
View SolutionA clay ball of mass m and speed v strikes another metal ball. of same mass m, which is at rest. They stick together after collision. The kinetic energy of the system after collision is –
View SolutionA ball of mass m moving with a constant velocity strikes against a ball of same mass at rest. If e= coefficient of restitution, then what will the the ratio of the velocities of the two balls after collision?
View SolutionA ball is moving with velocity 2m/s towards a heavy wall moving towards the ball with speed 1m/s as shown in figure. Assuming collision to be elastic, find the velocity of ball immediately after the collision.
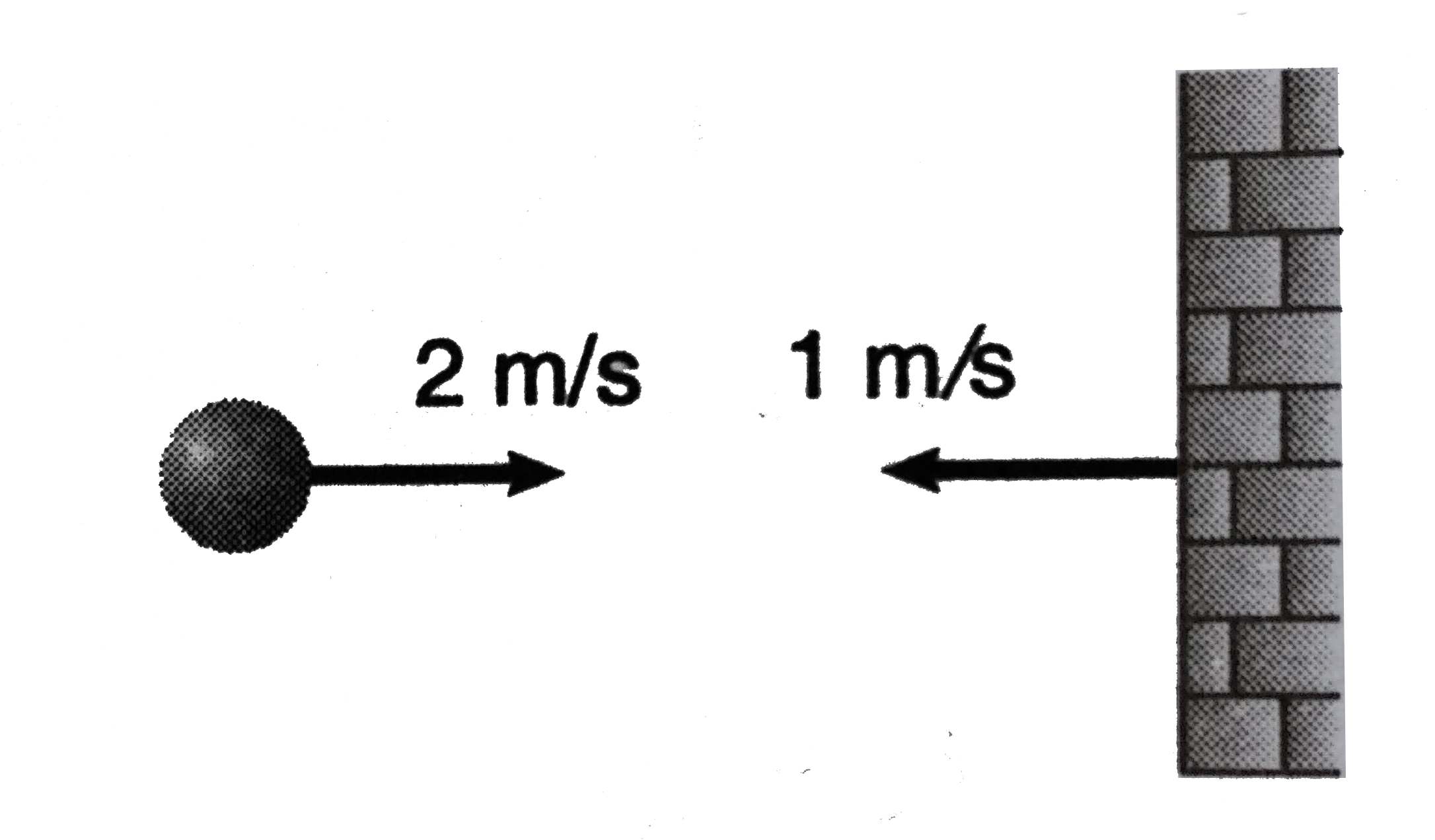 View Solution
View Solution

