A Point charge $q_{1} = 4 q_{0}$ is placed at origin.Another point charge $q_{2} = - q_{0}$ is placed at $x = 12 c m$ . Charge of proton is $q_{o} 0$ .The proton is placed on $x$ axis so that the electrostatic force on the proton is zero.In this situation,the position of the proton from the origin is ________ $c m$

Video Solution

Text Solution

Generated By DoubtnutGPT

To solve the problem, we need to find the position of a proton placed on the x-axis such that the net electrostatic force acting on it due to two other point charges is zero.
1. Identify the Charges and Their Positions:
- Charge $q_{1} = 4 q_{0}$ is located at the origin (0 cm).
- Charge $q_{2} = - q_{0}$ is located at $x = 12$ cm.
- The charge of the proton is $q_{0}$ .
2. Let the Position of the Proton be $x$ cm:
- We need to find $x$ such that the net force on the proton is zero.
3. Write the Expression for the Forces:
- The force on the proton due to charge $q_{1}$ (at the origin) is given by Coulomb's law:
$F_{1} = k \frac{| q_{1} \cdot q_{0} |}{x^{2}} = k \frac{4 q_{0}^{2}}{x^{2}}$
- The force on the proton due to charge $q_{2}$ (at $x = 12$ cm) is:
$F_{2} = k \frac{| q_{2} \cdot q_{0} |}{(x - 12)^{2}} = k \frac{q_{0}^{2}}{(x - 12)^{2}}$
4. Set the Forces Equal to Each Other:
- For the net force on the proton to be zero, the magnitudes of the forces must be equal:
$F_{1} = F_{2}$
$k \frac{4 q_{0}^{2}}{x^{2}} = k \frac{q_{0}^{2}}{(x - 12)^{2}}$
5. Cancel Common Terms:
- We can cancel $k$ and $q_{0}^{2}$ from both sides:
$\frac{4}{x^{2}} = \frac{1}{(x - 12)^{2}}$
6. Cross Multiply:
- Cross multiplying gives:
$4 (x - 12)^{2} = x^{2}$
7. Expand and Rearrange:
- Expand the left side:
$4 (x^{2} - 24 x + 144) = x^{2}$
$4 x^{2} - 96 x + 576 = x^{2}$
- Rearranging gives:
$3 x^{2} - 96 x + 576 = 0$
8. Divide the Equation by 3:
- Simplifying the equation:
$x^{2} - 32 x + 192 = 0$
9. Use the Quadratic Formula:
- The quadratic formula is $x = \frac{- b \pm \sqrt{b^{2} - 4 a c}}{2 a}$ :
- Here, $a = 1, b = - 32, c = 192$ :
$x = \frac{32 \pm \sqrt{(- 32)^{2} - 4 \cdot 1 \cdot 192}}{2 \cdot 1}$
$x = \frac{32 \pm \sqrt{1024 - 768}}{2}$
$x = \frac{32 \pm \sqrt{256}}{2}$
$x = \frac{32 \pm 16}{2}$
10. Calculate the Two Possible Values for $x$ :
- $x = \frac{48}{2} = 24$ cm
- $x = \frac{16}{2} = 8$ cm
11. Determine the Valid Position:
- The proton must be between the two charges to experience a net force of zero. Since $q_{1}$ is at 0 cm and $q_{2}$ is at 12 cm, the valid position for the proton is:
$x = 8 cm$
Final Answer:
The position of the proton from the origin is 8 cm.

Two point charges are fixed on the Xaxis , $q_{1} = 12.0 m C$ is located at the origin and $q_{2} = - 3.0 m C$ is located at point A, with $x_{2} = 8.0 c m$ . Where should a third charge $q_{3}$ , be placed on the X-axis so that the total, electrostatic force acting on it is zero?
Aat $x_{3} = 16 c m$
Bat $x_{3} = 12 c m$
Cat $x_{3} = 24 c m$
Dat $x_{3} = 6 c m$
A charge +q is located at the origin, while an identical charge is located on the x axis at x = +0.50 m. A third charge of +2 q is located on the x axis at such a place that the net electrostatic force on the charge at the origin doubles, its direction remaining unchanged. Where should the third charge be located ?
A $+ 0.50 m$
B $+ 0.71 m$
C $- 0.50 m$
D $- 0.71 m$
A point charge $q_{1}$ is placed inside cavity 1 and another point charge $q_{2}$ is inside cavity 2. A point charge q is placed outside the conductor. For the situation described above, answer the following questions.

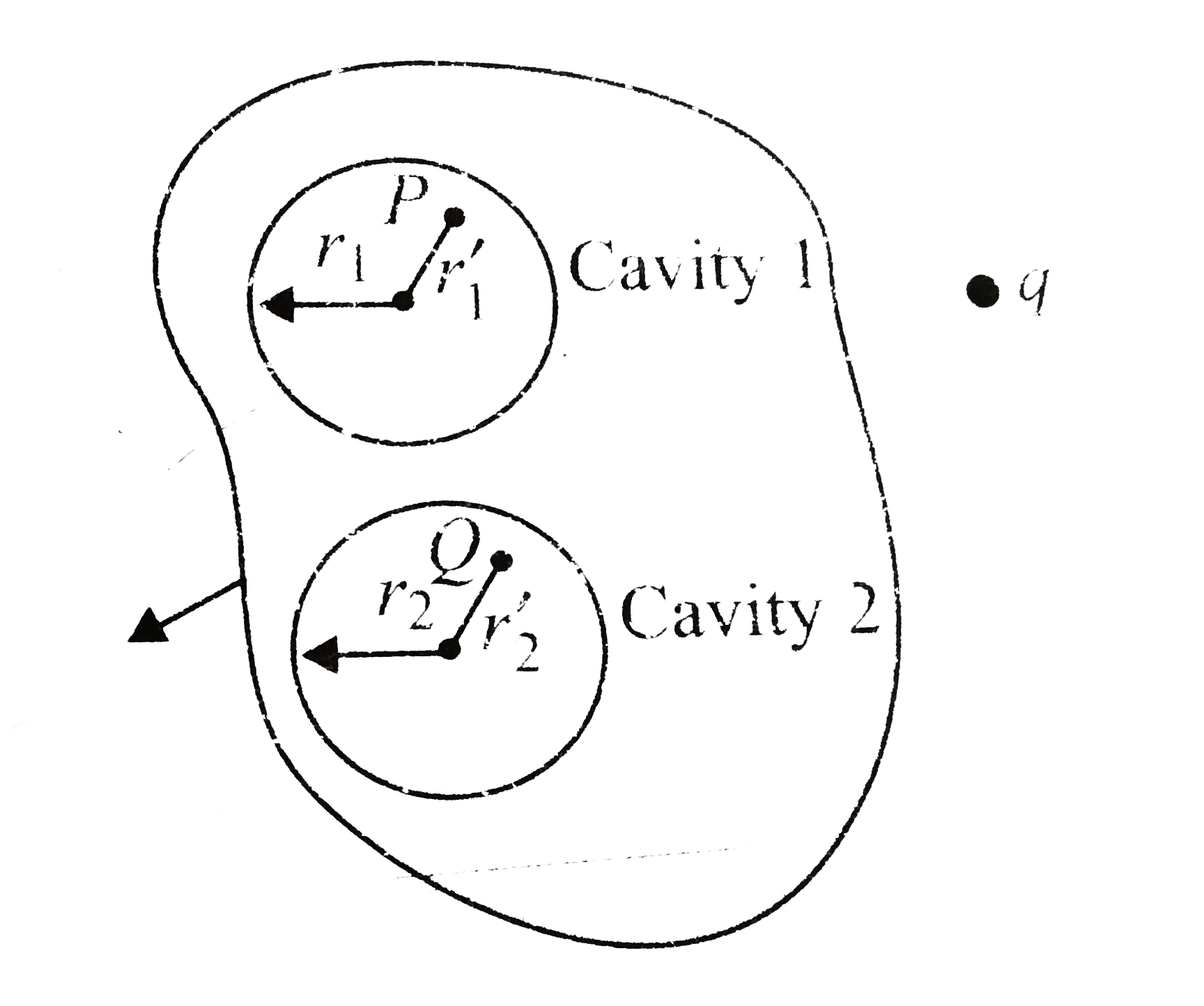
The charge on outer surface of the conductor would be
A $Q + q_{1} + q_{2}$ and nonuniformly distributed
B $Q + q_{1} + q_{2}$ and its uniform or nonuniform distribution depends upon locations of $q_{1} and q_{2}$
C $Q + q_{1} + q_{2}$ and would be distributed uniformly
D $Q + q_{1} + q_{2}$ and the distribution depends upon the locations of $q_{1}, q_{2}, and q$ .