Consider the 6×6 square in the figure. Let A1,A2,...,A49 be the points of intersections (dots in the picture) in some order. We say that AiandAj are friends if they are adjacent along a row or along a column. Assume that each point Ai has an equal chance of being chosen

Two distinct points are chosen randomly out of the points A1,A2,...A49 . Let p be the probability that they are friends. Then the value of 7p is
Step by step video solution for Consider the 6 xx 6 square in the figure. Let A_1,A_2, . . .,A_(49) be the points of intersections (dots in the picture) in some order. We say that A_i and A_j are friends if they are adjacent along a row or along a column. Assume that each point A_i has an equal chance of being chosen Two distinct points are chosen randomly out of the points A_1, A_2, . . . A_(49) . Let p be the probability that they are friends. Then the value of 7 p is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Consider the 6×6 square in the figure. Let A1,A2,...,A49 be the points of intersections (dots in the picture) in some order. We say that AiandAj are friends if they are adjacent along a row or along a column. Assume that each point Ai has an equal chance of being chosen

Let pi be the probability that a randomly chosen point has i many friends, i=0,1,2,3,4 . Let X be a random variable such that for i=0,1,2,3,4, the probability P(X=i)=pi. Then the value of 7E(X) is
View SolutionLet A1,A2,A3,...,A8 be the vertices of a regular octagon that lie on a circle of radius 2. Let P be a point on the circle and let PAi denote the distance between the points PandAi for i=1,2,...,8.If P varies over the circle, then the maximum value of the product PA1,PA2...PA8, is
View Solution
Knowledge Check
If the point A1,A2,….,A8 be the affixes of the roots of the equation z8−1=0 in the argand plane, then the area of the triangle A3A6A7 is equal to
A√2B√3C1√2D1√3The number of ways in which ten candidates A1,A2,....,A10 can be ranked so that A1 is always above A2 is
A10!2B10!C9!D8!2If the equation of the locus of a point equidistant from the points (a1,b1) and (a2,b2) is (a1−a2)x+(b2+b2)y+c=0, then the value of C is
Aa21−a22+b21−b22B√a21+b21−a22−b22C12(a21+a22+b21+b22)D12(a21+b22+a21+b22)
F is the set of all onto functions from A={a1,a2.......an}→B={x,y,z} and fεF is chosen randomly. What is the probability that x is the image of two elements in A.
View SolutionFor a post three person A1,A2,A3 appear in the interview. The probability of A1 being selected is twice as much as that of A2 and the probability of A2 being selected is three times as much as that of A3. What are the individual probabilities of A1,A2,A3 being selected?
View SolutionSuppose a1,A2,.....,A30 are thirty sets each with five elements and B1,B2,.......,Bn are n sets each with 3 elements. Let 30⋃i=1Ai=n⋃i=1=S. Assume that each element of S belongs to exactly 10 elements of Ai and exactly 9 of Bj. Find n
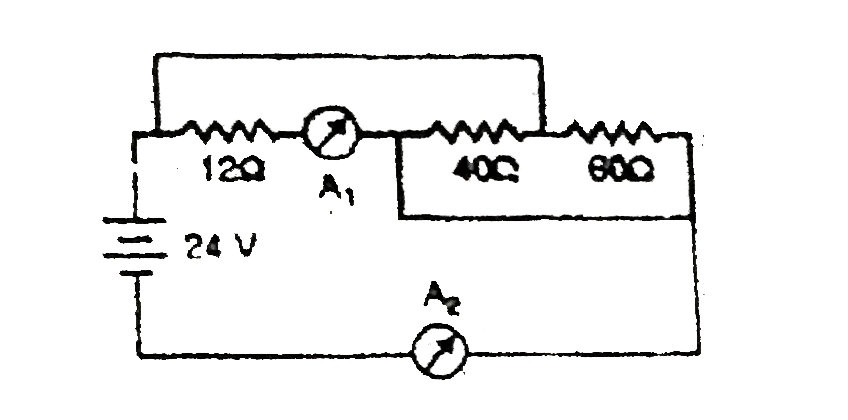
View SolutionIn the circuit of figure A1 and A2 are ideal ammeters. Then the reading in ammeter A2 is
View SolutionThe capacitance of the capacitors of plate areas A1 and A2(A1<A2) at a distance d is

View Solution
