Find absolute work done to stop the disc rolling on horizontal surface with 0.4 m/s velocity having mass 50 Kg and radius R
4 J
2 J
6 J
8 J
The correct Answer is:C
Topper's Solved these Questions
Similar Questions
A disc is in the condition of pure rolling on the horizontal surface. The velocity of center of mass isV0 If radius of disc is R then the speed of point P is (OP=R3)
View SolutionA uniform circular disc of radius R is rolling on a horizontal surface. Determine the tangential velocity : the centre of mass
View Solution
Knowledge Check
A solid cylinder of mass 1 kg and radius 0.02 m, is rolling on a smooth horizontal surface with a uniform velocity of 0.1 m/s. Its total energy is
A7.5×10−3JB7.5×10−2JC7.5×10−4JD7.5×10−6JA disc of mass 2 kg is rolling on a horizontal surface without slipping with a velocity of 0.1 m/s. What is its rotational kinetic energy?
A5×10−3JB2.5×10−3JC15×10−3JD8×10−3JA disc of mass 4.8 kg and radius 1 m is rolling on a horizontal surface without sliding with angular velocity of 600 rotations/min. What is the total kinetic energy of the disc ?
A1440π2JB360JC600π2JD4000π2J
A disc of mass 4.8 kg and radius 1 m is rolling on a horizontal surface without sliding with angular velocity of 600 rotations/min. What is the total kinetic energy of the disc ?
View Solution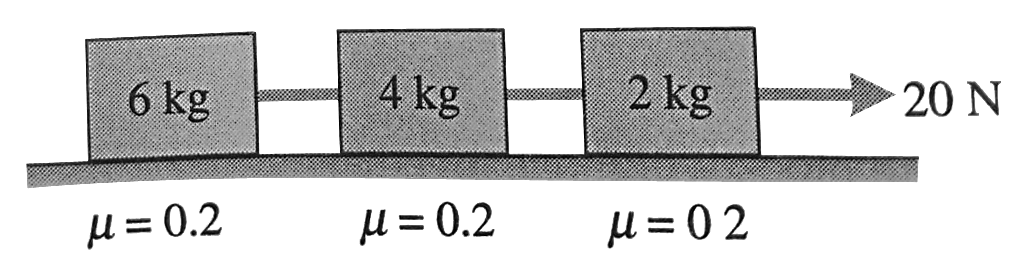
Three blocks of masses 6 kg, 4 kg and 2 kg are pulled on a rough surface by applying a constant force 20 N. The values of coefficient of friction between blocks and suface are shown in figure.
Q. In the arrangement shoen tension in the string connecting 4 kg and 6 kg masses is
View SolutionA circular disc of radius R rolls without slipping along the horizontal surface with constant velocity v0. We consider a point A on the surface of the disc. Then the acceleration of the point A is:
View SolutionA disc of mass 4 kg and of radius 1 m rolls on a horizontal surface without slipping such that the velocity of its centre of mass is 10 cm sec−1, Its rotatonal kinetic energy is
View SolutionA disc of mass 4 kg and of radius 1 m rolls on a horizontal surface without slipping such that the velocity of its centre of mass is 10 cm sec−1, Its rotatonal kinetic energy is
View Solution
