If mass is written as m=kcPG−12h12 then the value of "P" will be : (Constants have their usual meaning with "k" a dimensionless constant)
45352
-0.33333333333333
45323
1/2
To find the value of P in the equation m=kcPG−1/2h1/2, we will use dimensional analysis. We need to express each variable in terms of its fundamental dimensions: mass (M), length (L), and time (T).
Step 1: Identify the dimensions of each variable
1. Mass (m): The dimension is (M).
2. Speed of light (c): The dimension is (LT−1).
3. Gravitational constant (G): The dimension is (M−1L3T−2).
4. Planck's constant (h): The dimension is (ML2T−1).
Step 2: Write the dimensions of the right-hand side
The right-hand side of the equation is kcPG−1/2h1/2.
- For cP:
(cP)=(LT−1)P=(LPT−P)
- For G−1/2:
(G−1/2)=(M−1L3T−2)−1/2=(M1/2L−3/2T1)
- For h1/2:
(h1/2)=(ML2T−1)1/2=(M1/2L1T−1/2)
Step 3: Combine the dimensions on the right-hand side
Now, we combine the dimensions:
(kcPG−1/2h1/2)=(k)⋅(LPT−P)⋅(M1/2L−3/2T1)⋅(M1/2L1T−1/2)
Assuming k is dimensionless, we can ignore its dimensions. Now we combine the dimensions:
=(LP)⋅(M1/2M1/2)⋅(L−3/2L1)⋅(T−PT1T−1/2)
=(M1)⋅(LP−3/2+1)⋅(T−P+1−1/2)
=(M1)⋅(LP−1/2)⋅(T−P+1/2)
Step 4: Set up equations for each dimension
Now we equate the dimensions from both sides of the equation:
1. For mass (M):
1=1(this is satisfied)
2. For length (L):
P−12=0⟹P=12
3. For time (T):
−P+12=0⟹P=12
Conclusion
From both the length and time equations, we find that P=12.
Thus, the value of P is 12.
1. Mass (m): The dimension is (M).
2. Speed of light (c): The dimension is (LT−1).
3. Gravitational constant (G): The dimension is (M−1L3T−2).
4. Planck's constant (h): The dimension is (ML2T−1).
The right-hand side of the equation is kcPG−1/2h1/2.
(cP)=(LT−1)P=(LPT−P)
(G−1/2)=(M−1L3T−2)−1/2=(M1/2L−3/2T1)
(h1/2)=(ML2T−1)1/2=(M1/2L1T−1/2)
Now, we combine the dimensions:
(kcPG−1/2h1/2)=(k)⋅(LPT−P)⋅(M1/2L−3/2T1)⋅(M1/2L1T−1/2)
=(LP)⋅(M1/2M1/2)⋅(L−3/2L1)⋅(T−PT1T−1/2)
=(M1)⋅(LP−3/2+1)⋅(T−P+1−1/2)
=(M1)⋅(LP−1/2)⋅(T−P+1/2)
Now we equate the dimensions from both sides of the equation:
1. For mass (M):
1=1(this is satisfied)
P−12=0⟹P=12
−P+12=0⟹P=12
From both the length and time equations, we find that P=12.
Topper's Solved these Questions
Similar Questions
Obtain an expression for the centripetal force F acting on a particle of mass m moving with velocity v in a circle of radius r . Take dimensionless constant K = 1 .
View SolutionFor the reaction
N2(g)+3H2(g)⇔2NH3(g)
Equilibrium constant Kc=[NH3]2[N2][H2]3
Some reactions are written below in column I and their equilibrium constants in terms of Kc are written in Column II. Match the following reactions with the corresponding equilibrium constant.
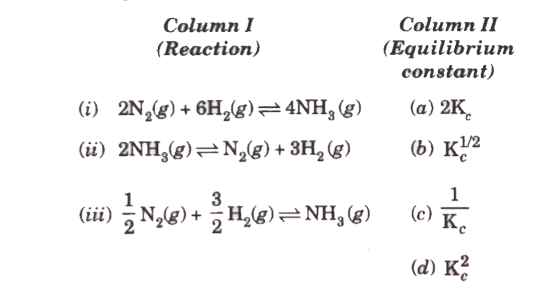
View Solution
Knowledge Check
STATEMENT-1 Dimensional constants are the quantities whose values are constant
STATEMENT 2 Quantities which are dimensionless are known as dimensional constantsAStatement-1 is True , Statement-2 is True ,Statement-2 is a correct explanation for Statement-1BStatement-1 is True , Statement-2 is True ,Statement-2 is NOT a correct explanation for Statement-1CStatement-1 is True , Statement-2 is FalseDStatement-1 is False, Statement-2 is TrueFor a given exothermic reaction, KpandK′p are the equilibrium constants at temperatures T1andT2 respectively. Assuming that heat of reaction is constant in temperature range between T1andT2, it is readily water that
AKp>Kp'BKp<Kp'CKp=Kp'DKp=1Kp'For the reaction H2(g)+I2(g)⇔2HI(g), the equilibrium constant Kp Changes with
Atotal pressureBcatalystCthe amount of H2andI2 presentDtemperature
(a)The value of equilibrium constant for the reaction:
H2(g)+I2(g)⇔2HI(g) is 48 at 270 K
What is the value of equilibrium constant for the reaction :
12H2(g)+12I2(g)⇔HI(g)
(b) Write equilibrium constant (Kc) for the reaction
Cu2+(aq)+2Ag(s)⇔Cu(s)+2Ag+ (aq)
View SolutionFor the reaction H2(g)+I2(g)⇔2HI(g)
the equilibrium constant Kp changes with
View SolutionFor the reaction H2(g)+I2(g)⇔2HI(g)
the equilibrium constant Kp changes with
View SolutionSTATEMENT-1: Dimensional constants are the quantities whose values are constant
STATEMENT-2: Dimensional constants are dimensionless
STATEMENT-3 Universal gravitational constant (G) is a dimensional constant
View SolutionThe speed (v) of sound in a gas is given by v=kPxρy
Where K is dimensionless constant, P is pressure, and ρ is the density, then
View Solution
