Consider a ring of mass m and radius r. Maximum gravitational intensity on the axis of the ring has value.
Gmr2
2Gm√3r2
2Gm3√3r2
Gm2r2
The correct Answer is:C
To find the maximum gravitational intensity on the axis of a ring of mass m and radius r, we can follow these steps:
Step 1: Understand Gravitational Intensity
Gravitational intensity (or gravitational field strength) g at a point in space due to a mass is given by the formula:
g=GMr2
where G is the gravitational constant, M is the mass creating the field, and r is the distance from the mass to the point where the field is being calculated.
Step 2: Set Up the Problem
Consider a ring of mass m and radius r. We want to find the gravitational intensity at a point along the axis of the ring. Let the distance from the center of the ring to the point on the axis be x.
Step 3: Calculate Gravitational Intensity on the Axis
The gravitational intensity g at a distance x from the center of the ring along the axis can be derived by integrating contributions from each infinitesimal mass element dm of the ring. The gravitational intensity due to a mass element dm at a distance d from the point on the axis is given by:
dg=Gdmd2
where d=√r2+x2 (using Pythagoras theorem).
Step 4: Integrate Over the Ring
Since the ring has a uniform mass distribution, we can integrate dg around the entire ring:
g=∫Gdmr2+x2
The total mass m can be expressed in terms of dm as dm=m2πrdθ where dθ is the angle subtended by the mass element at the center.
Step 5: Simplify the Expression
The gravitational intensity g can be simplified to:
g=Gm(r2+x2)3/2x
Step 6: Find Maximum Gravitational Intensity
To find the maximum gravitational intensity, we need to differentiate g with respect to x and set the derivative to zero:
dgdx=0
After differentiation and simplification, we find that the maximum occurs at x=r√2.
Step 7: Calculate Maximum Gravitational Intensity
Substituting x=r√2 back into the expression for g:
gmax=Gm(r2+(r√2)2)3/2⋅r√2
This simplifies to:
gmax=Gm(2r2)3/2⋅r√2=Gm2√2r3
Final Answer
Thus, the maximum gravitational intensity on the axis of the ring is:
gmax=Gm2√2r2
Gravitational intensity (or gravitational field strength) g at a point in space due to a mass is given by the formula:
g=GMr2
where G is the gravitational constant, M is the mass creating the field, and r is the distance from the mass to the point where the field is being calculated.
Consider a ring of mass m and radius r. We want to find the gravitational intensity at a point along the axis of the ring. Let the distance from the center of the ring to the point on the axis be x.
The gravitational intensity g at a distance x from the center of the ring along the axis can be derived by integrating contributions from each infinitesimal mass element dm of the ring. The gravitational intensity due to a mass element dm at a distance d from the point on the axis is given by:
dg=Gdmd2
where d=√r2+x2 (using Pythagoras theorem).
Since the ring has a uniform mass distribution, we can integrate dg around the entire ring:
g=∫Gdmr2+x2
The total mass m can be expressed in terms of dm as dm=m2πrdθ where dθ is the angle subtended by the mass element at the center.
The gravitational intensity g can be simplified to:
g=Gm(r2+x2)3/2x
To find the maximum gravitational intensity, we need to differentiate g with respect to x and set the derivative to zero:
dgdx=0
After differentiation and simplification, we find that the maximum occurs at x=r√2.
Substituting x=r√2 back into the expression for g:
gmax=Gm(r2+(r√2)2)3/2⋅r√2
This simplifies to:
gmax=Gm(2r2)3/2⋅r√2=Gm2√2r3
Thus, the maximum gravitational intensity on the axis of the ring is:
gmax=Gm2√2r2
Topper's Solved these Questions
- GRAVITATION BOOK - AAKASH INSTITUTECHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION -C (Objective Type Questions (More than one option are correct))
11 Videos
- GRAVITATION BOOK - AAKASH INSTITUTECHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION -D (Linked Comprehension Type Questions)
13 Videos
- GRAVITATION BOOK - AAKASH INSTITUTECHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION -A (Objective Type Questions (one option is correct))
50 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTECHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT SECTION - D
13 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTECHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
Similar Questions
Mass of 10 kg is distributed uniformly over a ring of radius 1m. Find the gravitational potential at a point lies on the axis of the ring at a distance of 1m from the centre.
View SolutionCalculate the moment of inertia of
a. a ring of mass M and radius R about an axis coinciding with the diameter of the ring.
b. as thin disc about an axis coinciding with the diameter.
View Solution
Knowledge Check
Moment of inertia of a ring of mass M and radius R about a tangent to the circle of the ring is
A52MR2B32MR2C12MR2DMR2Moment of inertia of a ring of mass M and radius R about a tangent to the circle of the ring is
AMR2B12MR2C32MR2D72MR2A thin circular ring of mass m and radius R is rotating about its axis perpendicular to the plane of the ring with a constant angular velocity ω. Two point particleseach of mass M are attached gently to the opposite end of a diameter of the ring. The ring now rotates, with an angular velocityω2. Then the ratiomM is
A1B2C12D√2
The maximum electric field intensity on the axis of a uniformly charged ring of charge q and radius R is at a distance x from the centre of the ring.The value of x is
View SolutionA particle of mass m is kept on the axis of a fixed circular ring of mass M and radius R at a distance x from the centre of the ring. Find the maximum gravitational force between the ring and the particle.
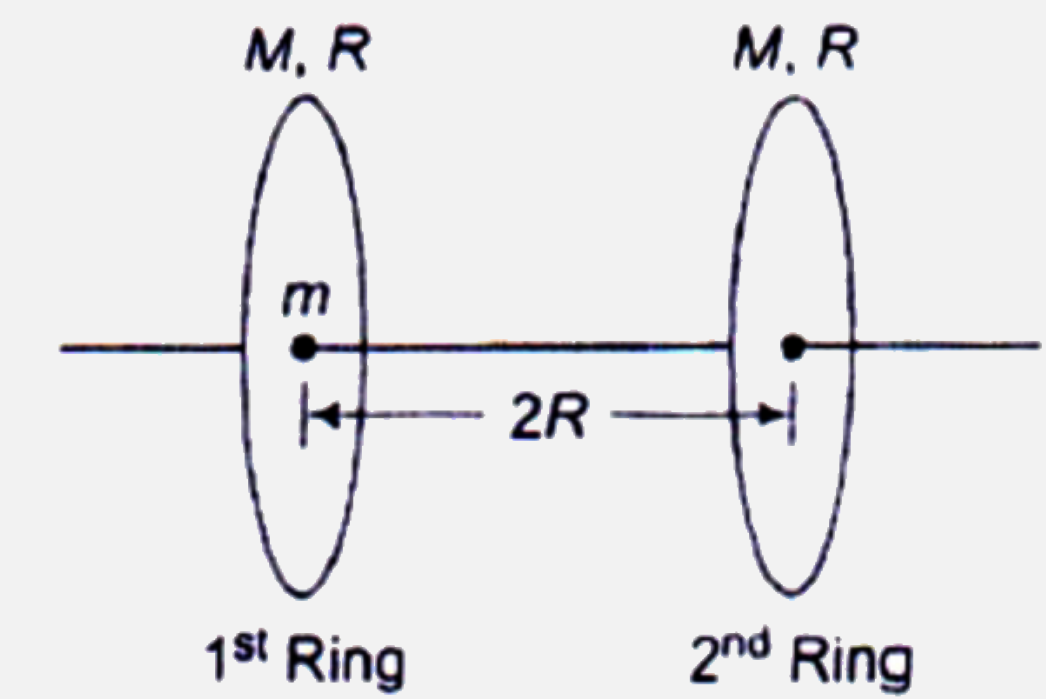
View SolutionConsider two identical rings of mass M and radius R fixed on a horizontal axis in a gravity - free space. A particle of mass m is held at rest at the centre of 1st ring and projected along the axis towards 2nd ring. The minimum speed of projection so that the particle reaches the centre of 2nd ring is
View SolutionFor a uniform ring of mass M and radius R at its centre
View SolutionThere is a ring or mass m and radius R is pivoted at a point O on its periphery. It is free to rotate about an axis perpendicular to its plane. What is the period of ring?
View Solution
