Circle K is inscribed in the first quadrant touching the circle radius of the circle K, is-
Step by step video & image solution for Circle K is inscribed in the first quadrant touching the circle radius of the circle K, is- by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
A circle touches the lines y=x√3,y=x√3 and unit radius. If the centre of this circle lies in the first quadrant then possible equation of this circle is
View SolutionIn a circle of radius R a square is inscribed, then a circle is inscribed in the square, a new square in the circle and so on for n times. If the ratio of the limit of the sum of areas of all the circles to the limit of the sum of areas of all the squares as n→∞ is k, then find the value of 4kπ.
View Solution
Knowledge Check
A square is inscribed in circle of radius R, a circle is inscribed in the square, a new square in the circle and so on for n times.
Sum of the areas of all circles isA4πR2(1−(12)n)B2πR2(1−(12)n)C3πR2(1−(13)n)DπR2(1−(12)n)The radius of the larger circle lying in the first quadrant and touching the line 4x+3y−12=0 and the coordinate axes, is
A5B6C7D8A square is inscribed in a circle then another circle is inscribed in the square . Another square is then inscribed in the circle. Finally a circle is inscirbed in the innermost square . Thus there are 3 circles and 2 squares as shown in the figure . The radius of the outer - most circle is R .
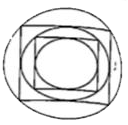
What is the radius of the inner - most circle ?AR2BR√2C√2RDnone of these
A square is inscribed in circle of radius R, a circle is inscribed in the square, a new square in the circle and so on for n times.
Sum of the areas of all circles is
View SolutionIn the given figure, PQR is a quadrant whose radius is 7 cm. A circle is inscribed in the quadrant as shown in the figure. What is the area (in cm2) of the circle
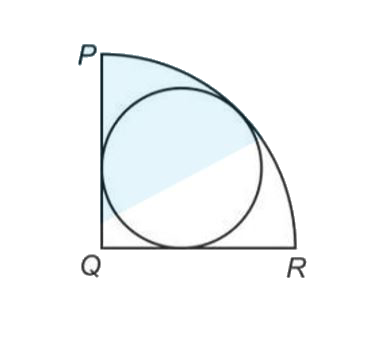
View SolutionThe radius of the larger circle lying in the first quadrant and touching the line 4x+3y−12=0 and the coordinate axes, is
View SolutionIn Figure-9, a square OPQR is inscribed in a quadrant OAQB of a circle. If the radius of circle is 6√2 cm, Find the area of the shaded region.
View SolutionIn Figure-9, a square OPQR is inscribed in a quadrant OAQB of a circle. If the radius of circle is 6√2 cm, Find the area of the shaded region.
View Solution
