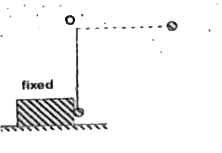
Two balls having masses m and 2m are fastened to two light strings of same length l figure. The other ends of the strings are fixed at O. The strings are kept in the same horizontal line and the system is released from rest. The collision between the balls is elastic. a. Find velocities of the balls just after their collision. b. How high will the balls rise after the collision.

Let the velocilty of m reaching at lower end
=v1
From work energy principle
∴(12)×mv21−(12)×m(0)2=mgl
→v1=√2gl
similarly velocity of heavy block will be ltbr> v2=sqt(2gl)
∴V1=v2=u(say)
Let fiN/A,l velocity of m and 2m are v1andv2 respectively.
According to law of conservation of momenum
→m×u−2μ=mv1+2mv2
→v1+2v2=−u
Aga∈v1−v−2=[(u−v)]=−2u
Substracting
3v2=u
→v2=u3=√2gl3
substituting in ii ltbr> v1−v2=−2u
→v1=−2u+v2
=−2u+(u3)
=−53u=−53×√2gl
√50gl3
b. putting the work energy principle
(1/2)xx2mxx(0)^2-(1/2)xx2mxx(v_2)^2<br>=-2mxxgxxh<br>[hrarrheightreachedbyheavyball]
→h=(L9)
similarly
(12)×mx(0)2−(12)×mv21=m×g×h2
[height reached by small ball] ltbr> (12)×50gL9g×h2
h2=0.25L9
some h2 is more than 2L the velocity at highest point will not be zero.
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMACHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective 2
11 Videos
- CALORIMETRYBOOK - HC VERMACHAPTER - CALORIMETRYEXERCISE - Exercises
18 Videos
- CIRCULAR MOTIONBOOK - HC VERMACHAPTER - CIRCULAR MOTIONEXERCISE - Exercises
30 Videos
Similar Questions
A glass ball collides with an identical ball at rest with v0=2 m/sec. If the coefficient of restitution of collision is e = 0.5, find the velocities of the glass balls just after the collision.
View SolutionA small ball is suspended from a fixed point 'O' by means of a light and inextensible string of length 'l'. The ball is first taken aside such that string becomes horizontal and then released from rest. At the borrom it collides with a fixed obstacle. The co-efficient of restitution is 'e'. Find the maximum angular deflection of the string after nth collision.
View Solution
Knowledge Check
Two balls having masses m and 2m are fasrtened to two light strings of same length (figure). The other ends of the strings are fixed at O. The strings are kept in the same horizontal line and the system is released from rest. The collision between the balls is elastic. Point A is the lowest point at which either ball can reach
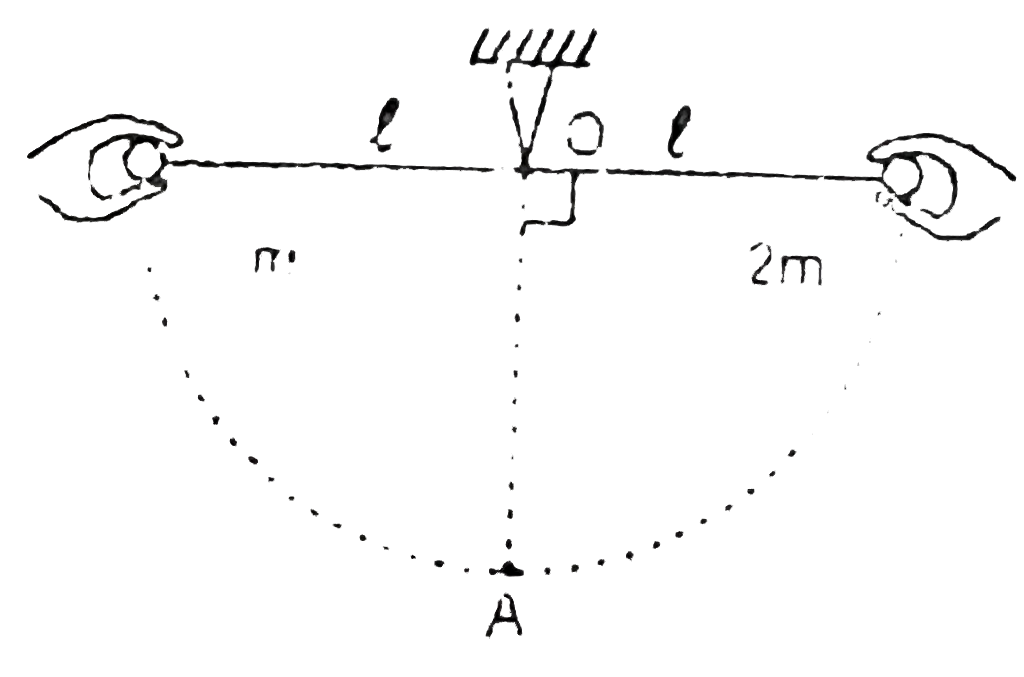
The position at which the balls will collide for the first timeAat point ABleft of point ACright of point ADNone of theseThe ball is released when the string is horizontal. The collision between the ball and the block is head - on elastic. The velocities of the ball and the block immediately after collision
 A23√2gL,13√2gLB−23√2gL,13√2gLC23√2gL,−13√2gLD−23√2gL,−13√2gL
A23√2gL,13√2gLB−23√2gL,13√2gLC23√2gL,−13√2gLD−23√2gL,−13√2gLTwo identical balls, each of mass m, are tied with a string and kept on a frictionless surface. Initially, the string is slack. They are given velocities 2u and u. in the same direction. Collision between the balls is perfectly elastic.

What is the final in kinetic energy of the balls?Amu2/4Bmu2/2C3mu2/4Dnone of these
Two balls of mass m and 2m are attached with strings of length 2L and L respectively. They are released from horizontal position. Find ratio of tensions in the string when the acceleration of both is only in vertical direction :
View SolutionTwo balls each of mass 'm' are moving with same velocity v on a smooth surface as shown in figure. If all collisions between the balls and balls with the wall are perfectly elastic, the possible number of collisions between the balls and wall together is

View SolutionA ball of mass m is fastened to a string. The ball swings in a vertical circle of radius R with the other end of the string held fixed. Neglecting the air resistance, the difference between the string's tension at the bottom of the circle and at the quarter of the cirlce is
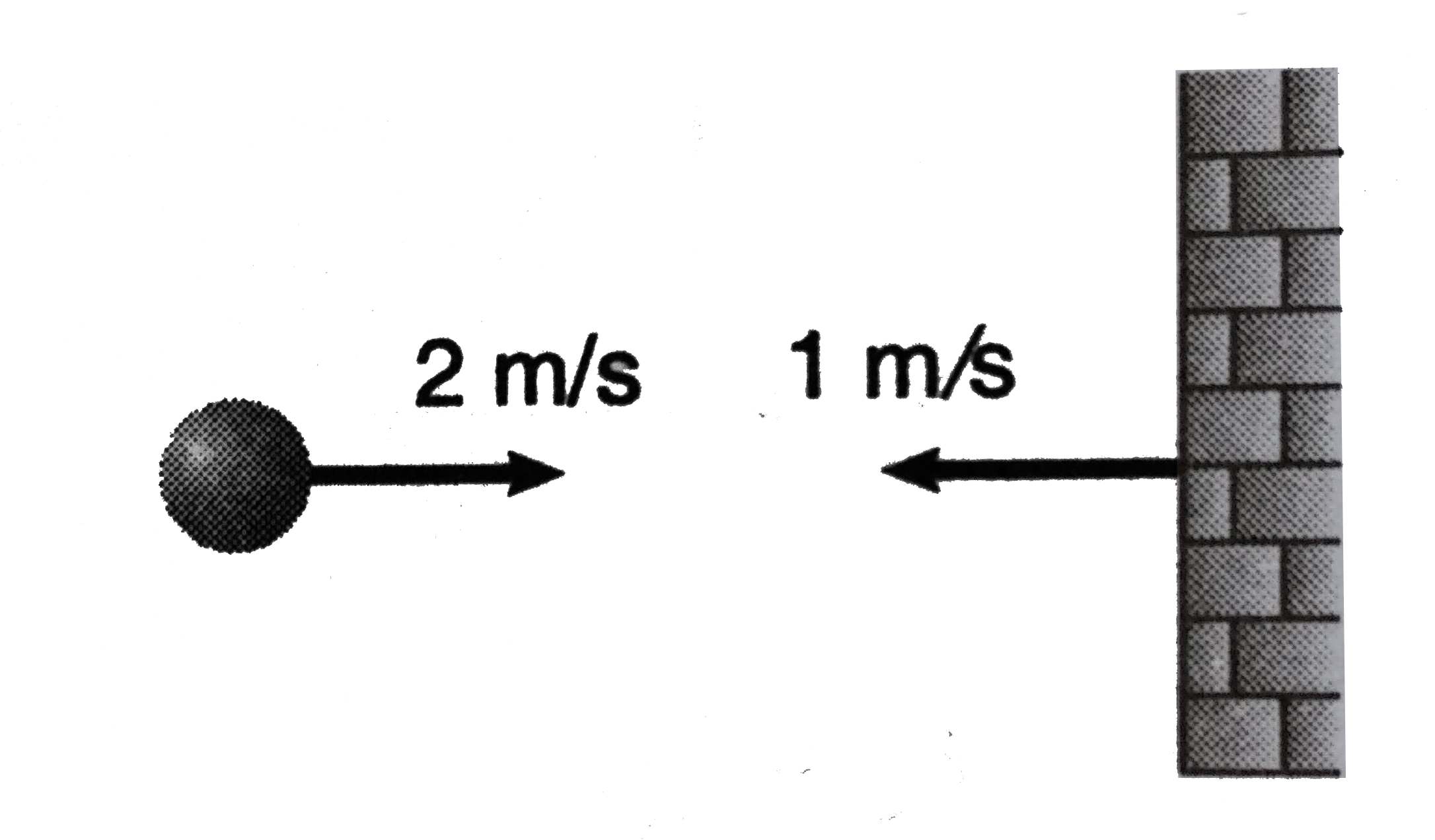
View SolutionA ball is moving with velocity 2m/s towards a heavy wall moving towards the ball with speed 1m/s as shown in figure. Assuming collision to be elastic, find the velocity of ball immediately after the collision.
View SolutionA steel ball of mass 0.5kg is fastened to a cord 20cm long and fixed at the far end and is released when the cord is horizontal. At the bottom of its path the ball strikes a 2.5kg steel block initially at rest on a frictionless surface. The collision is elastic. The speed of the block just after the collision will be.
View Solution
