The small orifice between two communicating vessels have area 1 sq. cm. The surface area of horizontal sections of the first and the second vessel are 10m2 and 5m2. Initially the level of liquid in the first and second vessel is 15 m and 5 m from the orifice. Given that velocity of liquid flow is v=σ√2g(h1−h2) m/s, where h1 and h2 is the height of liquid in the two vessels, then the time required for the liquid to reach the same level is 10t15σ√20g, where t is equal to _?
Step by step video solution for The small orifice between two communicating vessels have area 1 sq. cm. The surface area of horizontal sections of the first and the second vessel are 10m^2 and 5m^2. Initially the level of liquid in the first and second vessel is 15 m and 5 m from the orifice. Given that velocity of liquid flow is v= sigma sqrt(2g(h_(1)-h_(2))) m/s, where h_1 and h_2 is the height of liquid in the two vessels, then the time required for the liquid to reach the same level is 10^t/(15 sigma sqrt(20g), where t is equal to _? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023समान प्रश्न
A conducting liquid is filled in a vessel of area of cross section A upto height h then resistance of the liquid is
View SolutionA cylindrical vessel of uniform cross-section contains liquid upto the height' H’ . At a depth H=H2 below the free surface of the liquid there is an orifice. Using Bernoulli's theorem, find the velocity of efflux of liquid.
View Solution
ज्ञान जांच
If the surface tension of a liquid is 5 N/m then the surface energy of the liquid film on a ring of area 0.15 m2 is
A1.5JB3.0JC2.25JD0.75JAn open vessel containing the liquid upto a height of 15 m. A small hole is made at height of 10 m from the base of the vessel then the initial velocity of efflux is (g = 10 m/s2)
A1 m/sB10√2msC5 m/sD10 m/sA liquid having area of free surface A and has an orifice at a depth of h with an area a, below the liquid surface, then find the velocity v of flow through the orifice
Av=√2ghBv=√2gh√A2A2−a2Cv=√2gh√AA−aDv=√2gh√A2−a2A2
An open vessel containing the liquid upto a height of 15 m. A small hole is made at height of 10 m from the base of the vessel then the initial velocity of efflux is (g = 10 m/s2)
View SolutionFor maximum range R one orifice is made at the depth of h from the upper surface of incompressible liquid. If the height of liquid column in open vessel is 100 m then the value of h is
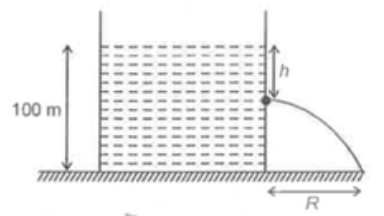
View SolutionFor maximum range R an orifice is made at the depth of h from the upper surface of incompressible liquid. If the height of liquid column in open vessel is 100 m then the value of h is

View SolutionWhat is filled in a cylindrical container to a height of 3m. The ratio of the cross-sectional area of the orifice and the beaker is 0.1. The square of the speed of the liquid coming out from the orifice is (g=10m/s2).
View SolutionWater is filled in a cylindrical container to a height of 3m. The ratio of the cross-sectional area of the orifice and the beaker is 0.1. The square of the speed of the liquid coming out from the orifice is (g=10m/s2).
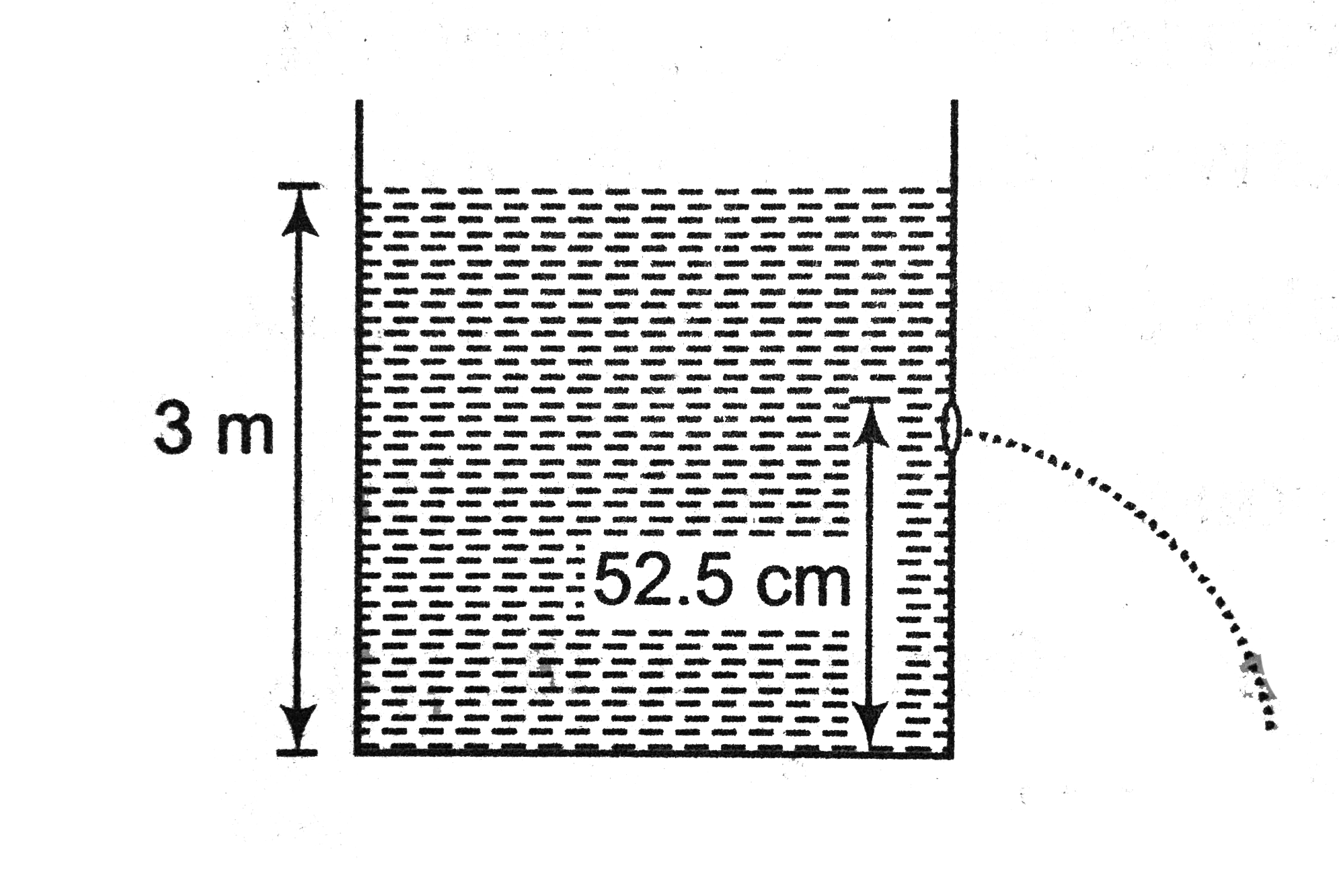
View Solution