An electron makes (3×105) revolutions per second in a circle of radius 0.5 angstrom. Find the magnetic field B at the centre of the circle.
To solve the problem of finding the magnetic field B at the center of a circular path where an electron makes 3×105 revolutions per second with a radius of 0.5 angstrom, we can follow these steps:
1. Convert the radius from angstroms to meters:
Radius(r)=0.5angstrom=0.5×10−10m
2. Calculate the current (I):
- The charge of an electron q=1.6×10−19C.
- The frequency of revolution f=3×105revolutions/second.
- The current can be calculated using the formula:
I=q×f
I=1.6×10−19C×3×105rev/s
I=4.8×10−14A
3. Use the formula for the magnetic field at the center of a circular loop:
The magnetic field B at the center of a circular loop is given by:
B=μ0I2r
where μ0=4π×10−7T m/A.
4. Substitute the values into the formula:
B=4π×10−7T m/A×4.8×10−14A2×(0.5×10−10m)
Simplifying the denominator:
2×(0.5×10−10)=10−10m
Now substituting:
B=4π×10−7×4.8×10−1410−10
5. Calculate the magnetic field:
B=4.8×4π×10−7×1010×10−14
B=4.8×4π×10−11T
B≈6.03×10−10T
Final Answer:
The magnetic field B at the center of the circle is approximately:
B≈6.03×10−10T
Radius(r)=0.5angstrom=0.5×10−10m
- The charge of an electron q=1.6×10−19C.
- The frequency of revolution f=3×105revolutions/second.
- The current can be calculated using the formula:
I=q×f
I=1.6×10−19C×3×105rev/s
I=4.8×10−14A
The magnetic field B at the center of a circular loop is given by:
B=μ0I2r
where μ0=4π×10−7T m/A.
B=4π×10−7T m/A×4.8×10−14A2×(0.5×10−10m)
Simplifying the denominator:
2×(0.5×10−10)=10−10m
Now substituting:
B=4π×10−7×4.8×10−1410−10
B=4.8×4π×10−7×1010×10−14
B=4.8×4π×10−11T
B≈6.03×10−10T
The magnetic field B at the center of the circle is approximately:
B≈6.03×10−10T
Topper's Solved these Questions
- MAGNETIC FIELD DUE TO CURRENTBOOK - HC VERMACHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Objective 2
7 Videos
- MAGNETIC FIELDBOOK - HC VERMACHAPTER - MAGNETIC FIELDEXERCISE - Exercises
61 Videos
- MAGNETIC PROPERTIES OF MATTERBOOK - HC VERMACHAPTER - MAGNETIC PROPERTIES OF MATTEREXERCISE - Exercises
9 Videos
Similar Questions
A point charge 3.2×10−19C makes 7×1015 revolutions per second in a circular path of radius 1Å . Calculate the magnetic induction at the centre of the circular path.
View SolutionIn a hydrogen atom the electron is making 4×107 revolution per minute in a path of radius 0.5 A∘. Find the magnetic field produced at the centre of orbit.
View Solution
Knowledge Check
A helium nucleus makes a full rotation in a circle of radius 0.8 m in 2 s. The value of the magnetic field B at the centre of the circle will be
A1019μ0B10−19μ0C2×10−10μ0D2×1010μ0A helium nucleus makes full rotation in a circle of radius 0.8 m in 2.5 seconds. The value of magnetic field B at the centre of the circle will be
A3.1π×10−26TB2π×10−26TC4π×10−25TD2π×10−25TA helium nucleus makes a full rotation in a circle of radius 0.8 metre in two seconds. The value of the magnetic field B at the centre of the circle will be
A10−19μ0B10−19μ0C2×10−10μ0D2×10−10μ0
In hydrozen atom, the electron is making 6.6×1015 revolution per second in a circular path of radius 0.53A∘. What is the magnetic induction produced at the centre of the orbit?
View Solution3 charges are placed in a circle of radius d as shown in figure. Find the electric field along x-axis at centre of circle.
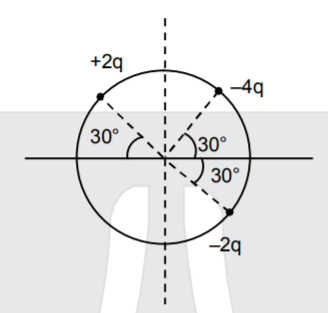
View SolutionA charge q coulomb moves in a circle at n revolution per second and the radius of the circle is r metre. Then magnetic feild at the centre of the circle is
View SolutionAn electron revolves in a circle of radius 0.4 Å with a speed of 105m/s. The magnitude of the magnetic field produced at the centre of the circular path due to the motion of the electron in W/m2 is
View SolutionA particle carrying a charge equal to 100 times the charge on an electron is rotating per second in a circular path of radius 0.8metre. The value of the magnetic field produced at the centre will be (μ0= permeability for vacuum)
View Solution
