If is a complex number such that then the minimum value of is
A
is strictly greater than
B
is strictly greater than but less than
C
is equal to
D
lies in the interval (1,2)
Video Solution
Text Solution
The correct Answer is:D
We observe that is the set of all points lying on or outside the circle centred at the origin and radius 2 and is the distance of any point in S from the point . It is evident from Fig. that AP is minimum when P coincides with B and in that case which lies in the interval (1,2).
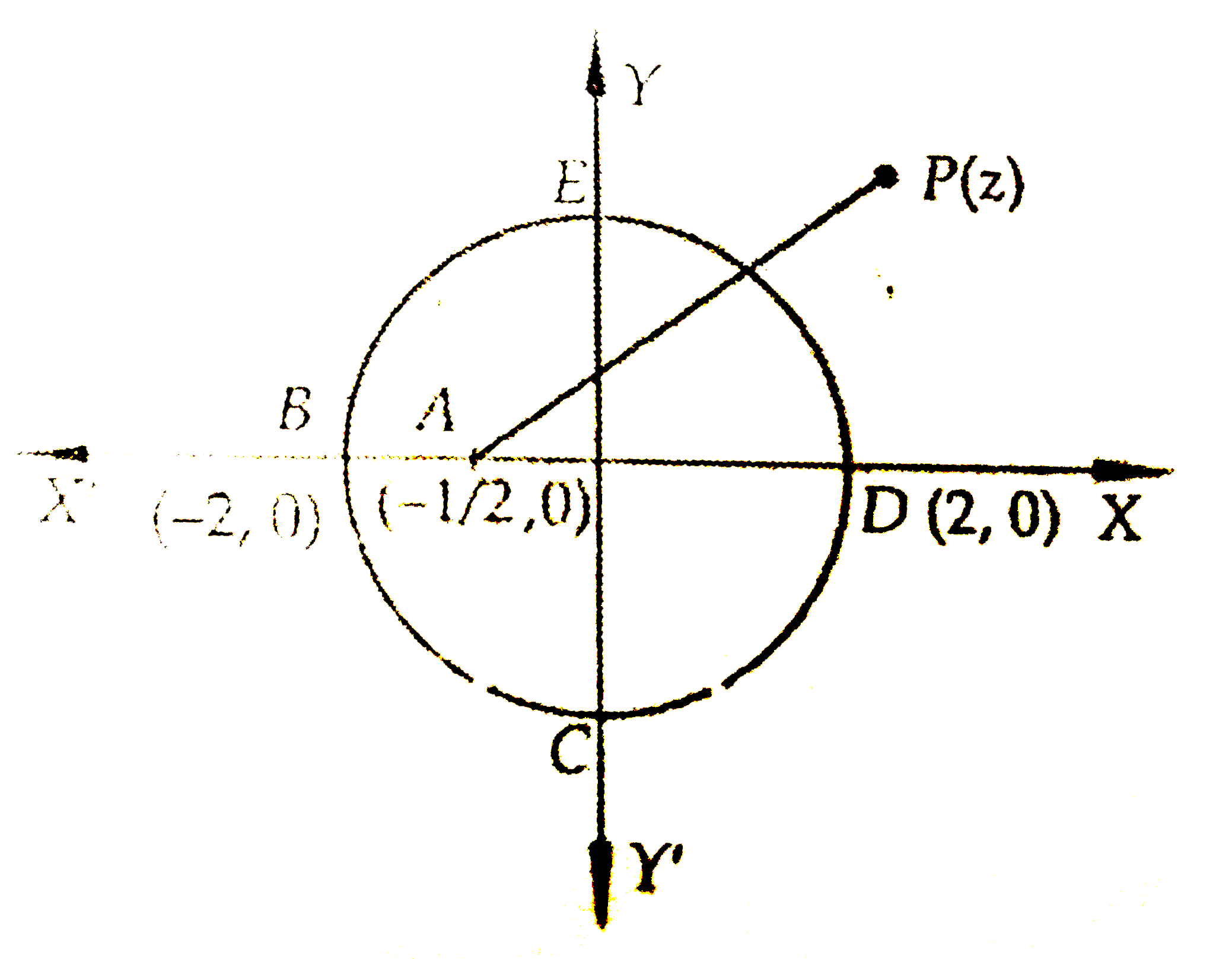
|
Topper's Solved these Questions
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Section II - Assertion Reason Type
15 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Exercise
131 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test
59 Videos
- CIRCLESBOOK - OBJECTIVE RD SHARMACHAPTER - CIRCLESEXERCISE - Chapter Test
55 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMACHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise
86 Videos
Similar Questions
Knowledge Check
If z is complex number such that |z|≥2 then the minimum value of ∣∣∣z+12∣∣∣
Ais equal to 52Blies in the interval [1, 2]Cis strictly greater than 32Dis strictly greater than 32 but less than 52If z is a complex number satisfying ∣∣z2+1∣∣=4|z|, then the minimum value of |z| is
A2√5+4B2√5−4C√5−2Dnone of theseIf z is any complex number satisfying |z−3−2i|≤1 then the minimum value of |2z−6+5i| is
A5B6C7D0
If z be the complex number such that ∣∣∣z+1z∣∣∣=2 then minimum value of |z|tan(π8) is
View Solutionz is a complex number such that z2+z+1=0 .Then the value of z30 is
View SolutionIf z is a complex number, then the minimum value of |z−2.8|+|z−1.42| is ____________
View SolutionIf z is a complex number, then find the minimum value of |z|+|z−1|+|2z−3|.
View SolutionIf z is a complex number, then the minimum value of |z|+|z−1| is -
View Solution
