Statement 1 : The plots of probability density and radial probability function versus distance r from the nucleus for any particular orbital are not identical,
Statement 2 : Probability density is whereas radial probability function represents probability of finding the electron in a shell of thickness dr.Solution in Malayalam
If both (A) and (R) are correct and (R) is the correct explanation of(A).
If both (A) and (R) are correçt, but (R) is not the correct explanation of (A).
If (A) is correct, but (R) is incorrect.
If both (A) and (R) are incorrect.
The correct Answer is:A
The plots are not identical. The probability density is along the radial distance whereas radial probability function is the probability in a shell of thickness dr.
Similar Questions
If P(r) is the radial probability density for a hydrogen atom then the probability that the separation of the electron and proton is between r and r+ dr is
View SolutionThe variation of radial probability density R^2 (r) as a function of distance r of the electron from the nucleus for 3p orbital: (A)(a) (b) (b) (c) (d)
View SolutionRadial probability density function || Illustration OF Radial probability density function
View SolutionThe radial probability is the probability of finding electron in a small spherical shell around the nucleus at a particular distance r. Hence radial probability is
View SolutionWhich of the following plots of radial - probability function 4π2ψ2 is /are correct
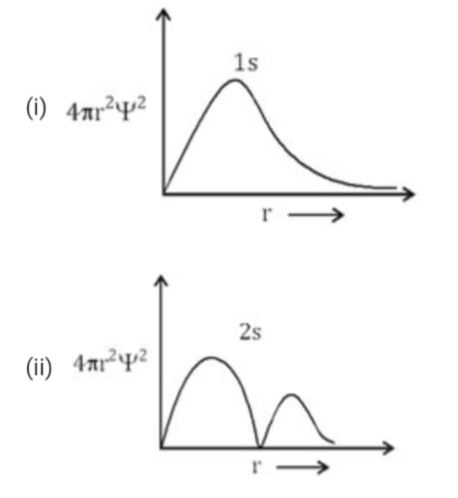
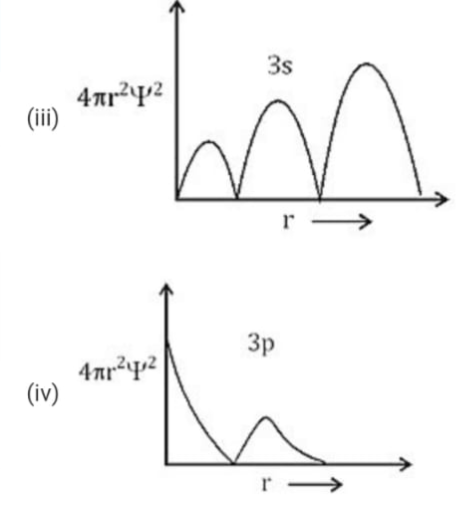
View SolutionSelect the correct plot of radial probability function (4πr2R2) for 2s - orbital.
View SolutionRepresent graphically, the variation of probability density (ψ2_(r)) as a function of distance (r ) of the electron from the nucleus for 1s and 2s orbitals.
View SolutionThe variation of radial probability density R^2 (r) as a function of distance r of the electron from the nucleus for 3p orbital:
View Solution
