A ball is kept at a height h above the surface of a heavy transparent sphere made of a material of refractive index The radius of the sphere is R. At t = 0, the ball is dropped to fall normally on the sphere. Find the speed of the image formed as a function of time for t<√2hg. Consider only the image by a single refraction.
The correct Answer is:A, B
At time t<√2hg,u=h−12>2,μ1=1

mu/v+g/u=(mu-1)/R<br>mu/v=(mu-1)/R-1/mu=(mu(mu-1)-R)/(muR)<br>v/mu=(uR)/(u(mu-1)-R)<br>=((h-1/2g^2)R)/((h-1/2gt^2)(mu-1)-R)<br>1/muxx(dv)/(dt)=[R(-gt)[(h-1/2gt^2)(mu-1)-R]]<br>+[R(-gt)(h-1/2gt^2)(mu-1)-R]^2<br>(R(h-1/2gt^2))gt(mu-1)<br>(dv)/(dt)xx1/mu=(gtxxR^2)/([(h-1/2gt^2)(mu-1)-R]^2)<br>(dv)/(dt)=(gtxxR^2)/([(h-1/2gt^2)(mu-1)-R]^2)<br>=μ>R2[(h−12>2)(μ−1)−R]2
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXAMPLE
9 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Question For short Answer
18 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Objective -2
7 Videos
- GAUSS LAWBOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Short Question
7 Videos
- LIGHT WAVESBOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Question for short Answer
11 Videos
Similar Questions
A hollow sphere made of a transparent material of refractive index 1.5 has a small mark on its surface as shown. The mark is observed from the farther side. Find the distance of the image of mark from the centre of the sphere.

View SolutionDoes the escape speed of a body from the earth depend on.
(i)mass of the body
(ii)the location from where it is projected
(iii)the direction of projection
(iv)the height of the location from where the body is launched?
View Solution
Knowledge Check
A transparent sphere of radius R and refractie index μ is kept in air. At what distance from the surface of the sphere shold a point object be placed so as to form a rea image at the same distance from the sphere?
AR/μBμRCRμ−1DRμ+1A sphere made of transparent material of refractive index (μ=32 )and of radius 50 cm has a small air bubble 10 cm below the surface. The apparent depth of the bubble if viewed from outside normally is
A507 cmB257cmC1007 cmD757cmA parallel narrow beam of light is incident on the surface of a transparent hemisphere of radius R and refractive index μ=1.5 as shown. The position of the image formed by refraction at the image formed by refraction at the spherical surface only as
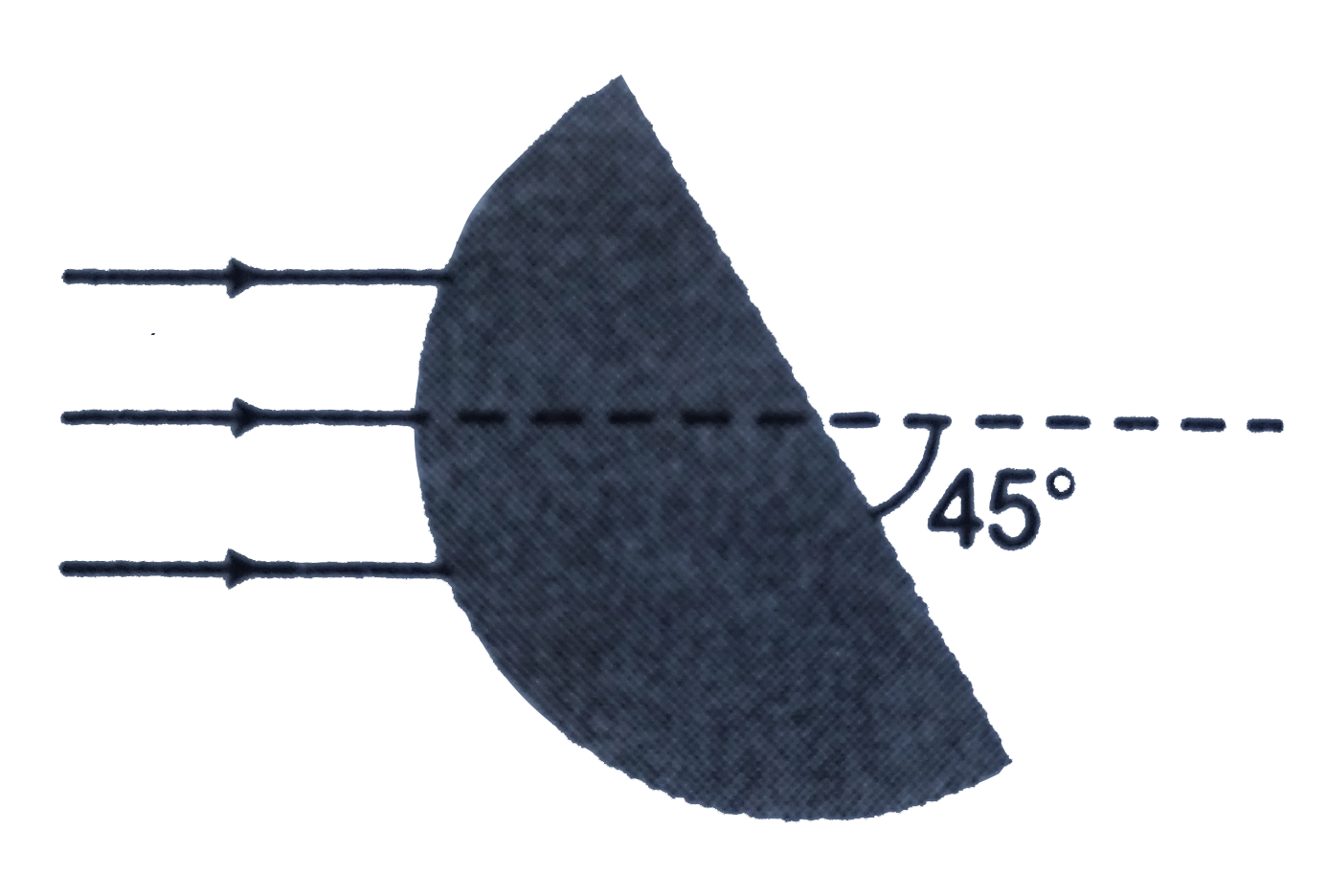 AR2B3RCR3D2R
AR2B3RCR3D2R
A sphere made of transparent material of refractive index (μ=32 )and of radius 50 cm has a small air bubble 10 cm below the surface. The apparent depth of the bubble if viewed from outside normally is
View SolutionA parallel beam of light is incident on the surface of a transparent hemisphere of radius R and refractive index 2.0 as shown in figure. The position of the image formed by refraction at the first surface is :

View SolutionA parallel narrow beam of light is incident on the surface of a transparent hemisphere of radius R and refractive index μ=1.5 as shown. The position of the image formed by refraction at the image formed by refraction at the spherical surface only as

View SolutionA narrow pencil of parallel light is incident normally on a solid transparent sphere of radius r. What should be the refractive index if the pencil is to be focused (a) at the surface of the sphere, (b) at the centre of the sphere.
View SolutionA narrow pencil of parallel light is incident normally on a solid transparent sphere of radius r. What should be the refractive index if the pencil is to be focussed (a) at the surface of the sphere, (b) at the centre of the sphere.
View Solution
