A ball is placed on a smooth inclined plane of inclination to the horizontal, which is rotating at frequency Hz about a vertical axiz passing through its lower end. At what distance from the lower end does the ball remain at rest?
The correct Answer is:D
To solve the problem, we need to analyze the forces acting on the ball placed on the inclined plane that is rotating about a vertical axis. Here’s a step-by-step solution:
Step 1: Understand the System
The inclined plane is at an angle θ=30∘ to the horizontal, and it is rotating with a frequency f=0.5 Hz. We need to find the distance d from the lower end of the inclined plane where the ball remains at rest.
Step 2: Convert Frequency to Angular Velocity
The angular velocity ω can be calculated using the formula:
ω=2πf
Substituting the given frequency:
ω=2π×0.5=πrad/s
Step 3: Analyze Forces Acting on the Ball
The forces acting on the ball are:
1. The gravitational force mg acting downwards.
2. The normal force N acting perpendicular to the surface of the inclined plane.
Step 4: Set Up the Equations
From the free body diagram, we can resolve the forces into components along the inclined plane:
- In the radial direction (perpendicular to the incline):
Ncosθ=mg
- In the tangential direction (along the incline):
Nsinθ=mrω2
where r is the horizontal distance from the axis of rotation to the ball.
Step 5: Solve for Normal Force
From the first equation:
N=mgcosθ
Step 6: Substitute Normal Force into the Second Equation
Substituting N into the second equation gives:
mgcosθsinθ=mrω2
Cancelling m from both sides:
gsinθcosθ=rω2
This simplifies to:
gtanθ=rω2
Step 7: Solve for r
Rearranging for r:
r=gtanθω2
Step 8: Substitute Known Values
Substituting g=10m/s2, θ=30∘, and ω=π:
tan30∘=1√3
Thus,
r=10⋅1√3π2
Step 9: Calculate r
Calculating r:
r=10√3⋅π2
Step 10: Calculate Distance d
The distance d from the lower end of the inclined plane can be found using:
d=rcosθ
Substituting cos30∘=√32:
d=10√3⋅π2√32=203π2
Step 11: Final Calculation
Calculating the numerical value:
d≈0.67m
Final Answer
The ball remains at rest at a distance of approximately 0.67m from the lower end of the inclined plane.
---
The inclined plane is at an angle θ=30∘ to the horizontal, and it is rotating with a frequency f=0.5 Hz. We need to find the distance d from the lower end of the inclined plane where the ball remains at rest.
The angular velocity ω can be calculated using the formula:
ω=2πf
Substituting the given frequency:
ω=2π×0.5=πrad/s
The forces acting on the ball are:
1. The gravitational force mg acting downwards.
2. The normal force N acting perpendicular to the surface of the inclined plane.
From the free body diagram, we can resolve the forces into components along the inclined plane:
- In the radial direction (perpendicular to the incline):
Ncosθ=mg
- In the tangential direction (along the incline):
Nsinθ=mrω2
where r is the horizontal distance from the axis of rotation to the ball.
From the first equation:
N=mgcosθ
Substituting N into the second equation gives:
mgcosθsinθ=mrω2
Cancelling m from both sides:
gsinθcosθ=rω2
This simplifies to:
gtanθ=rω2
Rearranging for r:
r=gtanθω2
Substituting g=10m/s2, θ=30∘, and ω=π:
tan30∘=1√3
Thus,
r=10⋅1√3π2
Calculating r:
r=10√3⋅π2
The distance d from the lower end of the inclined plane can be found using:
d=rcosθ
Substituting cos30∘=√32:
d=10√3⋅π2√32=203π2
Calculating the numerical value:
d≈0.67m
The ball remains at rest at a distance of approximately 0.67m from the lower end of the inclined plane.
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Match the columns
3 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Check point
45 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
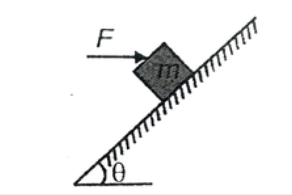
A block is placed on a smooth inclined plane as shown . For what value of horizontal force F, the block will remain at rest ?
View SolutionA small mass slides down an inclined plane of inclination θ with the horizontal the coefficient of friction is μ=μ0x, where x is the distance through which the mass slides down. Then the distance covered by the mass before it stops is
View SolutionA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View SolutionA body of mass m is placed over a smooth inclined plane of inclination θ. Which iis placed over a lift which is moving up with an acceleration a0 . Base length o f the inclined plane is L . Calculate the velocity of the block with respect to lift at the bottom, if it is allowed to slide down from the top of the plane from rest.
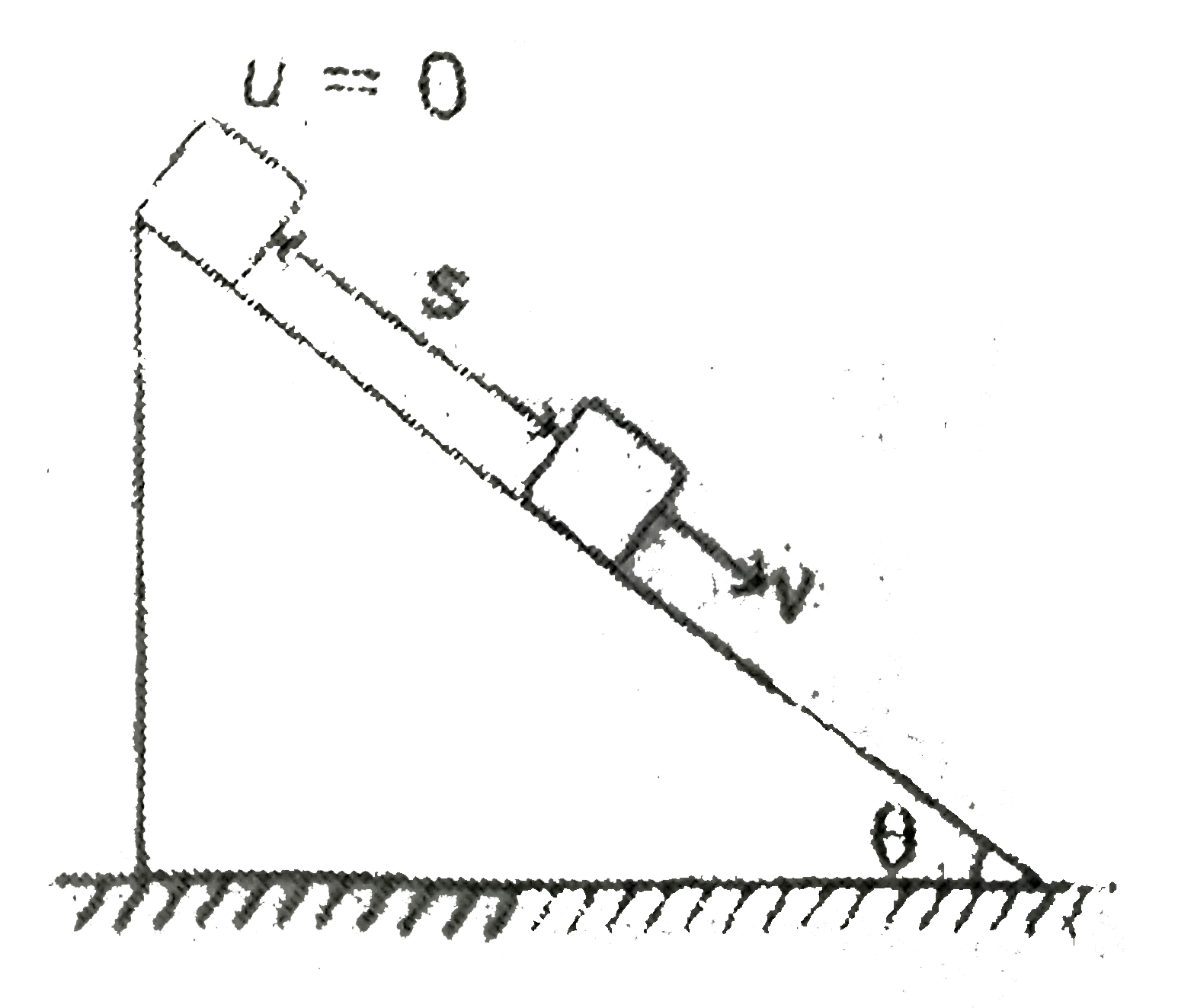
View SolutionA block is released from the top of a smooth inclined plane of inclination θ as shown in figure. Let υ be the speed of the particle after travelling a distance s down the plane. Then which of the following will remain constant ?
View SolutionA smooth inclined plane of length L, having an inclination θ with horizontal is inside a lift which is moving down with retardation a. The time taken by a block to slide down the inclined plane from rest will be
View SolutionA horizontal disk is rotating with angular velocity ω about a vertical axis passing through its centre. A ball is placed at the centre of groove and pushed slightly. The velocity of the ball when it comes out of the groove -

View SolutionA ball is projected form a point A on a smooth inclined plane which makes an angle a to the horizontal. The velocity of projection makes an angle θ with the plane upwards. If on the second bounce the ball is moving perpendicular to the plane, find e in terms of α and θ. Here e is the coefficient of restitution between the ball and the plane.
View SolutionA body of mass 'm' slides down a smooth inclined plane having an inclination of 45∘ with the horizontal. It takes 2S to reach the bottom. It the body is placed on a similar plane having coefficient friction 0.5 What is the time taken for it to reach the bottom ?
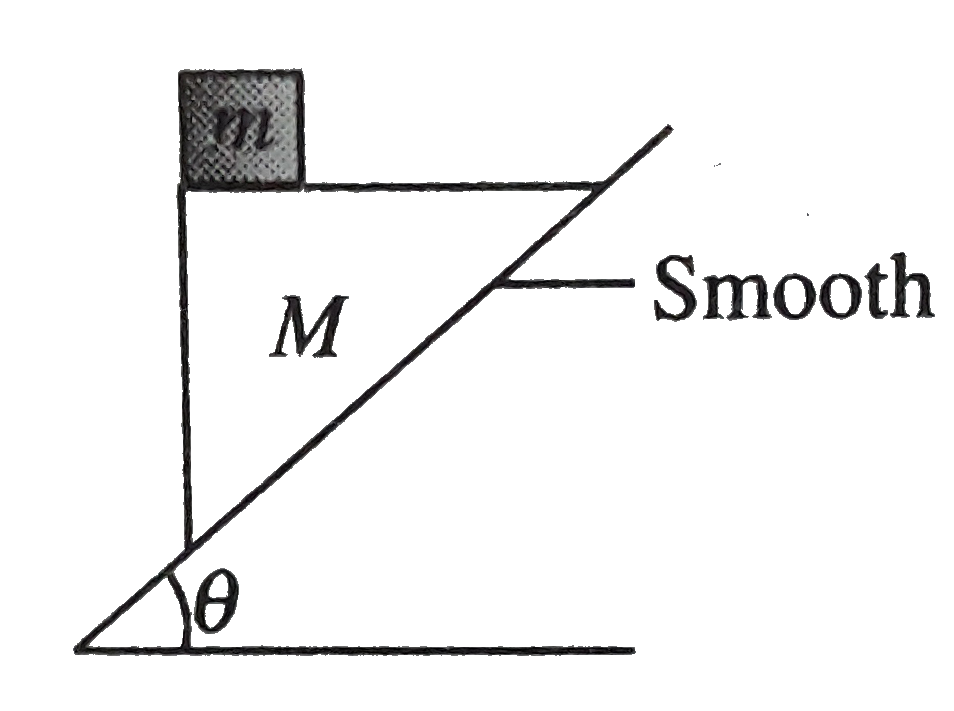
View SolutionA triangular prism of mass m placed on it is released from rest on a smooth inclined plane of inclination θ The block does not slip on the prism. Then
View Solution
