A heavy particle is tied to the end A of a string of the length1.6 m. Its other end O is fixed. It revolves as a conical pendulum with the string making 60∘ with the horizontal. Then,
its period of revolution is 4π7s
the tension is the string is 1√3 times the weight of the particle
the speed of the particle is 2.8√3ms−1
the centripetal acceleration of the particle is 9.8√3ms−2
The correct Answer is:D
To solve the problem of a heavy particle revolving as a conical pendulum, we can follow these steps:
Step 1: Understand the Geometry of the Problem
The length of the string (L) is given as 1.6 m, and the angle (θ) with the horizontal is 60°. The angle with the vertical will therefore be 30° (since 90° - 60° = 30°).
Step 2: Resolve Forces Acting on the Particle
The forces acting on the particle are:
1. The tension (T) in the string.
2. The weight (mg) of the particle acting downwards.
We can resolve the tension into two components:
- The vertical component: Tcos(30°)
- The horizontal component: Tsin(30°)
Step 3: Set Up the Equations of Motion
1. Vertical Forces: The vertical component of tension balances the weight of the particle.
Tcos(30°)=mg(Equation 1)
2. Horizontal Forces: The horizontal component of tension provides the centripetal force necessary for circular motion.
Tsin(30°)=mv2r(Equation 2)
where r is the radius of the circular path.
Step 4: Calculate the Radius of the Circular Path
The radius r can be calculated using the length of the string and the angle:
r=Lsin(60°)=1.6sin(60°)=1.6⋅√32=0.8√3m
Step 5: Substitute and Simplify
From Equation 1:
T=mgcos(30°)=mg√32=2mg√3
Substituting T into Equation 2:
2mg√3sin(30°)=mv2r
Since sin(30°)=12:
2mg√3⋅12=mv20.8√3
This simplifies to:
mg√3=mv20.8√3
Step 6: Cancel Mass and Solve for Speed
Cancel m from both sides:
g√3=v20.8√3
Cross-multiplying gives:
v2=0.8g
Thus,
v=√0.8g
Substituting g≈9.8m/s2:
v=√0.8⋅9.8≈√7.84≈2.8m/s
Step 7: Calculate the Time Period
The time period T of revolution is given by:
T=2πω
where ω=vr:
ω=2.80.8√3=2.81.3856≈2.02rad/s
Thus,
T=2π2.02≈3.11s
Summary of Results
- Speed of particle v≈2.8m/s
- Time period T≈3.11s
The length of the string (L) is given as 1.6 m, and the angle (θ) with the horizontal is 60°. The angle with the vertical will therefore be 30° (since 90° - 60° = 30°).
The forces acting on the particle are:
1. The tension (T) in the string.
2. The weight (mg) of the particle acting downwards.
- The vertical component: Tcos(30°)
- The horizontal component: Tsin(30°)
1. Vertical Forces: The vertical component of tension balances the weight of the particle.
Tcos(30°)=mg(Equation 1)
Tsin(30°)=mv2r(Equation 2)
where r is the radius of the circular path.
The radius r can be calculated using the length of the string and the angle:
r=Lsin(60°)=1.6sin(60°)=1.6⋅√32=0.8√3m
From Equation 1:
T=mgcos(30°)=mg√32=2mg√3
2mg√3sin(30°)=mv2r
Since sin(30°)=12:
2mg√3⋅12=mv20.8√3
This simplifies to:
mg√3=mv20.8√3
Cancel m from both sides:
g√3=v20.8√3
Cross-multiplying gives:
v2=0.8g
Thus,
v=√0.8g
Substituting g≈9.8m/s2:
v=√0.8⋅9.8≈√7.84≈2.8m/s
The time period T of revolution is given by:
T=2πω
where ω=vr:
ω=2.80.8√3=2.81.3856≈2.02rad/s
Thus,
T=2π2.02≈3.11s
- Speed of particle v≈2.8m/s
- Time period T≈3.11s
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Match the columns
3 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Check point
45 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
A particle is tied to the end of a string of length 20 cm and makes 5 rps. How many revolutions will it make in one second when the string is shortened to 10 cm ?
View SolutionA particle of mass 200 g , is whirled into a vertical circle of radius 80 cm uisig a massless string The speed of the particle when the string makes an angle of 60∘ with the vertical line is 1.5ms−1. The tension in the string at this position is
View SolutionA kite is attached to a string. Find the length of the string , when the height of the kite is 60 m and the string makes an angle 30∘ with the ground .
View SolutionA particle is moving in a circular path in the vertical plane. It is attached at one end of a string of length l whose other end is fixed. The velocity at lowest point is u. The tension in the string is T and acceleration of the particle is a at any position then T. a' is positive at the lowest point for
View SolutionA particle is moving in a circular path in the vertical plane. It is attached at one end of a string of length l whose other end is fixed. The velocity at lowest point is u . The tension in the string T and acceleration of the particle is a at any position. Then T.a is zero at highest point if
View SolutionA particle is fastened at the end of a string and whirled in a vertical circle with the other end of the string being fixed. The motion of the particle is
View SolutionA particle of maas m is attched to a light string of length l, the other end of which is fixed. Initially the string is kept horizontal and the particle is given an upwrd velocity v. The particle is just able to complete a circle
View SolutionA particle of mass 'm' is attached to one end of a string of length 'l' while the other end is fixed to a point 'h' above the horizontal table, the particle is made to revolve in a circle on the table, so as to make P revolution per sec. The maximum value of P if the particle is to be contact with the table is
View SolutionA particle of mass m and charge q is fastened to one end of a string of length. The other end of the string is fixed to the point O. The whole sytem liles on as frictionless horizontal plane. Initially, the mass is at rest at A. A uniform electric field in the direction shown in then switfched on. Then
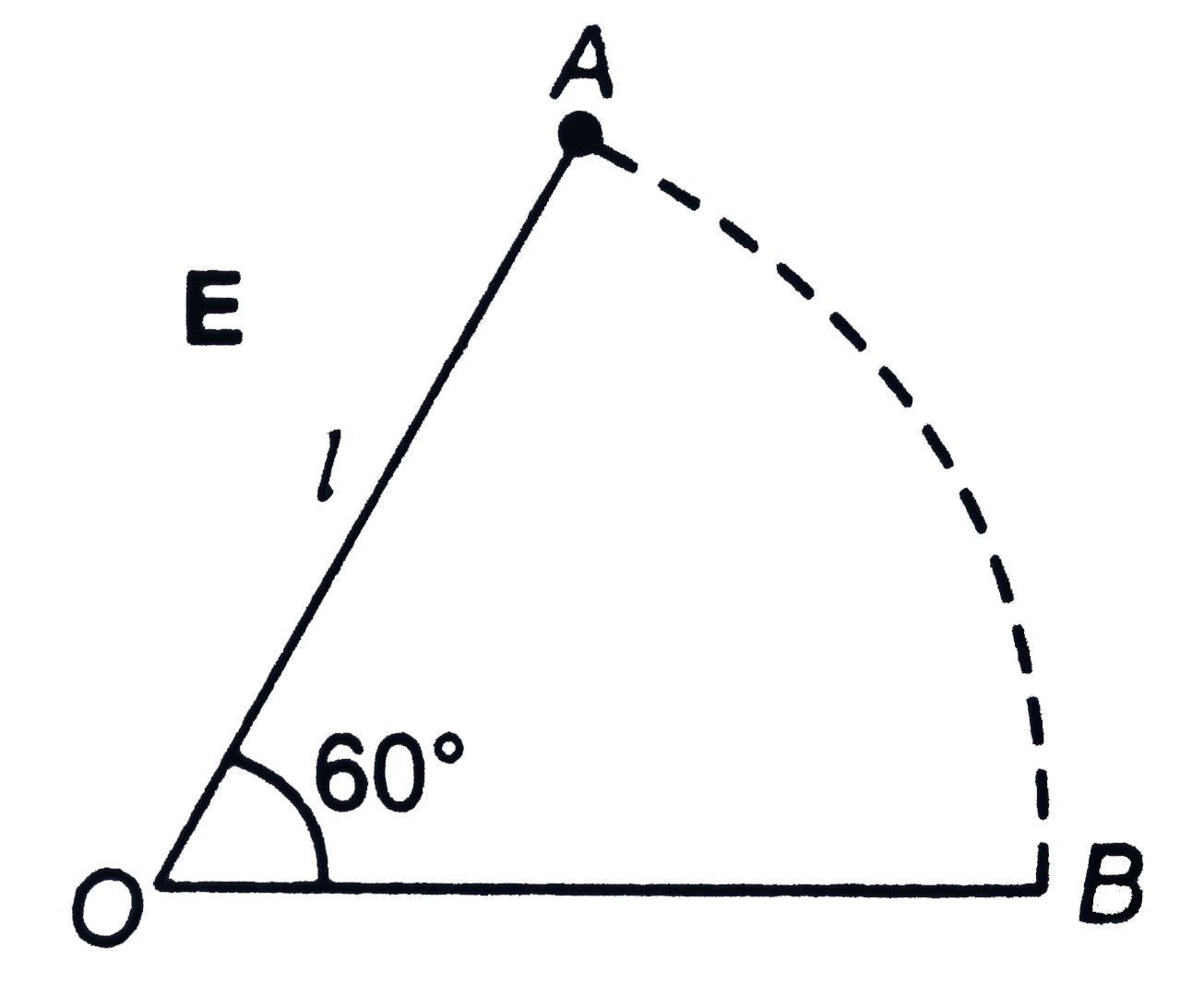
View SolutionA particle of mass m and charge q is fastened to one end of a string of length. The other end of the string is fixed to the point O. The whole sytem liles on as frictionless horizontal plane. Initially, the mass is at rest at A. A uniform electric field in the direction shown in then switfched on. Then

View Solution
