A ball is projected from a point on a smooth horizontal floor at an angle $θ$ with the horizontal . Speed of projection is u . Ball collides with the floor several times . Find the total time and horizontal distance covered by the ball .

Video Solution

Text Solution

Generated By DoubtnutGPT

To solve the problem of a ball projected at an angle $θ$ with an initial speed $u$ on a smooth horizontal floor, we need to find the total time of flight and the total horizontal distance covered by the ball after several collisions with the floor.
Step 1: Determine the time of flight for the first projectile motion
The time of flight for the first projectile motion can be calculated using the formula:
$T_{1} = \frac{2 u sin θ}{g}$
where $g$ is the acceleration due to gravity.
Step 2: Calculate the horizontal range for the first projectile motion
The horizontal range $R_{1}$ for the first projectile motion can be calculated using the formula:
$R_{1} = \frac{u^{2} sin 2 θ}{g}$
Step 3: Analyze the collisions and subsequent motions
After the first collision, the ball will rebound with a reduced vertical speed due to the coefficient of restitution $e$ . The vertical component of the velocity after the first collision will be:
$v = e \cdot u sin θ$
The angle of projection for the subsequent motion will be the same, but the vertical speed will be reduced.
Step 4: Calculate the time of flight for subsequent motions
For the second projectile motion, the time of flight $T_{2}$ can be calculated as:
$T_{2} = \frac{2 v sin θ}{g} = \frac{2 (e \cdot u sin θ)}{g} = \frac{2 e u sin θ}{g}$
Step 5: Calculate the horizontal range for subsequent motions
The horizontal range $R_{2}$ for the second projectile motion will be:
$R_{2} = \frac{v^{2} sin 2 θ}{g} = \frac{(e \cdot u sin θ)^{2} sin 2 θ}{g} = \frac{e^{2} u^{2} sin 2 θ}{g}$
Step 6: Generalize for all subsequent motions
The time of flight and horizontal range will continue to decrease by a factor of $e$ for each subsequent collision:
- Total time of flight:
$T_{total} = T_{1} + T_{2} + T_{3} + \dots = T_{1} (1 + e + e^{2} + \dots) = T_{1} \frac{1}{1 - e} = \frac{2 u sin θ}{g (1 - e)}$
- Total horizontal distance:
$R_{total} = R_{1} + R_{2} + R_{3} + \dots = R_{1} (1 + e + e^{2} + \dots) = R_{1} \frac{1}{1 - e} = \frac{u^{2} sin 2 θ}{g (1 - e)}$
Final Results
Thus, the total time of flight and the total horizontal distance covered by the ball are:
$T_{total} = \frac{2 u sin θ}{g (1 - e)}$
$R_{total} = \frac{u^{2} sin 2 θ}{g (1 - e)}$

A ball is projected from a point in a horizontal plane with a velocity of 20 m/s at $30^{\circ}$ with the horizontal. Coefficient of restitution is 0.8, then
Aduration for which ball will rebound is 20 s
Bduration for which ball will rebound is 10 s
Chorizontal component of velocity of the ball is 17.3 m/s.
Dhorizontal distance before the ball ceases to rebound is 173 m
A ball of mass $m$ is projected with a velocity $' u'$ at angle $θ$ with the horizontal. It collides with a smooth box of mass $' M'$ at its highest position. If the co-efficient of restitution is $' e'$ .

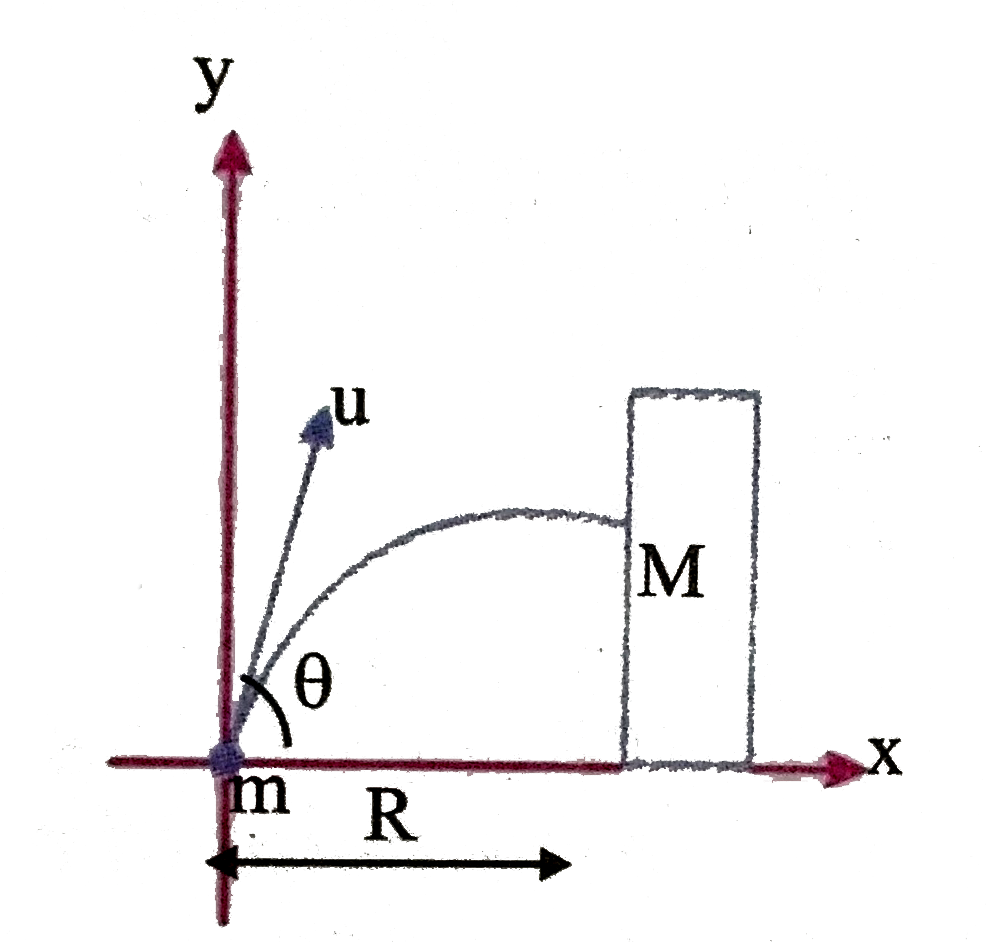
Find the horizontal distance travelled by the ball before collision.
A $\frac{u^{2} 2 sin θ cos θ}{g}$
B $\frac{u^{2} sin θ cos θ}{g}$
C $\frac{u^{2} sin θ cos θ}{2 g}$
D $\frac{u^{2} sin θ cos θ}{4 g}$
A ball is projected with initial velocity u at an angle $θ$ to the horizontal. Then horizontal displacement covered by ball as it collides third time to the ground would be, if coefficient of restitution is e :

A $(1 + e) \frac{u^{2} sin 2 θ}{g}$
B $e \frac{u^{2} sin 2 θ}{g}$
C $(1 - e) \frac{u^{2} sin 2 θ}{g}$
D $(1 + e + e^{2}) \frac{u^{2} sin 2 θ}{g}$