A block of mass M rests on a rough horizontal surface as shown. Coefficient of friction between the block and the surface is μ. A force F=mg acting at angle θ with the vertical side of the block pulls it. In which of the following cases the block can be pulled along the surface?

tanθ≥μ
tan(θ2≥μ)
cosθ≥μ
cot(θ2)≥μ
The correct Answer is:D
(d) N=Mg−Fcosθ
=Mg−Mgcosθ=2Mgsin2θ2
Further block can be pulled if,
Fsinθ≥μNcosθ
⇒ 2Mgsinθ2.cosθ2≥2μMgsin2θ2⇒cotθ2≥μ
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Assertion and reason
28 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Match the columns
7 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.4
20 Videos
- KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
A block of mass m rests on a rough horizontl surface as shown in figure (a) and (b). Coefficient of friciton between block and surface is μ. A force F-mg acting at an angle θ with the vertical side of the block. Find the condition for which block will move along the surface.
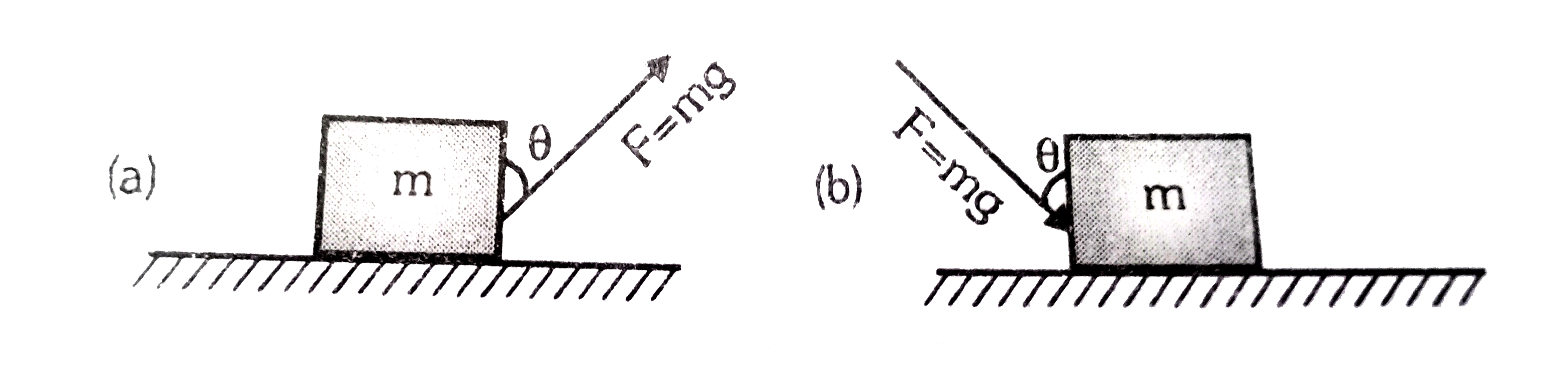
View SolutionA block of mass m rests on a rough horizontl surface as shown in figure (a) and (b). Coefficient of friciton between block and surface is μ. A force F-mg acting at an angle θ with the vertical side of the block. Find the condition for which block will move along the surface.

View Solution
Knowledge Check
A block of mass M is at rest on a rough horizontal surface. The coefficient of friction between the block and the surface is μ. A force F = Mg acting at an angle θ with the vertical side of the block pulls it. In which of the following cases, the block can be pulled along the surface?
Atanθ≥μBcotθ≥μCtanθ/2≥μDcotθ/2≥μA block is kept on a rough horizontal surface as shown. Its mass is 2 kg and coefficient of friction between block and surface (μ)=0.5. A horizontal force F is acting on the block. When
 AF = 4 N, acceleration is zero.BF = 4 N, friction is 10 N and acceleration is 3m/s2.CF = 14 N, acceleration is 2m/s2.DF = 14 N, friction is 14 N.
AF = 4 N, acceleration is zero.BF = 4 N, friction is 10 N and acceleration is 3m/s2.CF = 14 N, acceleration is 2m/s2.DF = 14 N, friction is 14 N.A block of mass M is using on a rough horizontal surface. μR is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
AMgBμMgCMg√1+μ2KDMg√1−μ2K
A block is kept on a rough horizontal surface as shown. Its mass is 2 kg and coefficient of friction between block and surface (μ)=0.5. A horizontal force F is acting on the block. When

View SolutionA block of mass m is moving on a rough horizontal surface. μ is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
View SolutionA block of mass 50 kg can slide on a rough horizontal surface. The coefficient of friction between the block and the surface is 0.6. The least force of pull acting at an
angle of 30∘ to the upwawrd drawn ve3rtical which causes the block to just slide is
View SolutionA block of mass 6 kg is being pulled by force 24 N as shown . If coefficient of friction between the block and the surface is 0.6 , frictional force acting on the block is :

View SolutionA block of mass 50 kg can slide on a rough horizontal surface. The coefficient of friction between the block and the surface is 0.6. The least force of pull acting at an
angle of 30∘ to the upwawrd drawn ve3rtical which causes the block to just slide is
View Solution
