A block slide down an incline of angle 30∘ with an acceleration g/4. Find the kinetic friction coefficient.
To solve the problem of finding the coefficient of kinetic friction for a block sliding down an incline at an angle of 30∘ with an acceleration of g4, we can follow these steps:
1. Identify the Forces Acting on the Block:
- The weight of the block W=mg acts vertically downward.
- The component of the weight parallel to the incline is W∥=mgsin(30∘).
- The component of the weight perpendicular to the incline is W_{\perpendicular} = mg \cos(30^\circ).
- The normal force N acts perpendicular to the incline and is equal to W_{\perpendicular}.
- The force of kinetic friction Fk acts opposite to the direction of motion, which is up the incline.
2. Write the Equation of Motion Along the Incline:
- According to Newton's second law, the net force acting along the incline is equal to the mass times acceleration:
mgsin(30∘)−Fk=ma
where a=g4.
3. Express the Kinetic Friction Force:
- The force of kinetic friction can be expressed as:
Fk=μkN
where μk is the coefficient of kinetic friction and N=mgcos(30∘).
4. Substituting the Normal Force:
- Substitute the expression for the normal force into the equation:
mgsin(30∘)−μk(mgcos(30∘))=m(g4)
5. Canceling Mass and Gravity:
- Since m and g are common in all terms, we can cancel them out:
sin(30∘)−μkcos(30∘)=14
6. Substituting the Values of Sine and Cosine:
- We know that sin(30∘)=12 and cos(30∘)=√32:
12−μk(√32)=14
7. Rearranging the Equation:
- Rearranging gives:
12−14=μk(√32)
14=μk(√32)
8. Solving for μk:
- Multiply both sides by 2√3:
μk=1/4⋅2√3=12√3
Final Answer:
The coefficient of kinetic friction μk is 12√3.
---
- The weight of the block W=mg acts vertically downward.
- The component of the weight parallel to the incline is W∥=mgsin(30∘).
- The component of the weight perpendicular to the incline is W_{\perpendicular} = mg \cos(30^\circ).
- The normal force N acts perpendicular to the incline and is equal to W_{\perpendicular}.
- The force of kinetic friction Fk acts opposite to the direction of motion, which is up the incline.
- According to Newton's second law, the net force acting along the incline is equal to the mass times acceleration:
mgsin(30∘)−Fk=ma
where a=g4.
- The force of kinetic friction can be expressed as:
Fk=μkN
where μk is the coefficient of kinetic friction and N=mgcos(30∘).
- Substitute the expression for the normal force into the equation:
mgsin(30∘)−μk(mgcos(30∘))=m(g4)
- Since m and g are common in all terms, we can cancel them out:
sin(30∘)−μkcos(30∘)=14
- We know that sin(30∘)=12 and cos(30∘)=√32:
12−μk(√32)=14
- Rearranging gives:
12−14=μk(√32)
14=μk(√32)
- Multiply both sides by 2√3:
μk=1/4⋅2√3=12√3
The coefficient of kinetic friction μk is 12√3.
Topper's Solved these Questions
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Objective -1
10 Videos
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Objective -2
5 Videos
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Questions for short Answer
11 Videos
- FLUID MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - All Questions
90 Videos
- GRAVITATIONBOOK - HC VERMA ENGLISHCHAPTER - GRAVITATIONEXERCISE - Question for short Answers
18 Videos
Similar Questions
A block of mass m slides down a rough inclined plane with an acceleration g2

View SolutionA block slides down on inclined plane (angle of inclination 60∘ ) with an accelration g/2 Find the coefficient friction

View SolutionA block slides down an inclined plane that makes an angle θ with the horizontal. If the coefficient of kinetic friction is μ, find the acceleration of the block.
View SolutionA block of mass 250 g slides down an incline of inclination 370 with a uniform speed. Find the work done against the friction as the block slides through 1.0 m.
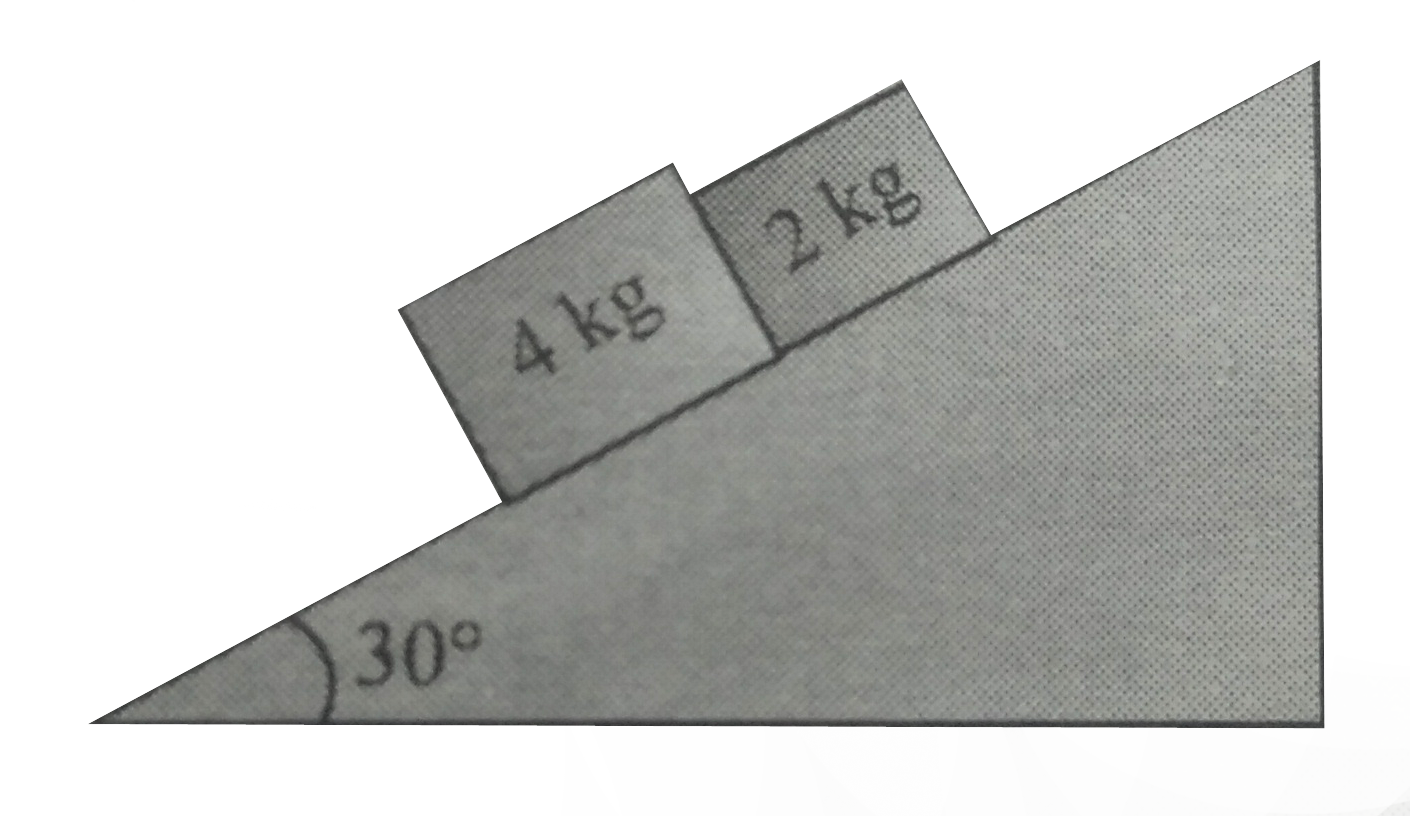
View SolutionFigure shown two blocks in contact sliding down an inclined surface of inclination 30∘. The friction coefficient between block of mass 2kgand the incline is μ1=0.20 and that between the block of mass 4kg and the incline is μ2=0.30 .Find the acceleration of 2.0kg block g=10ms−2
View SolutionA block of mass m slides down an inclined plane which makes an angle θ with the horizontal. The coefficient of friction between the block and the plane is μ. The force exerted by the block on the plane is
View SolutionA block of mass 5.0 kg slides down an incline of inclination 300 and length 10 m. find the work done by the force of gravity.
View SolutionAn ice cube is kept on an inclined plane of angle 30∘. The coefficient to kinetic friction between the block and incline plane is the 1/√3. What is the acceleration of the block ?
View SolutionA block of mass 2 kg slides down an incline plane of inclination 30°. The coefficient of friction between block and plane is 0.5. The contact force between block and plank is :
View SolutionA block slides down an inclined surface of inclination 300 with the horizontal. Starting from rest it covers 8 m in the first two seconds. Find the coefficietn of kinetic friction between the two.
View Solution
