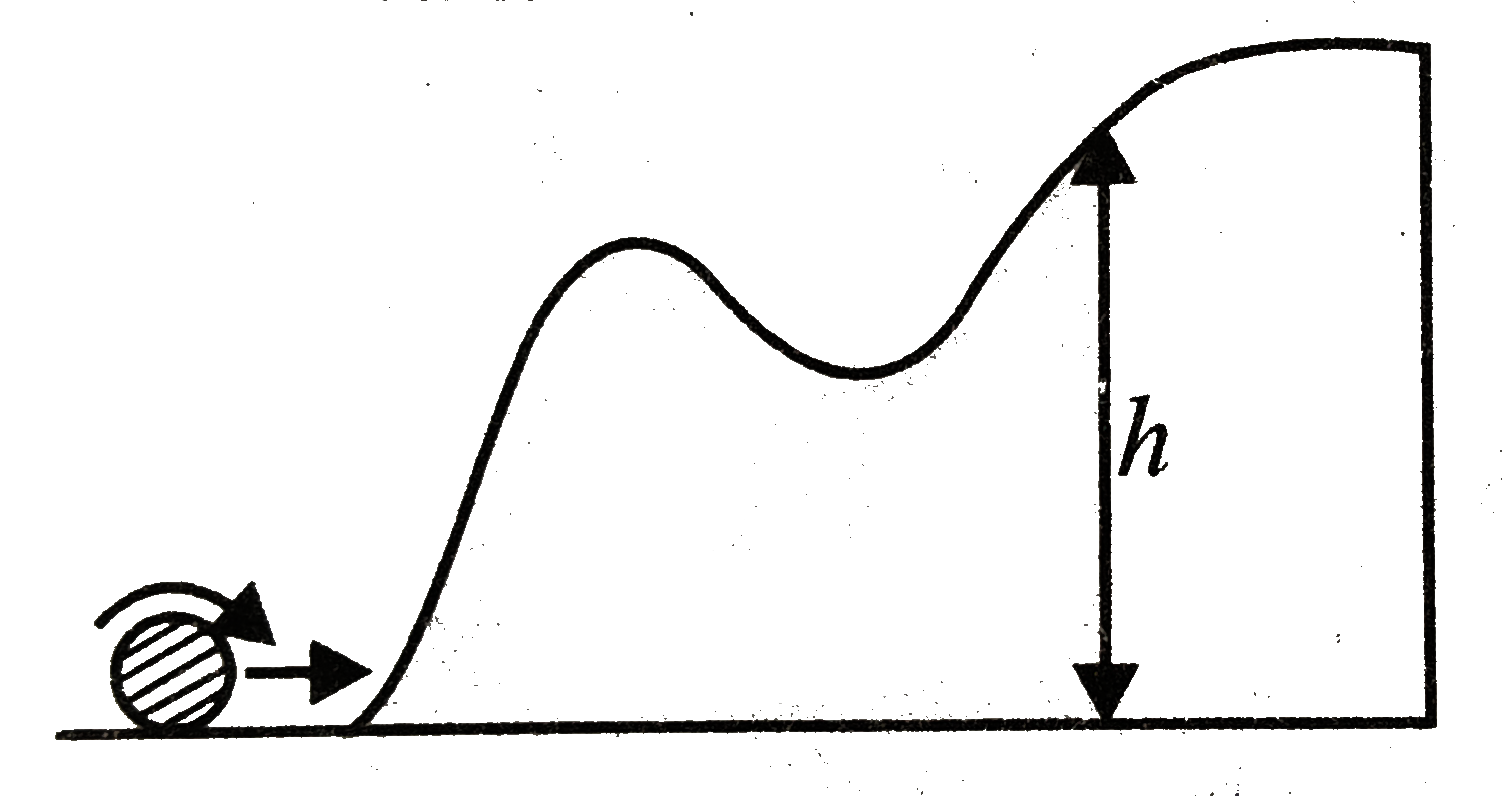
A body of radius R and mass m is rolling smoothly with speed v on a horizontal surface. It then rolls up a hill to a maximum height h. If h=3v2/4g. What might the body be ?
solid sphere
hollow sphere
disc
ring
The correct Answer is:C
To solve the problem, we will use the principle of conservation of energy. The kinetic energy of the rolling body will be converted into potential energy as it rolls up the hill.
1. Identify the given information:
- Mass of the body = m
- Radius of the body = R
- Initial speed of the body = v
- Maximum height reached = h=3v24g
2. Calculate the potential energy at maximum height:
The potential energy (PE) when the body reaches height h is given by:
PE=mgh=mg(3v24g)=3mv24
3. Calculate the initial kinetic energy:
The total kinetic energy (KE) of the rolling body consists of translational and rotational kinetic energy. The translational kinetic energy is:
KEtrans=12mv2
The rotational kinetic energy is given by:
KErot=12Iω2
where I is the moment of inertia and ω is the angular velocity. Since the body is rolling without slipping, we have:
ω=vR
Therefore, substituting ω:
KErot=12I(vR)2=12Iv2R2
4. Using conservation of energy:
The total initial kinetic energy equals the potential energy at the maximum height:
KEtrans+KErot=PE
Substituting the expressions we have:
12mv2+12Iv2R2=3mv24
5. Rearranging the equation:
Factor out v22:
v22(m+IR2)=3mv24
Dividing both sides by v2 (assuming v≠0):
12(m+IR2)=3m4
6. Solving for the moment of inertia I:
Multiply both sides by 2:
m+IR2=3m2
Rearranging gives:
IR2=3m2−m=m2
Therefore,
I=mR22
7. Identifying the body:
The moment of inertia I=mR22 is characteristic of a solid disc. Thus, the body is a disc.
Final Answer:
The body might be a disc.
- Mass of the body = m
- Radius of the body = R
- Initial speed of the body = v
- Maximum height reached = h=3v24g
The potential energy (PE) when the body reaches height h is given by:
PE=mgh=mg(3v24g)=3mv24
The total kinetic energy (KE) of the rolling body consists of translational and rotational kinetic energy. The translational kinetic energy is:
KEtrans=12mv2
The rotational kinetic energy is given by:
KErot=12Iω2
where I is the moment of inertia and ω is the angular velocity. Since the body is rolling without slipping, we have:
ω=vR
Therefore, substituting ω:
KErot=12I(vR)2=12Iv2R2
The total initial kinetic energy equals the potential energy at the maximum height:
KEtrans+KErot=PE
Substituting the expressions we have:
12mv2+12Iv2R2=3mv24
Factor out v22:
v22(m+IR2)=3mv24
Dividing both sides by v2 (assuming v≠0):
12(m+IR2)=3m4
Multiply both sides by 2:
m+IR2=3m2
Rearranging gives:
IR2=3m2−m=m2
Therefore,
I=mR22
The moment of inertia I=mR22 is characteristic of a solid disc. Thus, the body is a disc.
The body might be a disc.
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - A Only One Option is Correct
86 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - More than one option is correct
36 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
Similar Questions
An object of radius R and mass M is rolling horizontally without slipping with speed v . It then rolls up the hill to a maximum height h=3v24g . The moment of inertia of the object is ( g = acceleration due to gravity)
View SolutionA mass m of radius r is rolling horizontally without any slip with a linear speed v. It then rolls up to a height given by 34v2g
View SolutionA body of mass M and radius r, rolling on a smooth horizontal floor with velocity v, rolls up an irregular inclined plane up to a vertical height (3v2/4g). Compute the moment of inertia of the body.
View SolutionA disc of mass M and radius R rolls on a horizontal surface and then rolls up an inclined plane as shown in the figure. If the velocity of the disc is v, the height to which the disc will rise will be:

View SolutionA uniform disc of mass m and radius r rolls without slipping along a horizontal surface and ramp, as shown above. The disk has an initial velocity of υ . What is the maximum height h to which the center of mass of the disc rises ?

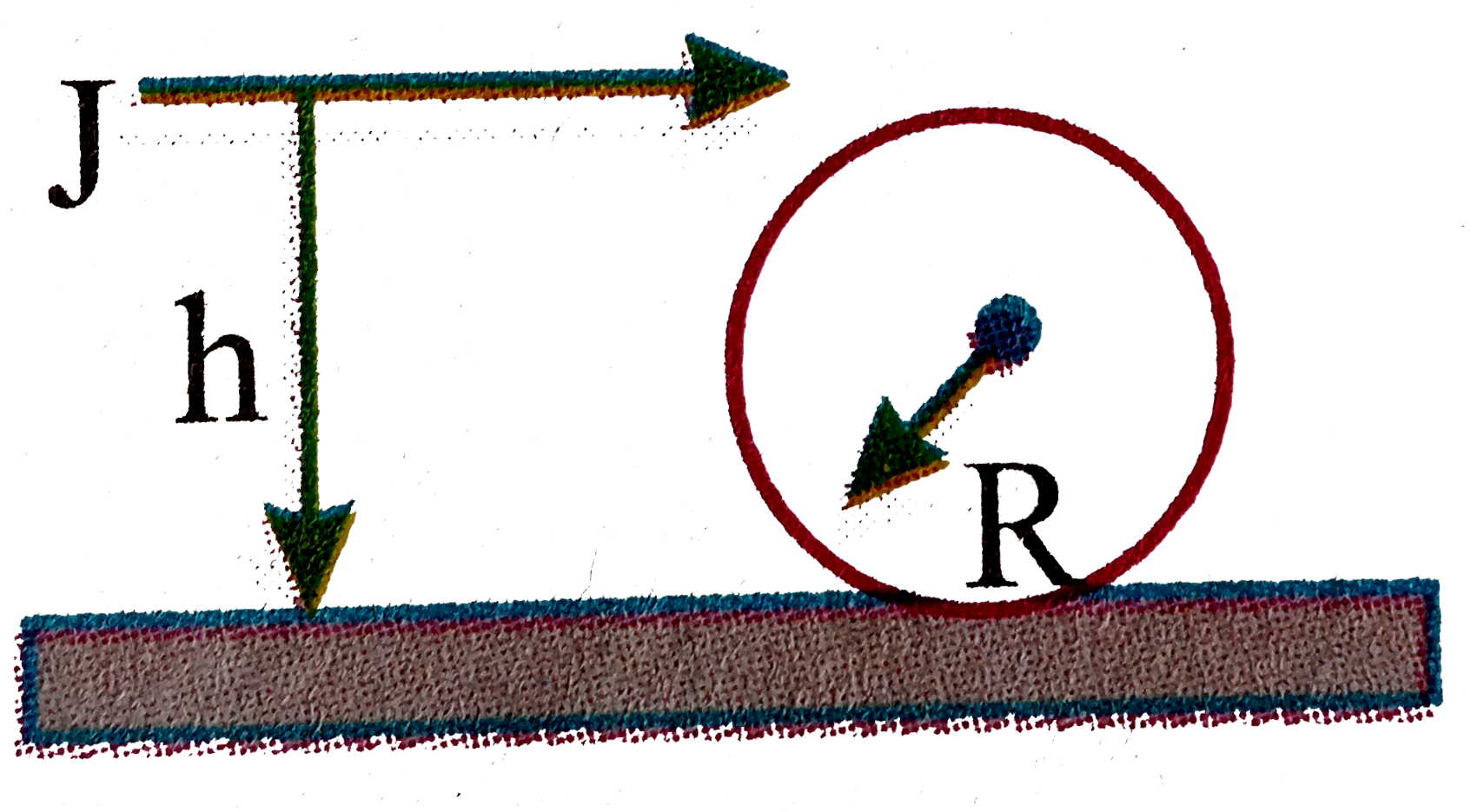
View SolutionA solid sphere of mass M and radius R is placed on a rough horizontal surface. It is stuck by a horizontal cue stick at a height h above the surface. The value of h so that the sphere performs pure rolling motion immediately after it has been stuck is
View SolutionA sphere of mass m and radius r rolls on a horizontal plane without slipping with a speed u. Now it rolls up vertically, then maximum height it would be attain will be
View SolutionA thin ring of mass m and radius R is in pure rolling over a horizontal surface. If v0 is the velocity of the centre of mass of the ring, then the angular momentum of the ring about the point of contact is
View SolutionA uniform circular disc of mass M and radius R rolls without slipping on a horizontal surface. If the velocity of its centre is v0, then the total angular momentum of the disc about a fixed point P at a height (3R)/2 above the centre C.

View SolutionA cylinder rolls on a horizontal plane surface. If the speed of the centre is 25 m/s, what is the speed of the highest point?
View Solution
