A cat is at rest on a horizontal turn table mounted on a vertical axis. If the cat begins to walk around the perimeter, what happens to the turn table ? Explain.
To solve the problem step by step, we will analyze the situation of the cat walking on the turntable and the resulting effects on the turntable itself.
1. Understanding the System:
- We have a cat sitting on a horizontal turntable that is mounted on a vertical axis. Initially, both the cat and the turntable are at rest.
2. Identifying the Forces:
- When the cat begins to walk around the perimeter of the turntable, it exerts a force on the turntable through its feet. This force is an internal force within the system (cat + turntable).
3. Conservation of Angular Momentum:
- Since there are no external forces acting on the system (cat + turntable), the total angular momentum of the system must be conserved. Initially, the angular momentum is zero because both the cat and the turntable are at rest.
4. Defining Angular Momentum:
- Let the mass of the cat be m, the radius of the turntable be r, and the angular velocity of the turntable be ω when the cat starts walking. The moment of inertia of the turntable can be denoted as I.
5. Setting Up the Equation:
- The total angular momentum of the system can be expressed as:
Ltotal=Lcat+Lturntable=0
- The angular momentum of the cat when it moves with velocity v is given by mvr (where v is the tangential speed of the cat).
- The angular momentum of the turntable is given by Iω.
6. Equating Angular Momenta:
- Since the total angular momentum must remain zero:
mvr+Iω=0
- Rearranging gives:
mvr=−Iω
- This indicates that if the cat moves in one direction, the turntable must rotate in the opposite direction to conserve angular momentum.
7. Conclusion:
- As the cat walks around the perimeter of the turntable, the turntable will start to rotate in the opposite direction to that of the cat's movement. This is a direct consequence of the conservation of angular momentum.
- We have a cat sitting on a horizontal turntable that is mounted on a vertical axis. Initially, both the cat and the turntable are at rest.
- When the cat begins to walk around the perimeter of the turntable, it exerts a force on the turntable through its feet. This force is an internal force within the system (cat + turntable).
- Since there are no external forces acting on the system (cat + turntable), the total angular momentum of the system must be conserved. Initially, the angular momentum is zero because both the cat and the turntable are at rest.
- Let the mass of the cat be m, the radius of the turntable be r, and the angular velocity of the turntable be ω when the cat starts walking. The moment of inertia of the turntable can be denoted as I.
- The total angular momentum of the system can be expressed as:
Ltotal=Lcat+Lturntable=0
- The angular momentum of the cat when it moves with velocity v is given by mvr (where v is the tangential speed of the cat).
- The angular momentum of the turntable is given by Iω.
- Since the total angular momentum must remain zero:
mvr+Iω=0
- Rearranging gives:
mvr=−Iω
- This indicates that if the cat moves in one direction, the turntable must rotate in the opposite direction to conserve angular momentum.
- As the cat walks around the perimeter of the turntable, the turntable will start to rotate in the opposite direction to that of the cat's movement. This is a direct consequence of the conservation of angular momentum.
Topper's Solved these Questions
- CIRCULAR MOTION Book:ICSEChapter:CIRCULAR MOTION Exercise:MODULE 2 (VERY SHORT ANSWER QUESTIONS)
Explore 14 Videos
- CIRCULAR MOTION Book:ICSEChapter:CIRCULAR MOTION Exercise:MODULE 2 (FROM EQUATIONS OF ROTATIONAL MOTION)
Explore 8 Videos
- CIRCULAR MOTION Book:ICSEChapter:CIRCULAR MOTION Exercise:MODULE 2 (LONG ANSWER QUESTIONS)
Explore 14 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:OBJECTIVE QUESTIONS FROM PREVIOUS IAS EXAMINATIONS
Explore 50 Videos
Similar Questions
A uniform rod AB of mass 3m and length 2l is lying at rest on a smooth horizontal table with a smooth vertical axis through the end A. A particle of mass 2m moves with speed 2u across the table and strikes the rod at its mid-point C if the impact is perfectly elastic. Find the speed of the particle after impact if
(a). It strikes rod normally,
(b). Its path before impact was inclinded at 60∘ to AC.
View SolutionA block of mass m kept on rough horizontal turn table at a distance x from the centre. If the coefficient of friction between the table and block is μ then maximum speed of point P on turn table so that the block does not slip is

View Solution
Knowledge Check
A small objective placed on a rotating horizontal turn table just slips when it is placed at a distance 4cm from the axis of rotation. If the angular velocity of the trun-table doubled, the objective slip when its distance from the axis of ratation is.
A1cmB2cmC4cmD8cm
A block of mass m is kept on horizontal turn table at x distance from the centre. If coefficient of friction between block and surface of turn table is μ, then maximum angular speed of the table so that block does not slip
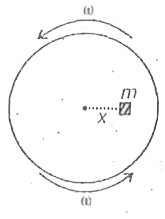
View SolutionA small objective placed on a rotating horizontal turn table just slips when it is placed at a distance 4cm from the axis of rotation. If the angular velocity of the trun-table doubled, the objective slip when its distance from the axis of ratation is.
View SolutionA mass m rests on a horizontal table. It is attached to a light inextensible string which passes over a smooth pulley and carries a mass m at the other end. If the mass m is raised vertically through a distance h and is then dropped, what is the speed with which the mass 2m begins to rise?

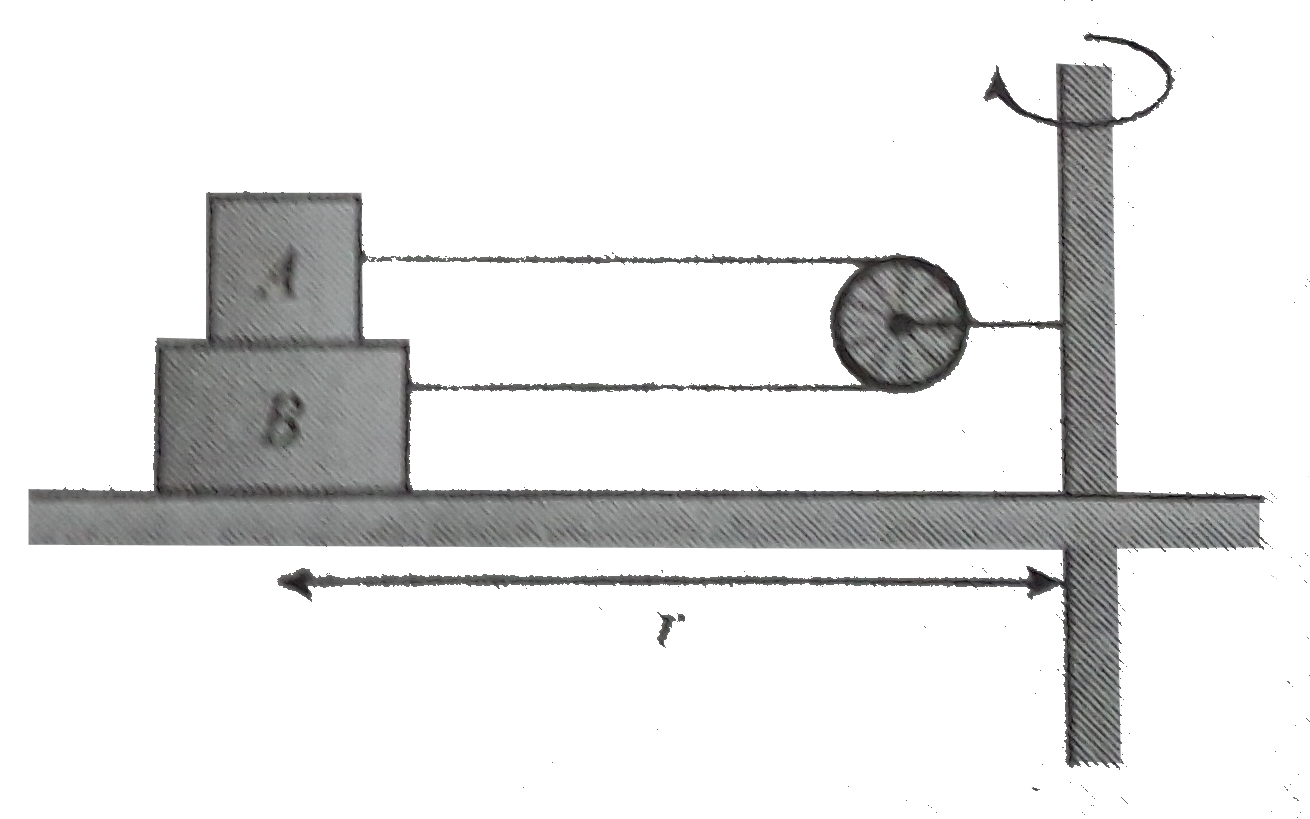
View SolutionIn fig two block of mass mAandmB are rotating at radius r kept on a rough table consider friction (μ) between all the contact surface pulley is frictionless determine the angular speed of the turn table for which the blocks just begin to slide
View SolutionA uniform cube of side a and mass m rests on a rough horizontal table. A horizontal force F is applied normal to one of the faces at a point directly above the centre of the face, at a height 3a4 above the base. What is the minimum value of F for which the cube begins to tip about an edge?
View SolutionA chain of mass m and length L is held at rest on smooth horizontal surface such that a part I of the chain is hanging vertically from the table. If the chain is let go, what is its speed as its end just leaves the horizontal surface?
View SolutionA uniform cube of side 'a' and mass m rests on a rough horizontal table. A horizontal force F is applied normal to one of the faces at a point directly below the centre of the face, at a height a/4 above the base.
a. What is the minimum value of F for which the cube begins to tip about an edge?
b. What is the minimum value of its so that toppling occurs?
c. If μ=μmin find minimum force for topping.
d. Find minimum μs so that Fmin can cause toppling.
View Solution