A circuit with capacitance and inductance generates free damped oscillations with current varying with time as . Find the voltage across the capacitor as a function of time, and in particular, at the moment .
We write
(gm means imaginary part )
Then
( An arbitrary constant of integration has been put equal to zero. )
Thus
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves
39 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
Knowledge Check
Figure shows the variation of voltage with time for an ac I=I0sinωt flowing through a circuit -
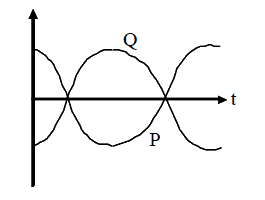 ACurve P is for R–L and Q for R–C circuitBCurve P is for R–C and Q for R–L circuitCBoth are for R–C circuitDBoth are for R–L circuit
ACurve P is for R–L and Q for R–C circuitBCurve P is for R–C and Q for R–L circuitCBoth are for R–C circuitDBoth are for R–L circuit
If the current in the ineer loop changes according to i=2t2 then, find the current in the capacitor as a function of time.
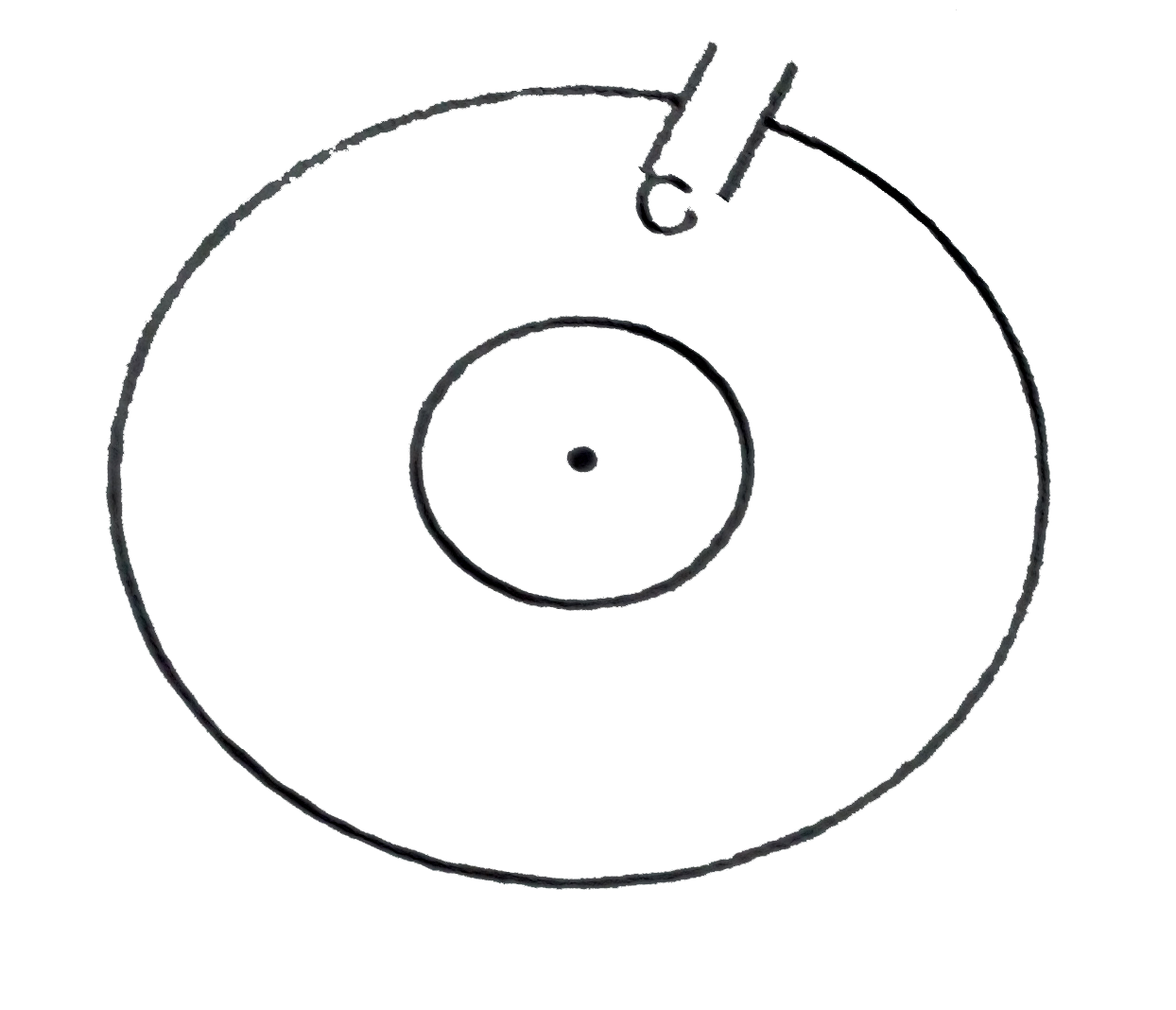
View SolutionIf the current in the inner loop changes according to I=2t2 (Fig .), then find the current in the capacitor as a function of time.

View SolutionAn oscillating circuit consisting of a capacitor with capacitance C and a coil of inductance L maintans free undamped oscillations with voltage amplitude across the capacitor equal to Vm. For an arbitrary moment of time find the relation between the current I in the circuit and the voltage V acroos the capacitor. Solve this problem usdini Ω's law and then the energy conservation law.
View SolutionA circuit consisting of a capacitor with capacitance C and a resistance R connected in series was connected at the moment t=0 to a source of ac voltage V=Vmcosωt . Find the current in the circuit as a function of time t.
View SolutionA point performs dampled oscillations according to the law x=a0e−βtsinωt. Find :
(a) the oscillation amplitude and the velocity of the point at the moment t=0,
(b) the moments of time at which the point reaches the extreme positions.
View SolutionFind the quality factor of a circuit with capacitance C=2.0μF and inductance L=5.0mH if the maintenance of undamped oscillations in the circuit with the voltage amplitude across the capacitor being equal to Vm=1.0V requires a power (:P:)=0.10mW. The damping of oscillations is sufficiently low
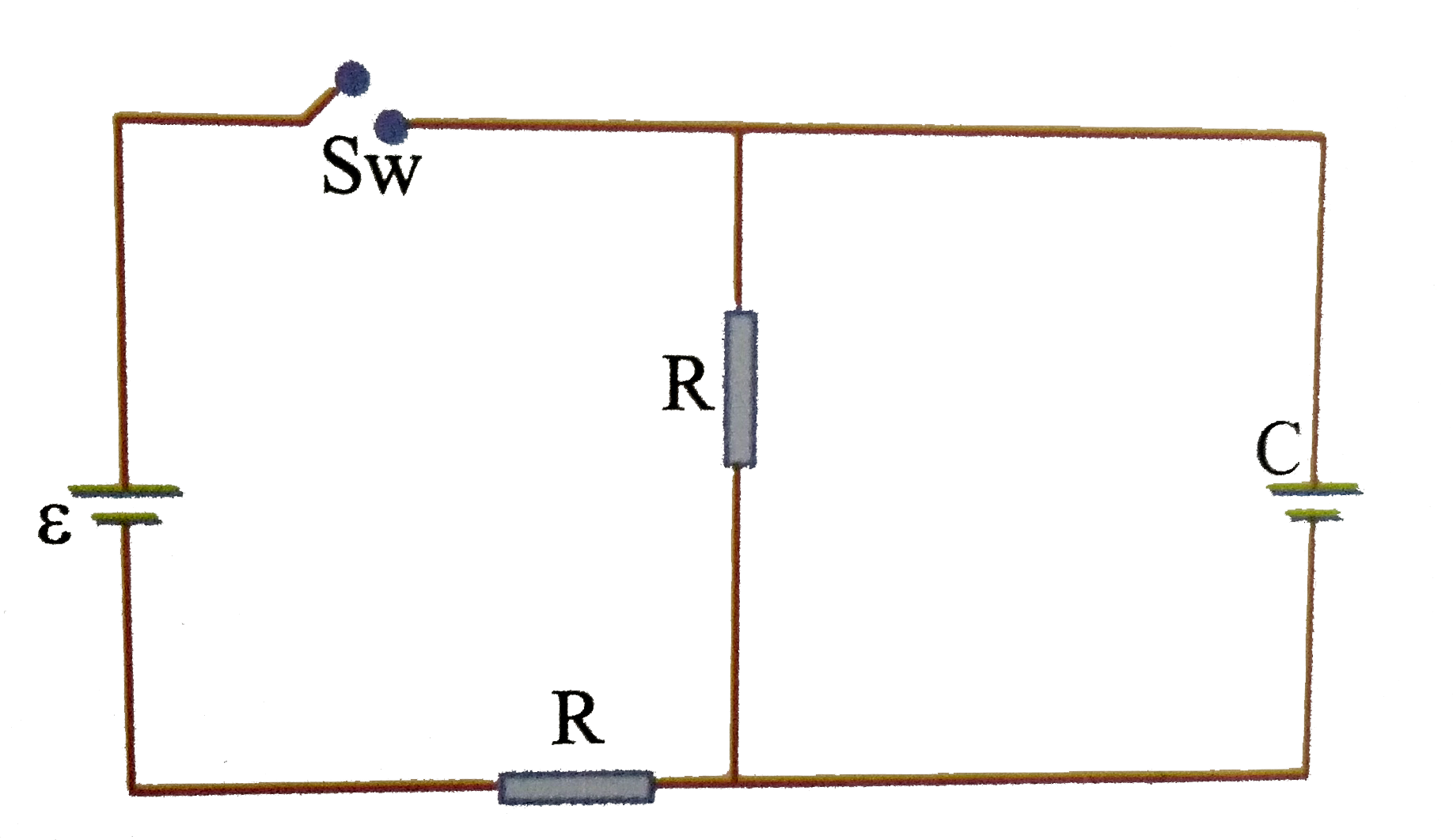
View SolutionA circuit consists of a capacitor with capacitance C and a coil of inductance L connected in series, as well as a switch and a resistance equal to the critical value for this circuit. With the switch disconnected, the capacitor was charged to a voltage V0, and at the moment t=0, the switch was closed . Find the corrent I in the circuit as a function of time t.What is Imax equal to ?
View Solution

 .
.