A cubical block of wood of edge a and density ρ floats in water of density 2ρ. The lower surface of the cube just touches the free end of a mass less spring of force constant K fixed at the bottom of the vessel. The weight W put over the block so that it is completely immersed in water without wetting the weight is
a(a2ρg+k)
a(aρg+2k)
a(aρg2+2k)
a(a2λg+k2)
The correct Answer is:D
To solve the problem step by step, we will analyze the forces acting on the cubical block of wood when it is completely immersed in water.
Step 1: Understand the Problem
We have a cubical block of wood with edge length a and density ρ floating in water with density 2ρ. The block is completely immersed in water, and we need to find the weight W that is placed on the block to achieve this condition.
Step 2: Calculate the Volume and Weight of the Block
The volume V of the cubical block can be calculated as:
V=a3
The weight of the block Wblock can be calculated using its density:
Wblock=density×volume×g=ρ⋅a3⋅g
Step 3: Calculate the Buoyant Force
The buoyant force Fb acting on the block when it is fully immersed in water can be calculated using Archimedes' principle:
Fb=density of water×volume of displaced water×g
Since the block is fully immersed, the volume of displaced water is equal to the volume of the block:
Fb=2ρ⋅a3⋅g
Step 4: Set Up the Force Balance Equation
When the block is fully immersed, the upward buoyant force must balance the downward forces, which include the weight of the block and the additional weight W:
Fb=Wblock+W
Substituting the expressions we found:
2ρ⋅a3⋅g=ρ⋅a3⋅g+W
Step 5: Solve for the Weight W
Rearranging the equation to solve for W:
W=2ρ⋅a3⋅g−ρ⋅a3⋅g
W=ρ⋅a3⋅g
Step 6: Include the Spring Force
If we consider the spring force Fs when the block is pressed down by the weight W, we can express the spring force as:
Fs=K⋅x
where x is the compression of the spring.
Step 7: Final Expression for Weight W
The total downward force when the spring is compressed will be:
W+Wblock=Fb+Fs
Substituting the values we have:
W+ρ⋅a3⋅g=2ρ⋅a3⋅g+K⋅x
From this, we can derive the final expression for W:
W=2ρ⋅a3⋅g−ρ⋅a3⋅g−K⋅x
This leads to the final expression for W in terms of a, ρ, g, and K.
Conclusion
The weight W that must be placed on the block to keep it fully immersed in water without wetting the weight is given by:
W=ρ⋅a3⋅g+K⋅x
We have a cubical block of wood with edge length a and density ρ floating in water with density 2ρ. The block is completely immersed in water, and we need to find the weight W that is placed on the block to achieve this condition.
The volume V of the cubical block can be calculated as:
V=a3
The weight of the block Wblock can be calculated using its density:
Wblock=density×volume×g=ρ⋅a3⋅g
The buoyant force Fb acting on the block when it is fully immersed in water can be calculated using Archimedes' principle:
Fb=density of water×volume of displaced water×g
Since the block is fully immersed, the volume of displaced water is equal to the volume of the block:
Fb=2ρ⋅a3⋅g
When the block is fully immersed, the upward buoyant force must balance the downward forces, which include the weight of the block and the additional weight W:
Fb=Wblock+W
Substituting the expressions we found:
2ρ⋅a3⋅g=ρ⋅a3⋅g+W
Rearranging the equation to solve for W:
W=2ρ⋅a3⋅g−ρ⋅a3⋅g
W=ρ⋅a3⋅g
If we consider the spring force Fs when the block is pressed down by the weight W, we can express the spring force as:
Fs=K⋅x
where x is the compression of the spring.
The total downward force when the spring is compressed will be:
W+Wblock=Fb+Fs
Substituting the values we have:
W+ρ⋅a3⋅g=2ρ⋅a3⋅g+K⋅x
From this, we can derive the final expression for W:
W=2ρ⋅a3⋅g−ρ⋅a3⋅g−K⋅x
This leads to the final expression for W in terms of a, ρ, g, and K.
The weight W that must be placed on the block to keep it fully immersed in water without wetting the weight is given by:
W=ρ⋅a3⋅g+K⋅x
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct
21 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Comprehension
32 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
A cubical block 'A' of mass m0(=a3ρ=3kg) of edge 'a' and density ρ floats in a liquid of density 3ρ. The lower surface of the cube just touches the free end of massless spring of spring of spring constant k(=a2ρg) fixed at the bottom of the vessel. Another block 'B' of mass 'm' is lept over block 'A', so that is completely immersed in liquid without wetting the block 'B'. Then find the value of m in kg.
View SolutionA cubical block of wood of edge 3 cm floats in water. The lower surface of the cube just touches the free end of a vertical spring fixed at the bottom of the pot. Find the maximum weight that can be put on the block without wetting it. Density of wood =800kgm−3 and spring constant of the spring =50Nm−1Takeg=10ms−2.
 ,
,
View SolutionA cubical block of wood of edge 3 cm floats in water. The lower surface of the cube just touches the free end of a vertical spring fixed at the bottom of the pot. Find the maximum weight that can be put on the block without wetting it. Density of wood =800kgm−3 and spring constant of the spring =50Nm−1Takeg=10ms−2.
 ,
,
View SolutionA wooden ball of mass m (density σ) is kept inside water (density ρ>σ) by the help of a masseless string shown in the figure. One end of the string is fixed at the bottom of vessel. When the vessel containing the water is accelerated upwards with acceleration a as shown in the figure.

View SolutionA cubic block of side a is connected with two similar vertical springs as shown. Initially, bottom surface of the block of density σ touches the surface of the fluid of density 2σ while floating. A weight is placed on the block so that it is immersed half in the fluid, find the weight.

View SolutionA wooden block of mass 8 kg is tied to a string attached to the bottome of the tank. In the equilibrium the block is completely immersed in water. If relative density of wood is 0.8 and g=10ms−2, the tension T, in the string is
View SolutionA wooden block of mass 0.5 kg and density 800 kgm−3 is fastened to the free end of a vertical spring of spring constant 50 Nm−1 fixed at the bottom. If the entire system is completely immersed in water, find a. the elongation (or compression) of the spring in equilibrium and b. the time period of vertical oscillations of the block when it is slightly depressed and released.
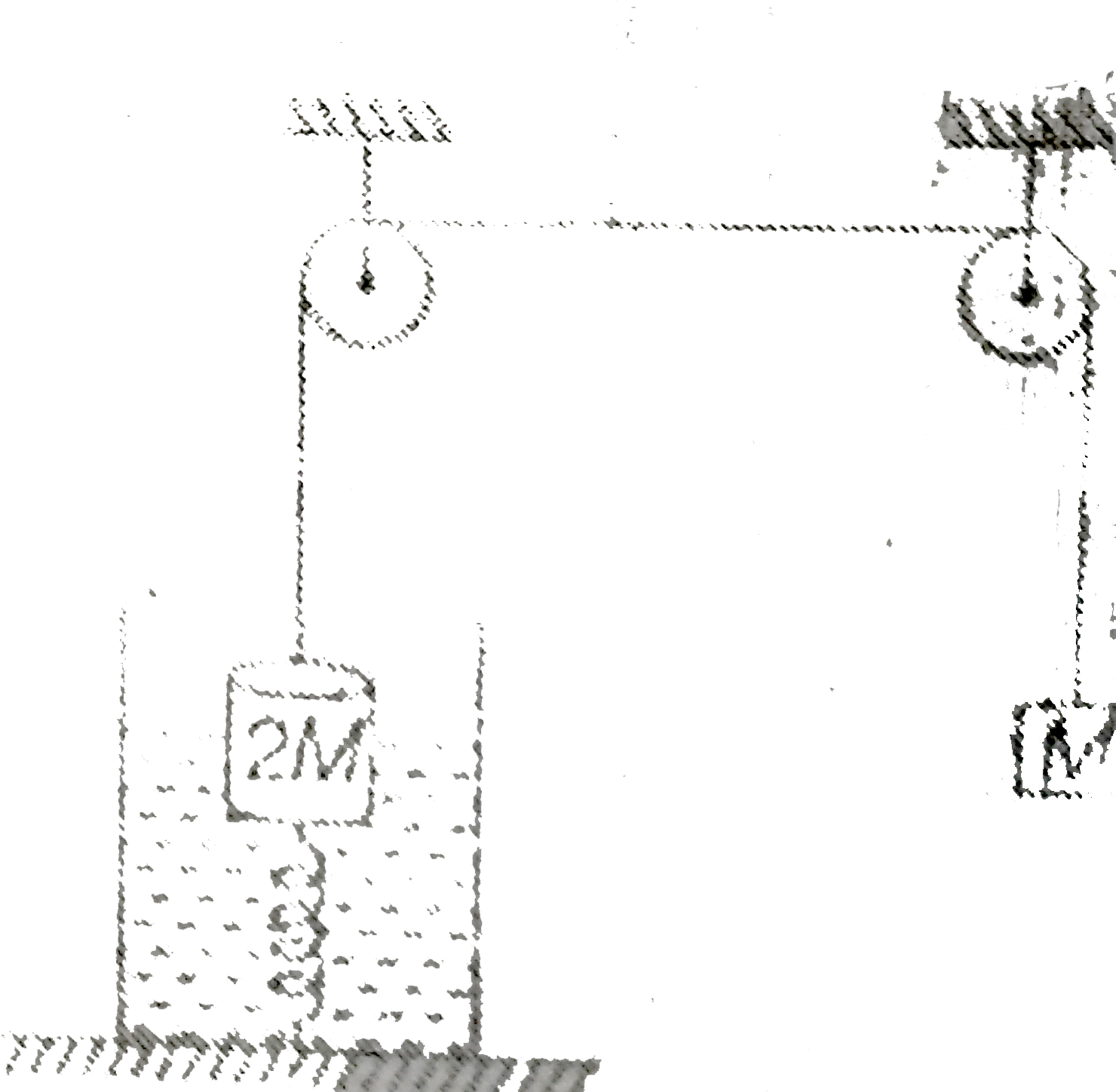
View SolutionPassage XIV) A uniform cylindrical block of mass 2M and cross-sectional area A remains partially submerged in a non viscous liquid of density ρ, density of the material of the cylinder is 3ρ. The cylinder is connected to lower end of the tank by means of a light spring of spring constant K. The other end of the cylinder is connected to anotehr block of mass M by means of a light inextensible sting as shown in the figure. The pulleys shown are massless and frictionless and assume that the cross-section of the cylinder is very small in comparison to that of the tank. Under equilibrium conditions, half of the cylinder is submerged. [given that cylinder always remains partially immersed)
Under equilibrium conditions
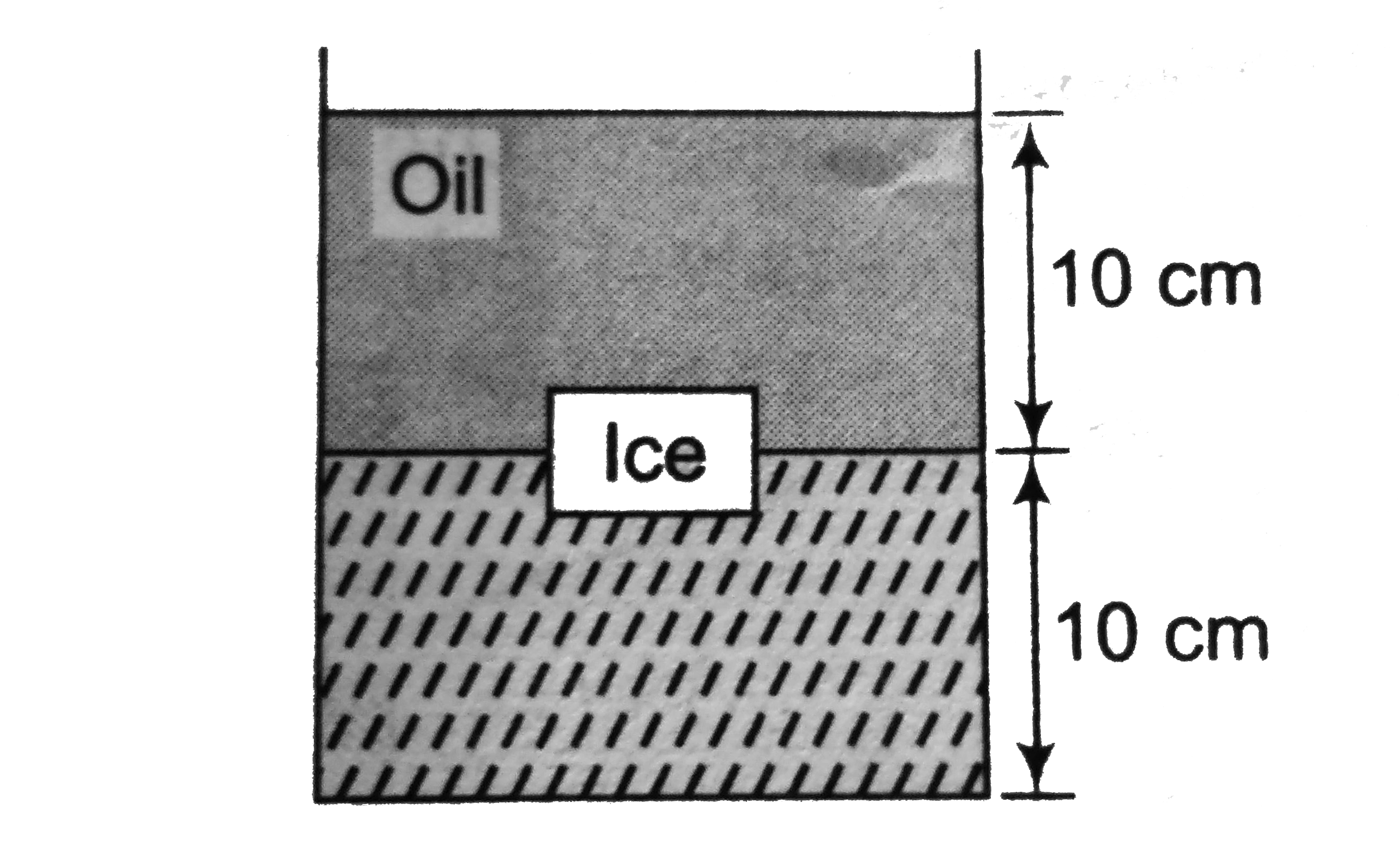
View SolutionA cubical block of side 10cm floats at the interface of an oil and water as shown in the figure. The density of oil is 0.6gcm−3 and the lower face of ice cube is 2cm below the interface. The pressure above that of the atomosphere at the lower face of the block is
View SolutionPassage XIV) A uniform cylindrical block of mass 2M and cross-sectional area A remains partially submerged in a non viscous liquid of density ρ, density of the material of the cylinder is 3ρ. The cylinder is connected to lower end of the tank by means of a light spring of spring constant K. The other end of the cylinder is connected to anotehr block of mass M by means of a light inextensible sting as shown in the figure. The pulleys shown are massless and frictionless and assume that the cross-section of the cylinder is very small in comparison to that of the tank. Under equilibrium conditions, half of the cylinder is submerged. [given that cylinder always remains partially immersed)

By what maximum distance cylinder will be pushed downward into the liquid from equilibrium position so that when it is set free then tension in the string will not vanish [Assume at equilibrium position system was at rest]
View Solution
