A cylindrical conductor of radius 'R' carries a current 'i'. The value of magnetic field at a point which is R/4 distance inside from the surface is 10T. Find the value of magnetic field at point which is 4R distance outside from the surface
43T
83T
403T
803T
The correct Answer is:B
To solve the problem step by step, we will use the formulas for the magnetic field inside and outside a cylindrical conductor.
Step 1: Understand the problem
We have a cylindrical conductor of radius R carrying a current i. We need to find the magnetic field at a point that is 4R distance outside from the surface of the conductor, given that the magnetic field at a point R4 distance inside from the surface is 10T.
Step 2: Define the distance for the inside point
The distance from the center of the cylinder to the point inside the conductor is:
r=R−R4=3R4
Step 3: Use the formula for the magnetic field inside the cylindrical conductor
The magnetic field B inside a cylindrical conductor is given by the formula:
Binside=μ04π⋅2irR2
Substituting r=3R4:
Binside=μ04π⋅2i⋅3R4R2
This simplifies to:
Binside=μ0⋅3i8πR
Step 4: Set the inside magnetic field equal to the given value
We know from the problem that:
Binside=10T
Thus, we can set up the equation:
μ0⋅3i8πR=10
Step 5: Use the formula for the magnetic field outside the cylindrical conductor
The magnetic field B outside a cylindrical conductor is given by:
Boutside=μ04π⋅2ir
For a point 4R outside the surface, the distance r from the center of the cylinder is:
r=R+4R=5R
Thus, we can write:
Boutside=μ04π⋅2i5R
Step 6: Relate the two magnetic fields
Now we can relate the two magnetic fields using the equations we derived:
BinsideBoutside=(R−R4)(R+4R)R2
Substituting the values:
10Boutside=(3R4)(5R)R2
This simplifies to:
10Boutside=15R4R2=154R
Step 7: Solve for Boutside
Rearranging gives:
Boutside=10⋅4R15=40R15=8R3
Step 8: Conclusion
Thus, the magnetic field at a point which is 4R distance outside from the surface of the cylindrical conductor is:
Boutside=83T
We have a cylindrical conductor of radius R carrying a current i. We need to find the magnetic field at a point that is 4R distance outside from the surface of the conductor, given that the magnetic field at a point R4 distance inside from the surface is 10T.
The distance from the center of the cylinder to the point inside the conductor is:
r=R−R4=3R4
The magnetic field B inside a cylindrical conductor is given by the formula:
Binside=μ04π⋅2irR2
Substituting r=3R4:
Binside=μ04π⋅2i⋅3R4R2
This simplifies to:
Binside=μ0⋅3i8πR
We know from the problem that:
Binside=10T
Thus, we can set up the equation:
μ0⋅3i8πR=10
The magnetic field B outside a cylindrical conductor is given by:
Boutside=μ04π⋅2ir
For a point 4R outside the surface, the distance r from the center of the cylinder is:
r=R+4R=5R
Thus, we can write:
Boutside=μ04π⋅2i5R
Now we can relate the two magnetic fields using the equations we derived:
BinsideBoutside=(R−R4)(R+4R)R2
Substituting the values:
10Boutside=(3R4)(5R)R2
This simplifies to:
10Boutside=15R4R2=154R
Rearranging gives:
Boutside=10⋅4R15=40R15=8R3
Thus, the magnetic field at a point which is 4R distance outside from the surface of the cylindrical conductor is:
Boutside=83T
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - single correct Answer Type35videos
SOURCES OF MAGNETIC FIELD
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.612videos
RAY OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Comprehension Type14videos
WAVE OPTICS
Similar Questions
A long copper tube of inner radius R carriers a current i. The magnetic field B inside the tube is
View SolutionA circular coil of radius R carries a current i. The magnetic field at its centre is B. The distance from the centre on the axis of the coil where the magnetic field will be B/8 is
View SolutionA conductor is carrying a current i. the magnetic field intensity at a the point O which is the common centre of three arcs is
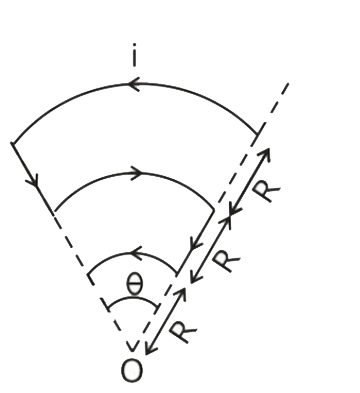 View Solution
View SolutionA cylindrical long wire of radius R carries a current I uniformly distributed over the cross sectional area of the wire. The magnetic field at a distance x from the surface inside the wire is
View SolutionA circular coil of radius 2R is carrying current 'i' . The ratio of magnetic fields at the centre of the coil and at a point at a distance 6R from the centre of the coil on the axis of the coil is
View SolutionA thin but long, hollow, cylindrical tube of radius r carries a current i along its length. Find the magnitude of the magnetic field at a distance r/2 from the surface (a) inside the tube (b) outside the tube.
View SolutionA cylinderical conductor of radius R is carrying constant current. The plot of the magnitude of the magnetic field, B with the distance, d from the centre of the conductor , is correctly represented by the figure:
View SolutionShown in Fig. is a conductor carrying current I. Find the magnetic field intensity at point O.
View SolutionA circular coil of wire of radius 'r' has 'n' turns and carries a current 'I'. The magnetic induction (B) at a point on the axis of the coil at a distance √3r from its centre is
View SolutionA long cylindrical conductor of radius R carries a current i as shown in the figure. The current density J varies across the cross-section as J=kr2, where, k is a constant. Find an expression for the magnetic field B at a distance r(<R) from the axis
 View Solution
View Solution

