A gum (mass=M) fires a bullet (mass=m) with speed vr relative to barrel of the gum which is inclined at an anlge of 60∘ with horizontal. The gun is placed over a smooth horizontal surface. Find the recoil speed of gun.
The correct Answer is:B
To solve the problem of finding the recoil speed of the gun when it fires a bullet, we can use the principle of conservation of momentum. Here’s a step-by-step solution:
Step 1: Identify the system
We have a gun of mass M and a bullet of mass m. The bullet is fired with a speed vr relative to the barrel of the gun, which is inclined at an angle of 60∘ with the horizontal.
Step 2: Set up the momentum conservation equation
Since the gun is placed on a smooth horizontal surface, there are no external horizontal forces acting on the system. Therefore, the total momentum before firing must equal the total momentum after firing.
Before firing:
- The total momentum is 0 because both the gun and the bullet are at rest.
After firing:
- The momentum of the bullet can be resolved into horizontal and vertical components. The horizontal component of the bullet's velocity is given by:
vbx=vrcos(60∘)=vr⋅12=vr2
- The momentum of the bullet is then m⋅vbx=m⋅vr2.
Let v be the recoil speed of the gun. The momentum of the gun is −M⋅v (the negative sign indicates that the gun moves in the opposite direction to the bullet).
Step 3: Write the conservation of momentum equation
According to the conservation of momentum:
Total momentum before firing=Total momentum after firing
This gives us:
0=−M⋅v+m⋅vr2
Step 4: Solve for the recoil speed v
Rearranging the equation gives:
M⋅v=m⋅vr2
Thus, we can solve for v:
v=m⋅vr2M=m⋅vr2M
Final Answer
The recoil speed of the gun is:
v=m⋅vr2M
We have a gun of mass M and a bullet of mass m. The bullet is fired with a speed vr relative to the barrel of the gun, which is inclined at an angle of 60∘ with the horizontal.
Since the gun is placed on a smooth horizontal surface, there are no external horizontal forces acting on the system. Therefore, the total momentum before firing must equal the total momentum after firing.
- The total momentum is 0 because both the gun and the bullet are at rest.
- The momentum of the bullet can be resolved into horizontal and vertical components. The horizontal component of the bullet's velocity is given by:
vbx=vrcos(60∘)=vr⋅12=vr2
- The momentum of the bullet is then m⋅vbx=m⋅vr2.
According to the conservation of momentum:
Total momentum before firing=Total momentum after firing
This gives us:
0=−M⋅v+m⋅vr2
Rearranging the equation gives:
M⋅v=m⋅vr2
Thus, we can solve for v:
v=m⋅vr2M=m⋅vr2M
The recoil speed of the gun is:
v=m⋅vr2M
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Type 11videos
CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Solved Examples13videos
CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions15videos
CENTRE OF MASS, IMPULSE AND MOMENTUM
- BOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery19videos
CIRCULAR MOTION
Similar Questions
A gun of mass M fires a bullet of mass m with a Kinetic energy E. The velocity of recoil of the gun is
View SolutionFrom the ground, a projectile is fired at an angle of 60 degree to the horizontal with a speed of 20 m s−1 The horizontal range of the projectile is
View SolutionA loaded spring gun of mass M fires a bullet of mass m with a velocity v at an angle of elevation θ. The gun is initially at rest on a horizontal smooth surface. After firing, the centre of mass of the gun and bullet system
View SolutionA loaded spring gun of mass M fires a bullet of mass m with a velocity v at an angle of elevation θ. The gun is initially at rest on a horizontal smooth surface. After firing, the centre of mass of the gun and bullet system
View SolutionA gun of mass M fires a bullet of mass m with a velocity v relative to the gun. The average force required to bring the gun to rest in 0.5 sec. is
View SolutionA block of mass m is placed at rest on a smooth wedge of mass M placed at rest on a smooth horizontal surface. As the system is released
 View Solution
View SolutionA gun fires a bullet. The barrel of the gun is inclined at an angle of 45∘ with horizontal. When the bullet leaves the barrel it will be travelling at an angle greater than 45∘ with the horizontal. Is this statement true or false?
View SolutionA gun fires a bullet. The barrel of the gun is inclined at an angle of 45∘ with horizontal. When the bullet leaves the barrel it will be travelling at an angle greater than 45∘ with the horizontal. Is this statement true or false?
View SolutionA gun of mass M fires a bullet of mass m, with a Kinetic energy E. The total kinetic energy in the firing process is
View SolutionIn the figure-2.61 a bar of mass m is on the smooth inclined face of the wedge of mass M,the inclination to the horizontal being 0.The wedge is resting on a smooth horizontal plane.Assuming the pulley to be smooth and the string is light and inextensible. Find the acceleration of M,when M and m are always in contact.
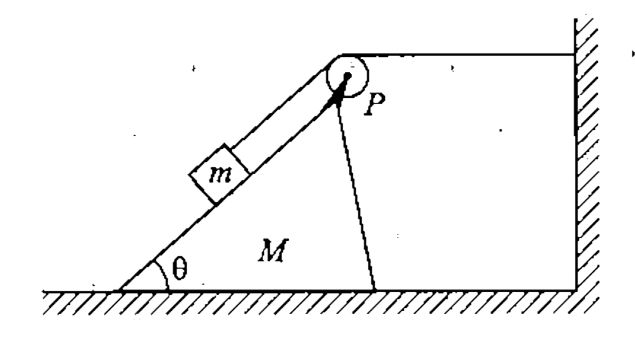 View Solution
View Solution
