(a) Obtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in Fig.
(b) Evaluate the induced emf in loop if the wire carries a current of 50 A and the loop has an instantaneous velocity υ=10ms−1 at the location x = 0.2 m, as shown. Take a = 0.1m and assume that the loop has a large resistance.
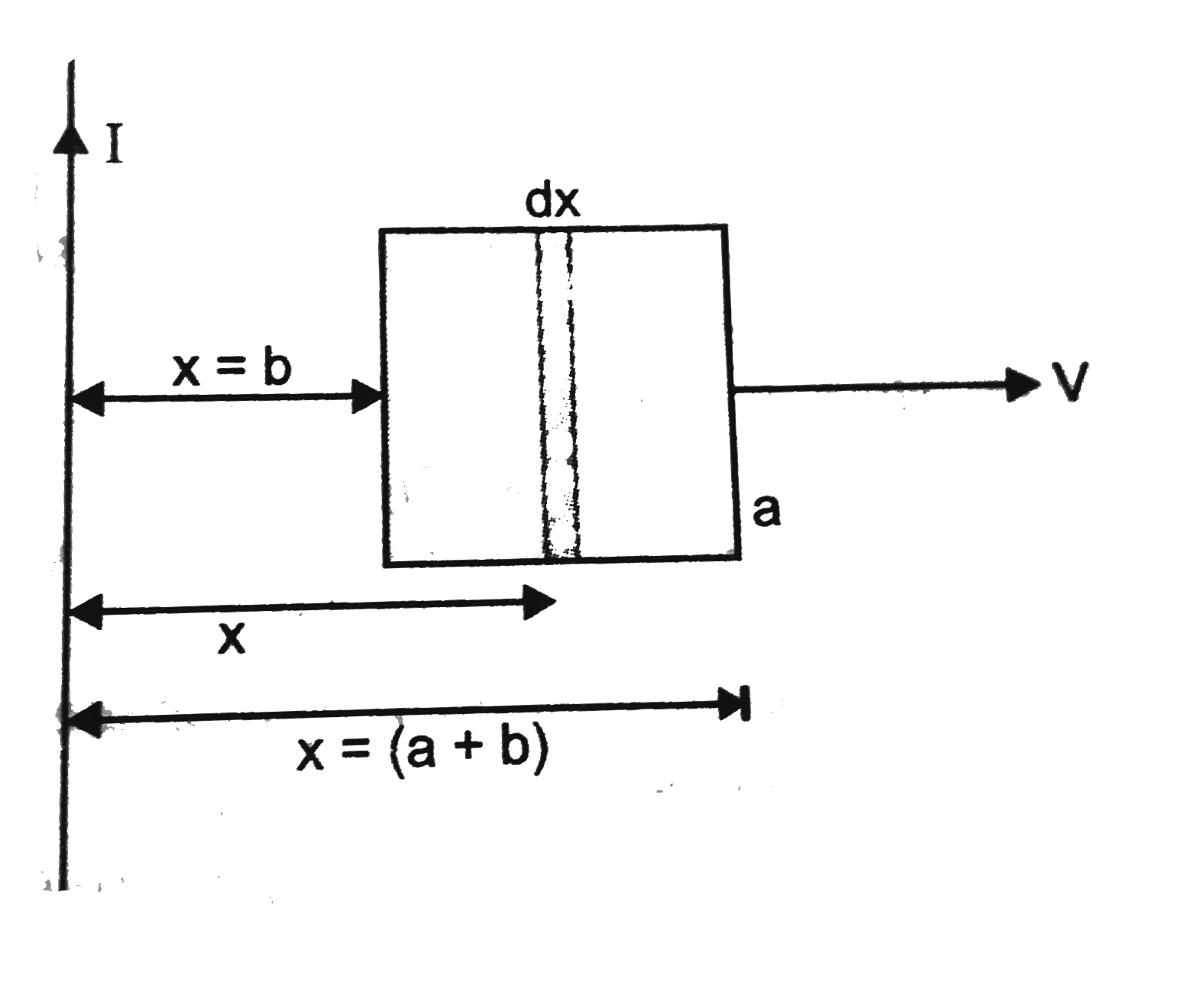
(a) Take a small element dy in the loop at a distance y from the long straight wire (as shown in the given figure).
Magnetic flux associated with element dy,dϕ=Bda
Where
dA = Area of element dy = a dy
B= magnetic field at distance y
=μ0I2πy
I= current in the wire
μ0 =Permeability of free space =4π×10−7TmA−1
∴dϕ=μ0Ia2πdyy
ϕ=μ0Ia2π∫dyy
y tends from x to a+x
∴ϕ=μ0Ia2πa+x∫xdyy
=μ0Ia2π[logey]a+xx
=μ0Ia2πloge(a+xx)
For mutyual inductance M, the flux is given as :
ϕ=MI
∴MI=μ0Ia2πloge(ax+1)
M=μ0a2πloge(ax+1)
(b) Emf induced in the loop, e=B'av=(μ0I2πx)av
Given
I=50 A
x=0.2 m
a=0.1 m
v=10 m/s
e=4π×10−7×50×0.1×102π×0.2
e=5×10−5V
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise
17 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise
34 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise
15 Videos
Similar Questions
Find the mutual inductance between the straight wire and the square loop of figure.
View Solution(a) Obtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in Fig. 6.06.
(b) Now assume that the straight wire carries a current of 50 A and the loop is moved to the right with a constant velocity, v = 10 m/s. Calculate the induced emf in the loop at the instant when x = 0.2 m. Take a = 0.1 m and assume that the loop has a large resistance.
View Solution
Knowledge Check
The mutual inductance between the rectangular loop and the long straight wire as shown in figure is M.
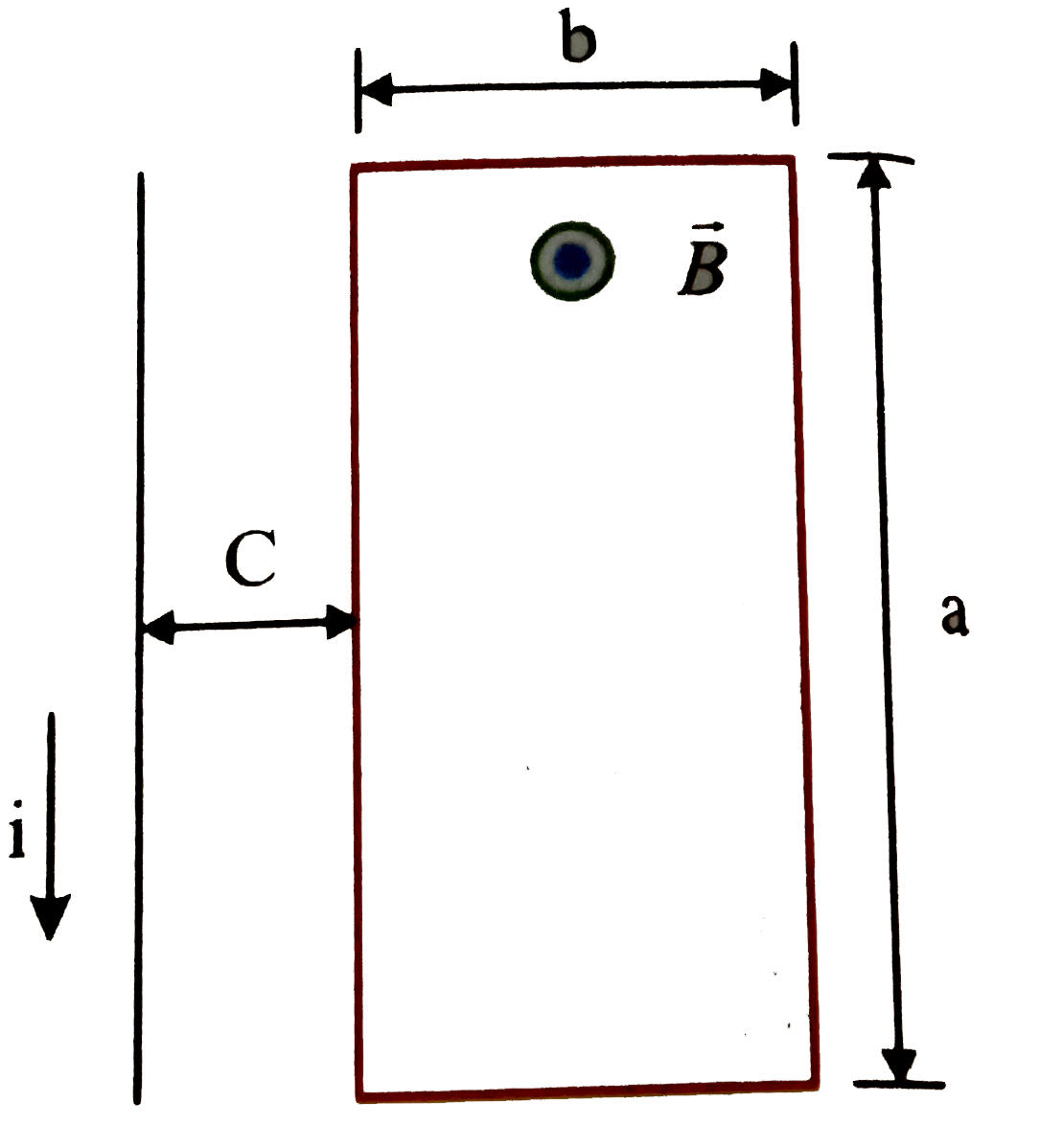 AM= zeroBM=μ0a2πln(1+cb)CM=μ0a2πln(a+bb)DM=μ0a2πln(1+bb)
AM= zeroBM=μ0a2πln(1+cb)CM=μ0a2πln(a+bb)DM=μ0a2πln(1+bb)The mutual inductance between the rectangular loop and the long straight wire as shown in figure is M.
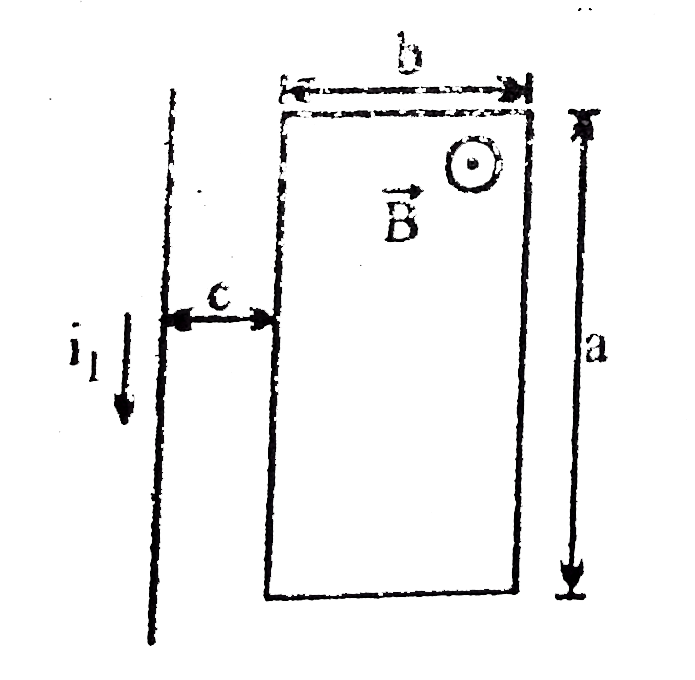 AM=ZeroBM=μ0a2πIn(1+cb)CM=μ0b2πIn(a+cb)DM=μ0a2πIn(1+bc)
AM=ZeroBM=μ0a2πIn(1+cb)CM=μ0b2πIn(a+cb)DM=μ0a2πIn(1+bc)A loop and an infinite wire are placed side by side as shown in the figure. If the current I in the wire is increased then the induced current in the loop is
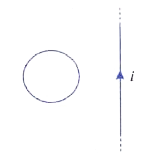 AclockwiseBanticlockwiseCzeroDcannot determine
AclockwiseBanticlockwiseCzeroDcannot determine
Find the mutual inductance of a straight long wire and a rectangular loop, as shown in the figure.
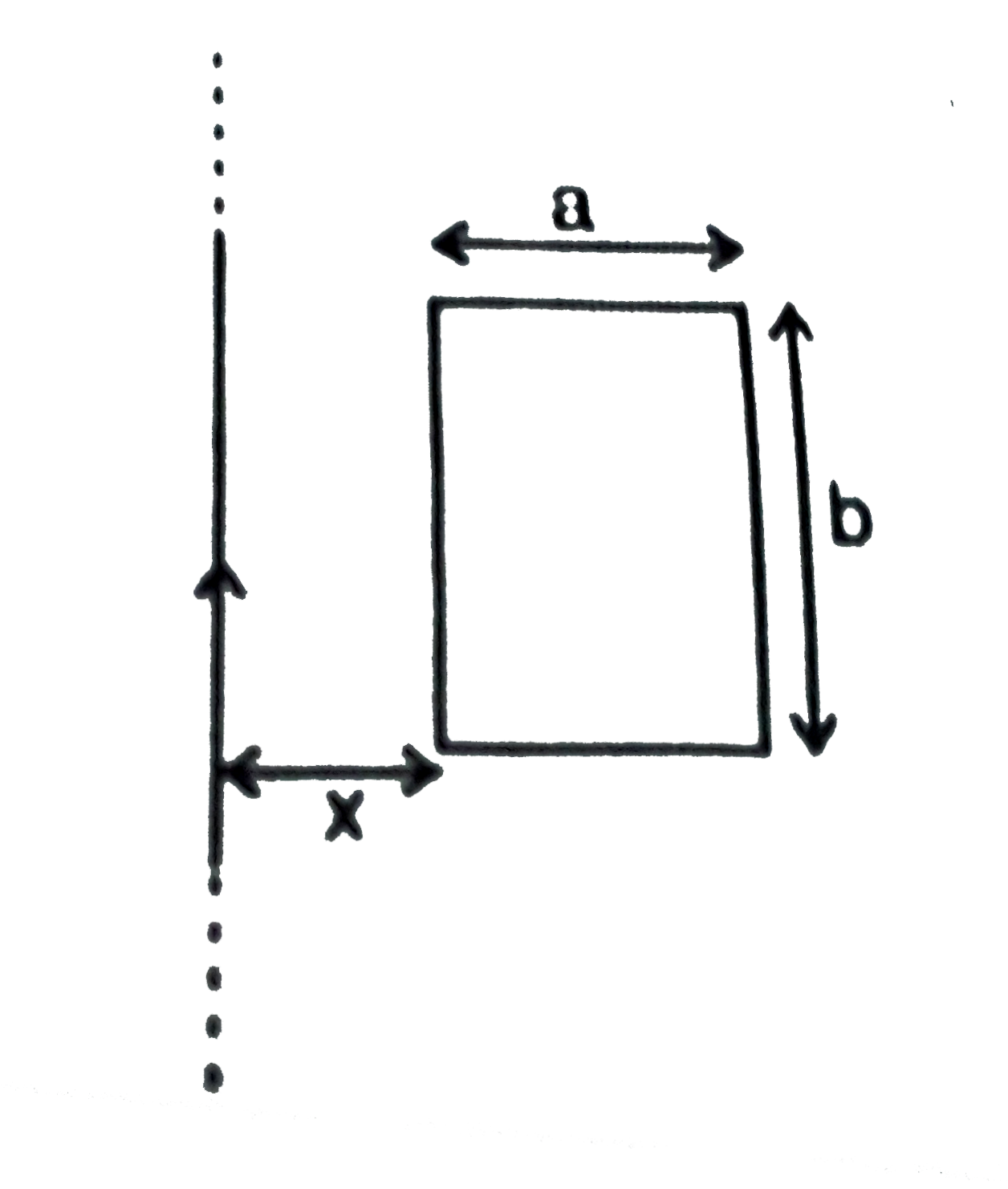
View SolutionFind the mutual inductance of a straight long wire and a rectangular loop, as shown in the figure.

View SolutionFind the mutual inductance of a straight long wire and a rectangular loop, as shown in the figure.

View SolutionShow a conduction loop placed near a long, straight wire carrying current i as shown. If the current increases continuously, find the direction of the induced current in the loop.
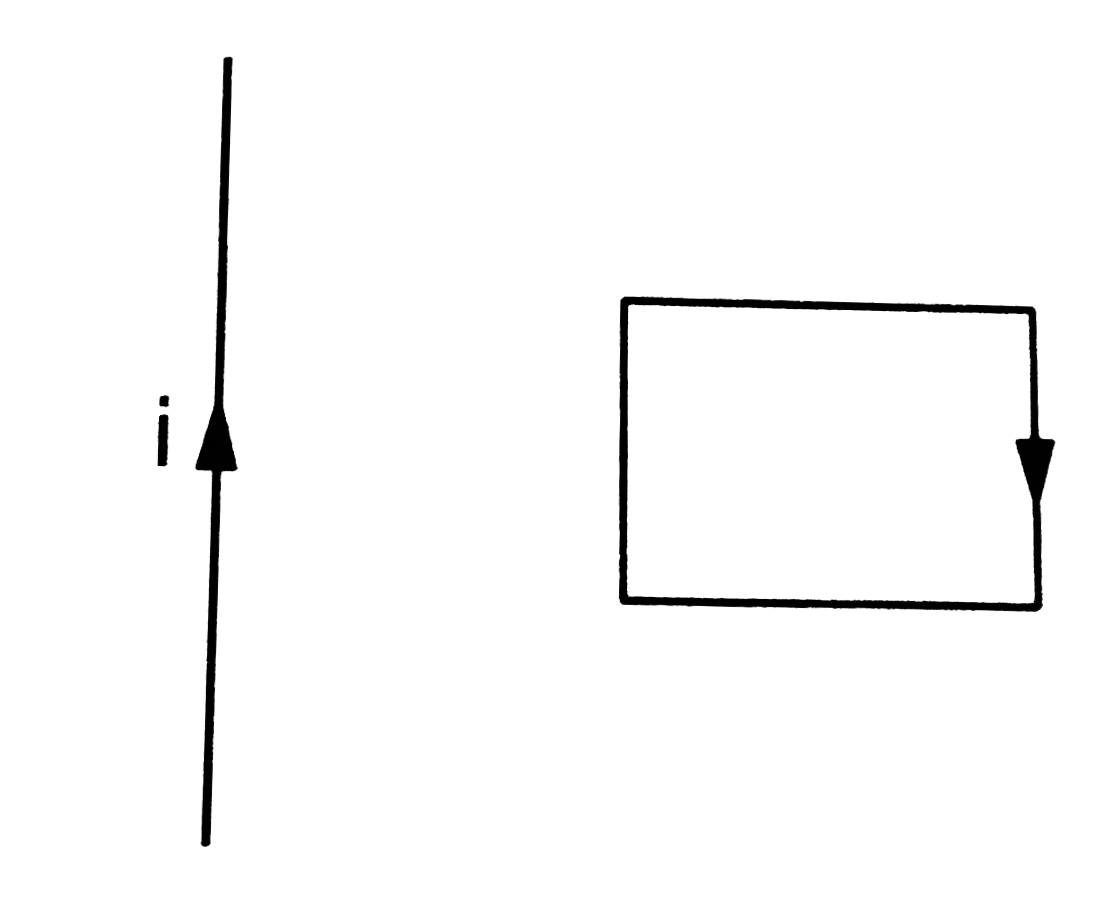
View Solutiona rectangular loop, as shown in Fig. 3.66, moves away from an infinitely long wire carrying a current I. Find the emf induced in the rectangualr loop.

View Solution
